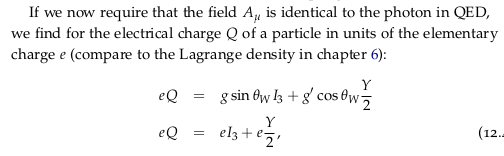In our lecture, we arrived at the following expression for the Lagrange density of the $\gamma$ and the $Z^{0}$ boson:

$$\mathcal L_{\gamma, Z^{0}} = -\sum_{e\nu}\left\{ \bar{\psi}\gamma^{\mu}\left( g\sin\theta_{W}I_3 + g'\cos\theta_{W}\frac{Y}{2}\right)A_{\mu}\psi + \bar{\psi}\gamma^{\mu} \left( g\cos\theta_{W}I_{3} - g'\sin\theta_{W}\frac{Y}{2} \right) Z_{\mu}\psi\right\}. \tag{1.1}$$

This comes from the electroweak unification.

And then we said:

The first Eq., i. e. $eQ = g\sin\theta_{W}I_{3} + g'\cos\theta_{W}\frac{Y}{2}$ is perfectly clear to me. However, where does the second Eq., i. e. $$eQ = eI_{3} + e\frac{Y}{2}\tag{1.2}$$ come from? Is this through comparison with the QED Lagrangian $$\mathcal L_{\text{QED}} = \bar{\psi}\left( i\gamma^{\mu}\partial_{\mu} - m\right)\psi - \bar{\psi}\gamma^{\mu}eQA_{\mu}\psi - \frac{1}{4}F_{\mu\nu}F^{\mu\nu}. \tag{1.3}$$

If so, then I don't see how we arrive at (1.2) by comparing $(1.1)$ and $(1.3)$.