But I'm not agree with its conclusion (I think it is wrong). Indeed, we have (I use a slightly different notation, hope it's clear): $$U(x,y,2f)=kost \ F[g(x_{1};y_{1})]_{(\frac{kx}{f};\frac{ky}{f})}$$ and after the second mask: $$U(x,y,2f)=kost \ F[g(x_{1};y_{1})]_{(\frac{kx}{f};\frac{ky}{f})} F[h(x_{1};y_{1})]_{(\frac{kx}{f};\frac{ky}{f})}$$ Finally: $$U(x,y,4f)=kost \ F(F[g(x_{1};y_{1})]F[h(x_{1};y_{1})])_{(\frac{kx}{f};\frac{ky}{f})}$$ And so I can't say that $U(x,y,4f)$ is the convolution product of $g$ and $h$ because to say it, it should be: $$U(x,y,4f)=kost \ F^{-1}(F[g(x_{1};y_{1})]F[h(x_{1};y_{1})])_{(\frac{kx}{f};\frac{ky}{f})}$$ not?
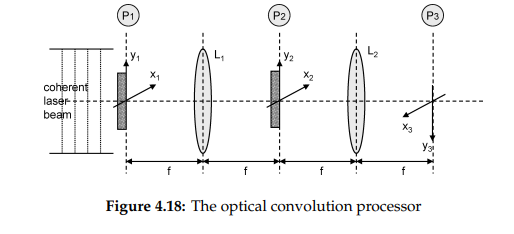
Optical convolution processor
Landau
- 768
- 10
- 21