I know that we can calculate the maximum potential of a van de graaff generator by ( radius* electric field in which corona discharge begin to form in the surrounding gas( according to wikipedia 30 kv/cm)). What if i covered the sphere with Mica with a dielectric strength ( according to wikipedia) 1.18 Mv/cm , will that increase the maximum potential of the sphere? I know it will be hard to discharge the sphere in this case , but is it possible?
$\begingroup$
$\endgroup$
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
In theory, if you had a sphere encased in a great dielectric than maybe you could charge it up to the breakdown limit of the dielectric. However, for a VdG generator, you need access to the sphere for the support and charging system, which then becomes the breakdown path.
As noted by @UncleAl, real accelerators use high pressure gas (not always SF6 since is expensive and a greenhouse gas), with the pressure and gap determined by the Paschen curves.
Another benefit of gas insulation is that it self heals after a spark, which your solid dielectric would not do, so you would need to replace the mica every time it sparked.
answered Jul 11, 2014 at 13:37
$\begingroup$
$\endgroup$
1
Van de Graaffs used as accelerators are immersed in high pressure SF6 gas,
http://accelconf.web.cern.ch/accelconf/p65/PDF/PAC1965_0266.PDF
http://www.mpi-hd.mpg.de/blaum/accelerators/mp-tandem-accelerator/index.en.html
-
$\begingroup$ well, mainly i am asking if we can replace the gas with a solid dielectric material $\endgroup$ Commented Feb 3, 2014 at 21:17
$\begingroup$
$\endgroup$
Consider the Van de Graaff generator as shown below below.
Let us get very close to the surface of the conducting sphere such that it appears like a plane charged surface. The magnitude of $\vec{E}$ in the immediate surroundings( also called the local electric field intensity ) is approximately equal to the dielectric strength of air. Any addition of an infinitesimal positive charge to the sphere would result in a breakdown. Very close to the sphere, the local electric field intensity is approximately uniform. Let the field intensity be uniform over a distance $T$ cm. Beyond this range, the magnitude of $\vec{E}$ follows an inverse distance squared relationship. In other words, $|\vec{E}|$ $\propto$ $\frac{1}{r^2}$
Look at the diagram below.
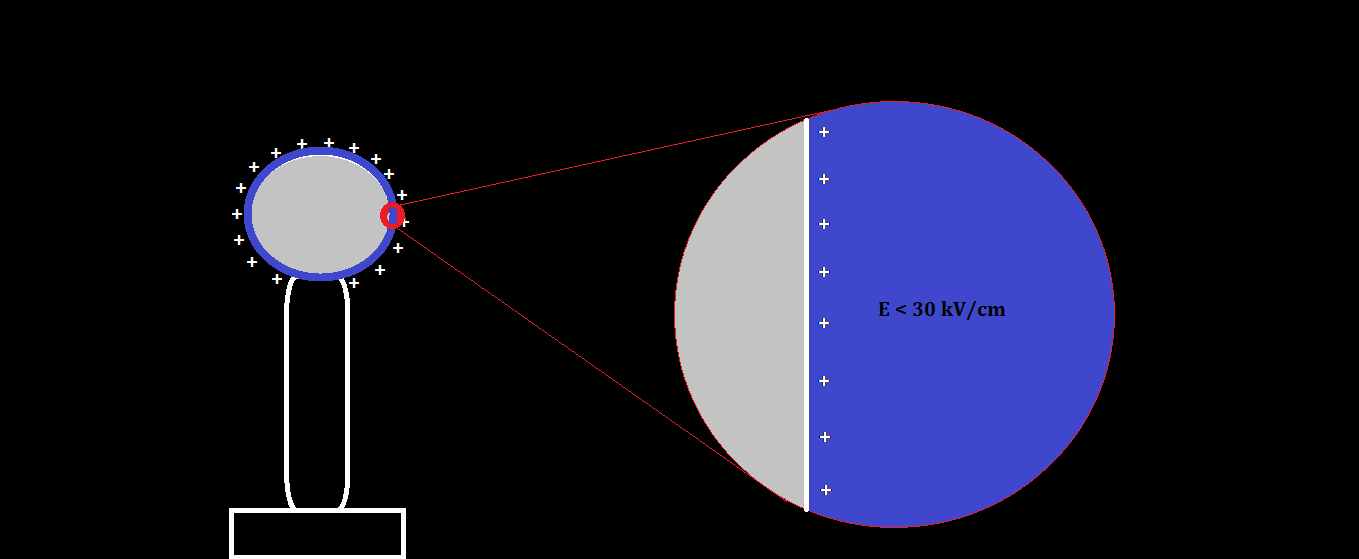
I now cover the conducting sphere with a dielectric( Mica ) of thickness $T$ cm. The electric field intensity inside the dielectric drops by a factor of $\kappa$ also known as the dielectric constant. Beyond the dielectric material, in air, $|\vec{E}|$ $\propto$ $\frac{1}{r^2}$.
I now pick a point( in air ) that is closest to the dielectric material in the diagram above. It is clear that the electric field intensity at this location is less than the local electric field intensity( scroll up for local electric field intensity ). That's because of the inverse distance squared relationship. I'm now confident that I can add an infinitesimal positive charge to the conducting sphere without leading to a breakdown. Considering the capacitance of the sphere to be a constant, from $Q$ $=$ $C$$\Delta$$V$, an increase in $Q$ on the sphere would result in an increase in $\Delta$$V$. By the way, you can simply write $V$ because we take potential at infinity to be zero. I'm used to writing it the other way. I have therefore increased the potential of the conducting sphere. I'm allowed to add extra charge till $|\vec{E}|$ at the point closest to the dielectric material is approximately equal to 30 kV/cm. Mathematically, $|\vec{E}|$ $\approx$ $30 kV/cm$. In this case, Mica has a very high dielectric strength when compared to air and you can thus expect the surroundings( air ) to first undergo a breakdown. That's the reason I mentioned a limit to addition of charge to the conducting sphere with reference to dielectric strength of air.