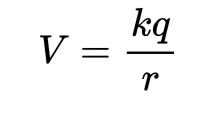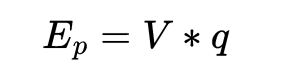4
$\begingroup$

Let's say a typical (50cm radius) Van de Graaff generator (around 60pF capacitance) is charged to around 200kV (I am following the example seen in this video).

According to the formula for an RC circuit, and assuming the human body can be approximated by a resistance of about 1000 ohms (with wet hands and at such high voltages), a spike of maximum current I = V/R = 200 amps will occur at t=0 (formula for this on this page), however this will clearly not last long at all (until about 1 microsecond where the value is essentially zero after graphing the above equation).

My first question is: are my calculations correct? Is there indeed an incredibly short but very high 200 amp spike at the beginning of the discharge?

My second question is: how is this safe? I have read things about all the energy being dissipated almost immediately as heat (maybe you will feel a shock on your finger tips only...?) but I am not sure I fully understand this. Obviously, a sustained current of 200 amps through your body is not safe at all, so what is going on here?

Note that, although my estimation of 1000ohms might be too low (although according to my research it isn't due to the breakdown of the skin) even higher resistances which still cause currents substantially above what our body can handle.

Improve this question
$\endgroup$
2
  • $\begingroup$ What is the stored energy, and how does that compare with a hazardous level of ~10J? And, don’t touch it with wet hands… $\endgroup$
    – Jon Custer
    Commented Jun 1, 2022 at 17:53
  • $\begingroup$ @JonCuster Minor point, but when you get to those voltage levels the skin already affords negligible protection even if dry. $\endgroup$
    – Bob D
    Commented Jun 1, 2022 at 18:19

2 Answers 2

Reset to default
3
$\begingroup$

Why is it safe to touch a Van de Graaff Generator?

If by "safe" you mean not causing ventricular fibrillation, then it's because the energy of the capacitive discharge is not sufficient to cause ventricular fibrillation.

The energy provided by a capacitive discharge is given by

$$E=\frac{1}{2}CV^2$$ where C is in farads and V in volts.

Plugging in your data for the Van Der Graaf generator, that energy would be 1.2 J.

To put this into perspective, I read that the ACLS (Advanced Cardiac Life Support) guidelines are a single shock of 360 Joules is indicated for causing ventricular fibrillation. That is the upper range of defibrillators intended to restore normal heart rhythm (stop fibrillation).

Although the threshold for fibrillation is lower than 360 J, perhaps on the order of 10's of Joules, the energy of the Van Der Graaf generator is still below that.

Hope this helps.

Improve this answer
$\endgroup$
7
  • 1
    $\begingroup$ I think you forgot to square the voltage. C = 60 pF and V = 200 kV give E = 1.2 J. $\endgroup$
    – Puk
    Commented Jun 1, 2022 at 19:06
  • $\begingroup$ Hmmm... but if a current of 200amps flows through your body, even for a split second, is this not "dangerous"? Or is it energy (joules) which is actually the culprit? My research seems to indicate current through the body is the culprit, but maybe this is where the underlying confusion is $\endgroup$
    – Gary Allen
    Commented Jun 1, 2022 at 19:07
  • $\begingroup$ @PukOops. Thanks $\endgroup$
    – Bob D
    Commented Jun 1, 2022 at 19:10
  • $\begingroup$ @GaryAllen The culprit is the combination of current and duration of exposure. The IEC rms current level for low likelihood of ventricular fibrillation for a 1 second exposure is about 50 ma. Given an internal body resistance of about 500 ohms, the RC time constant for the generator is about 30 ns. I believe a rule of thumb to fully charge or discharge a capacitor is about 5 time constants, or in this case, 150 ns. So after 150 ns there is essentially no voltage on the cap (and thus no current in the body). $\endgroup$
    – Bob D
    Commented Jun 1, 2022 at 19:23
  • $\begingroup$ Great this definitely makes sense then! Is there anywhere more where I can read about these IEC recommendations, how they are chosen, and how the current affects our bodies, potentially outside of the "physics realm"? @BobD $\endgroup$
    – Gary Allen
    Commented Jun 1, 2022 at 19:30
0
$\begingroup$

I guess this could be used to explain the difference between 'potential' and 'potential energy'.

Scenario 1: Van de Graff Generator:

The potential is large here, no doubt: 200kV

But:

enter image description here

If we solve for q, we get a small value.

and finally find the Energy using:

enter image description here

It comes to around 2 Joules.

Scenario 2: 110V socket: The potential is small here (110V) but the amount of charge per second could nearly be 1C (this is a very high value for charge), resulting in an Energy of 110 Joules. and so it is very dangerous to touch it.

Conclusion: The potential (energy per charge) matters less, but what is more important is the number of charges.

Scenario 1(Van de Graff) is the equivalent of throwing a few 100 tennis balls from the top of Mt.Everest.

Scenario 2(110V socket) is the equivalent of throwing a few million tennis balls from the top of empire state building.

I hope the analogy is right!

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.