Magnetic Flux in presence of variable magnetic field

Question

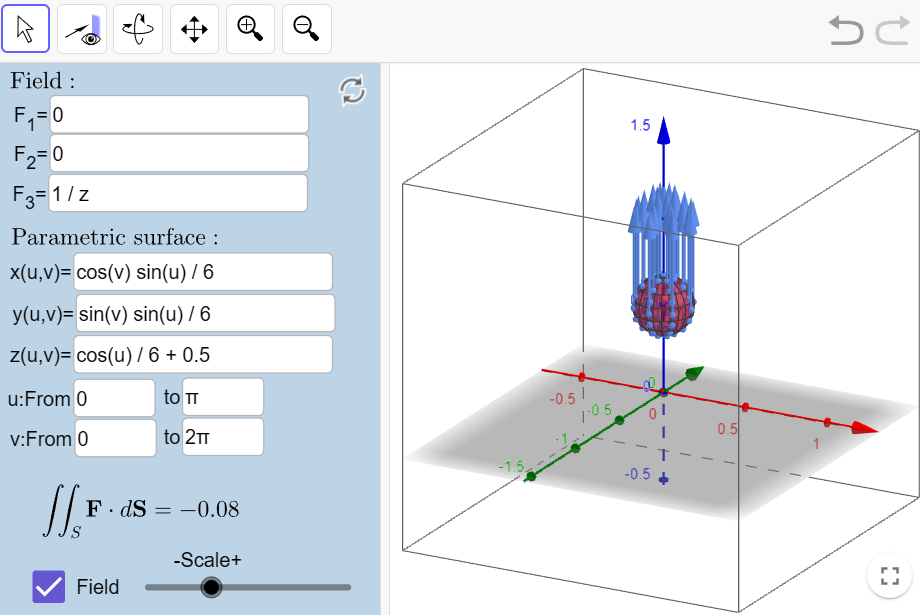
Suppose we have a changing magnetic field parallel with the $z$ axis that follows the equation

$$\vec{B} = \frac{B_0}{z} \hat{u_z}$$

If we were to choose a spherical closed surface $\Sigma$ with radius $r$ and centered at a distance $d>>r$ from the origin of the $z$ axis, Gauss's law for magnetism states that the net magnetic flux through that closed surface equals zero.

$$\Phi(\vec{B}) = \oint_{\Sigma}^{}\vec{B} \cdot dA\hat{u_z} = 0$$

However, when we calculate the flux, $z$ is smaller on the lower half on the sphere since it is closer to the origin than the other half and thus $\vec{B}$ is stronger on the closer half and weaker on the other. Wouldn't those contribution add up to a nonzero magnetic flux, even though the same number of field lines both enter and exit the spherical surface? Is this a violation of Gauss' theorem for magnetic fields?

Image for reference:

Answer 1

You are correct that your magnetic field does not have zero flux through some closed surfaces. In terms of the differential forms of Maxwell's equations, this can be seen as a failure of $\vec{B}$ to satisfy Gauss's law for magnetic fields: $$ \vec{\nabla} \cdot \vec{B} = - \frac{B_0}{z^2} \neq 0. $$ So this is not a valid field configuration for $\vec{B}$; there is no way that a magnetic field can have this configuration in space.

This shouldn't be at all surprising, but I think the conceptual issue that's giving you problem is this:

even though the same number of field lines both enter and exit the spherical surface?

If you want to think of this in terms of field lines, note that the density of field lines corresponds to the magnitude of the magnetic field. In particular, this means that the density of field lines must be greater on the lower half of the sphere. And that in turn means that field lines must be entering the sphere and terminating inside it. Magnetic field line can't do this, of course, which is another way of seeing why this is an invalid magnetic field. But if you called this an electric field instead, it would be an indication that there would be some (negative) charge inside the sphere.

(IMO, the contortions that one has to go through to think about this in terms of field lines are an indication that field lines are not the best way to think about electric and magnetic fields. They're a useful visualization tool, but what you should really try to do is develop intuition about how fields behave, not how field lines behave.)