Magnetic flux on the whole $x$-$y$ plane due to a circular loop

Question

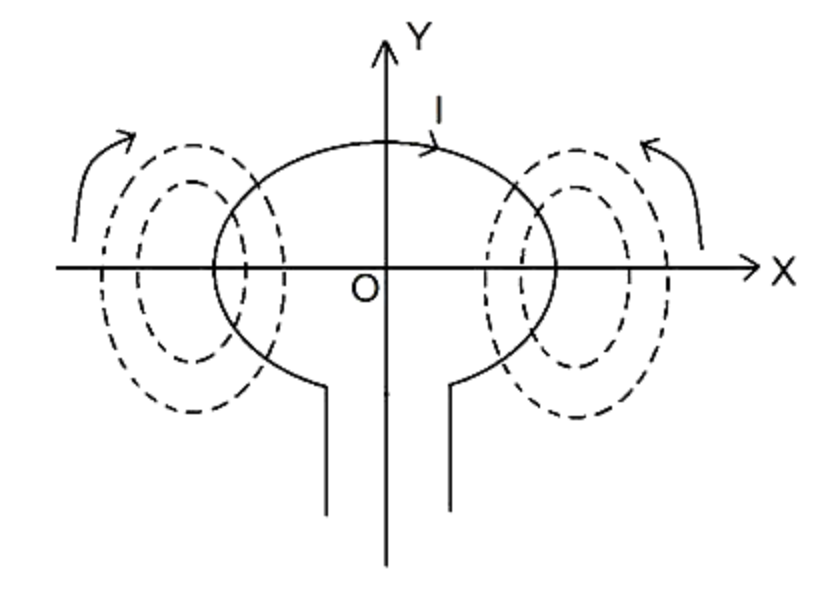
A circular loop of radius R carrying current I lies in x-y plane with its centre at origin. The total magnetic flux through $x$-$y$ plane is:

JEE Mains (1999,2M)

The question looks innocent, but then we realize that if the loop is planar, then gauss law of magnetism is out of reach. The one thing I know is that $\nabla \cdot \vec{B}=0$ and the total flux is given by:

$$ \int_{R^2} \vec{B} \cdot dA $$

But, it is tough to find an expression for field due to the at each point in plane to calculate the net flux. An explanation I found on Sarthaks explains this using the fact that magnetic field forms closed loops:

But I don't think this implies net flux is zero, because even though the field forms closed loops, the field at one end needn't contribute opposite flux to towards at the other place where the loop pierces the x-y plane i.e: the magnetic field decays as you move outwards.

Answer 1

To apply "Gauss's theorem" for the magnetic field, you can consider the whole plane and complete it with a half sphere whose radius goes to infinity. As the dipolar field decreases as $1/r^3$ and the surface increases as $r^2$, the flux through the hemisphere tends towards zero.

Since the total flux is zero, the flux through the whole plane is zero.

It is interesting to note that the flux through the circular loop alone $0<r<R$ diverges logarithmically: when approaching the wire, the field diverges as 1 / (distance to the wire) and therefore the magnetic flux diverges logarithmically when the distance to the wire tends to zero. The integral is thus zero only if it is considered as a principal value.

Answer 2

Heuristically, recall that you can loosely think of flux as the (signed) number of field lines penetrating a surface and field strength as the density of field lines in a particular region. It's fairly intuitive that if the field lines form closed loops, then the number of field lines penetrating the interior of the loop is equal to the number of field lines penetrating the exterior (with the opposite sign).

The fact that the field gets weaker further away from the loop is reflected by the fact that the field lines get spread further apart, but this is compensated for by the fact that the amount of area you're integrating over is much larger.

---

Alternatively, note that you can invoke Gauss's law by considering a box whose bottom face lies on the $(x,y)$-plane. In the limit as the box gets infinitely large, the field strength at the other 5 faces goes to zero faster than $1/r^2$, so the flux through those surfaces goes to zero and the total flux through the box is just equal to the flux through the $(x,y)$-plane. Note that to be really rigorous, this argument requires a slightly careful treatment and understanding of limits.