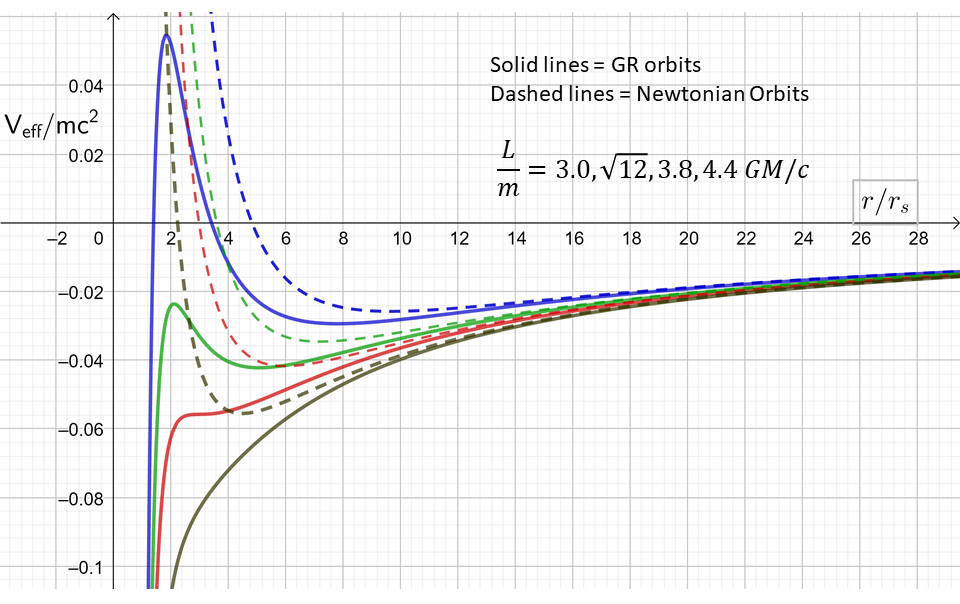
In classical mechanics, it is possible to have points of stable, unstable, and neutral equilibrium depending on the gradient of the potential field. Near a black hole, the gravitational potential becomes highly nonlinear and relativistic effects dominate.
Is it theoretically possible to find a location in the spacetime around a black hole where a test particle could be in an unstable equilibrium, balancing gravitational forces and other factors like radiation pressure or frame-dragging effects?
Would such a position depend solely on classical mechanics, or would general relativity fundamentally rule it out?
How could the dynamics of small perturbations evolve in such a scenario—would they exhibit chaotic or predictable behavior?