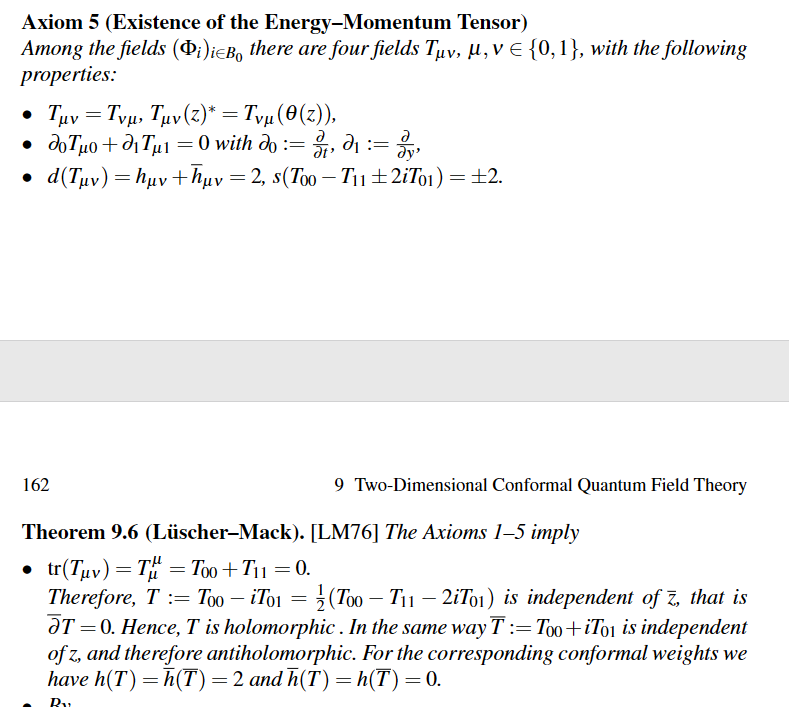
I'm studying axiomatic 2 dimensional Conformal Field Theory using my textbook by Schottenloher. (You can read here for free at RG.) I got stuck at an axiom and a theorem.
The axiom and theorem are followings.
The $B_0$ is countable set and each $\Phi_i$ is field operator. The variable $z=t+iy\ (t,y\in\mathbb{R})$. $\theta (z) \equiv -\overline{z}$.
I'd like to know what $ d$ and $s$ are in the axiom(there is not definition of d and s in the textbook) and how to prove $T$ is independent of $\overline{z}$.
\begin{align} 2\overline{\partial}(T_{00}-T_{11}-2iT_{01}) &= \partial_0 T_{00}-\partial_0 T_{11}-2i\partial_0 T_{01}+i\partial_1 T_{00}-i\partial_1 T_{11}+2\partial_1 T_{01}\\ &= (\partial_0 T_{00} + \partial_1 T_{01})-i(i\partial_0 T_{01}+\partial_1 T_{11})+i(\partial_1 T_{00}-\partial_0 T_{01})-(\partial_0 T_{11}-\partial_1 T_{01})\\ &= i(\partial_1 T_{00}-\partial_0 T_{01})-(\partial_0 T_{11}-\partial_1 T_{01}) \end{align}
In third equality, I used the 2nd equation of the axiom.
Could you give me advice or help?