Finding the accelerations and tension of a pully wedge system [closed]

Question

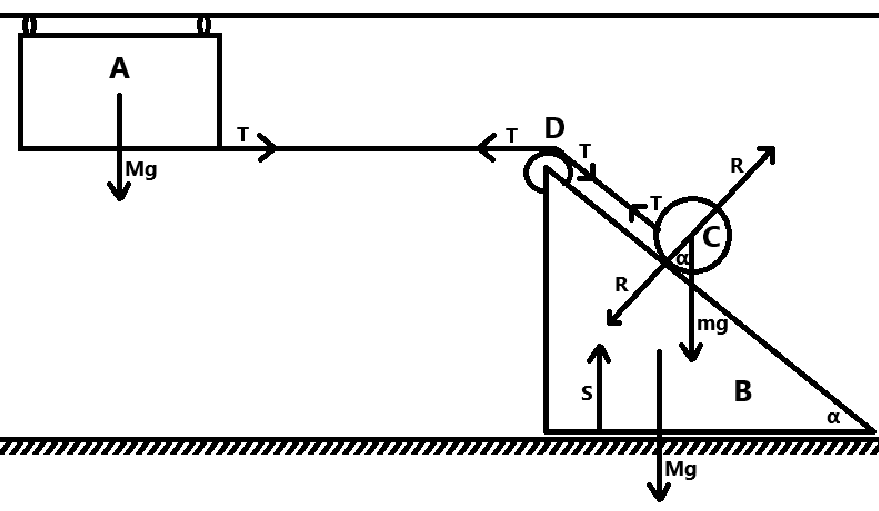
According to the figure below, a wooden cuboid A of mass "M" is suspended to a horizontal fixed straight inextensible string which passes through 2 smooth rings which are attached to the wooden cuboid such the cuboid can move freely along the string. a wedge B of mass "M" is kept on a smooth horizontal plane. a ball C of mass "m" is kept on the slope face is attached to an inextensible string which passes on a pully at D and the other end is attached to the wooden cuboid, here the portion of the string AD is horizontal. also there is no friction anywhere in this system (the ball slides)

i am to show that the acceleration of the wedge B is $$\frac{(M+m\cos\alpha)mg\sin\alpha}{(M+m)(2M+m)-(M+m\cos\alpha)^2}$$ and that the tension of the string is $$\frac{(M+m(1-\cos\alpha))Mmg\sin\alpha}{(M+m)(2M+m)-(M+m\cos\alpha)^2}$$ after the system is released from the rest

my idea on approaching this question is to label the accelerations as below

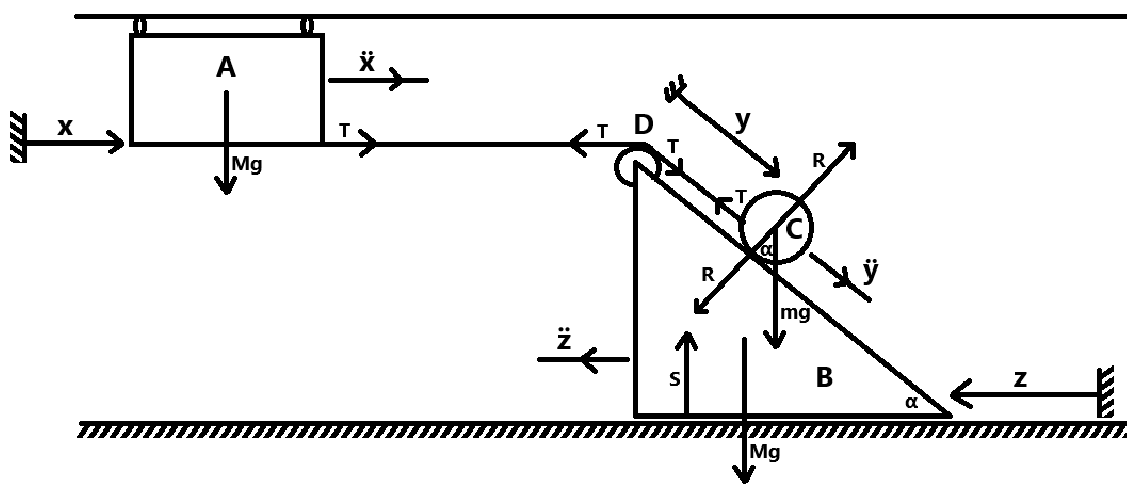
such the relative velocities are (E is for earth) $$a(A,E) = \ddot xi$$ $$a(B,E) = -\ddot zi$$ $$a(C,E) = (-\ddot z + \ddot y\cos\alpha )i - \ddot y \sin\alpha j$$

but the values for the accelerations and tensions dosnt even come close to the complexity of the final answer

can anyone explain or give mean a starting point for solving this problem? did i denote the accelerations wrongly?

Answer 1

It seems tedious but not very complicated.

You don't show what is your answer or how you arrive to it. So if you are making a mistake for example in the the forces you put in the 2nd law equation, we cannot see it.

Process:

• Make a free-body diagram
• Make an an inventory of forces acting in each body identifying the agent of each force and hence the reaction (you seem to have that right).
• Then resolve the 2nd law equations (you only showed the accelerations, now make $F=ma$).

(Hint: Forget about the pulley, consider it a frictionless feature fixed to the wedge, so the tensions act on the wedge.)

You are expressing $a(C, E)=a(C, B)+a(B, E)$. That's not wrong but also not needed. If I were to try to resolve the problem I would just do these 4 equations:

$\Sigma F_{A_x}=M\cdot a_A$

$\Sigma F_{B_x}=M\cdot a_B$

$\Sigma F_{C_x}=m\cdot a_{C_x}$

$\Sigma F_{C_y}=m\cdot a_{C_y}$

where $x$ and $y$ are just the horizontal and vertical directions relative to Earth for all 3 bodies.

Don't forget both tensions in the pulley as forces acting on $B$ (that's a mistake many of the students would make)

Note that The acceleration of $A$ is not equal to the one of $C$, regardless the string being inextensible. As a counter example, imagine leaving $A$ fixed and just sliding $B$ by hand (accelerated). $A$ will not be accelerated but $C$ will. We need to find a better relationship between the 3 accelerations that takes into account the restriction that the string is inextensible.

Finally, note that, given the previous paragraph, your last equation is wrong unless you are taking your distance $y$ (and its second derivative) relative to the the wedge, not to Earth. But that will not be a problem if you take all the accelerations in the vertical and horizontal axis relative to Earth as I proposed.