Is there a principle that determines the tension in this system?

Question

I encountered a doubt while solving this question:

My try. (I have not shown friction force direction but its leftward for all three blocks.)

I have solved this question and have got all correct answer but I did this by intution, I considered that the friction force on block of mass 2 kg and mass 4 kg will be the limiting value of it. There can be different possible cases where the friction wont be maximum for these 2 blocks like this

I think the friction is trying to minimize the tension in the ropes. Is there a principle which is applied here?

Answer 1

Apologies for posting a second answer. My first answer addressed what I considered to be the more realistic case of non-infinitely rigid connections. This answer specifically addresses the hypothetical case of infinitely rigid connections that appears to be what the text book had in mind.

Reviewing other related questions on PSE, there are two main schools of thought or interpretations and they could both be applied to the text book question in the OP and arrive at the same answer. However, I have come up with a scenario where the two approaches come up with very different results.

There are four blocks, $M_1 = 2 \, \mathrm{Kg}, M_2 = 4 \, \mathrm{Kg}, M_3 = \, \mathrm{Kg} \text{ and } M_4 = 16 \, \mathrm{Kg}$ from right to left. A force of $7 \, \mathrm{N}$ pulls on the rightmost block. The acceleration of gravity is rounded to $g = 10 \, \mathrm{m/s^2}$ and the maximum coefficient of static friction is defined to be $0.1$ so that the limiting static friction of each block is conveniently equal in magnitude to the mass of the block.

In the diagram above, the left hand side represents the first school of thought, where the friction of the blocks closest to the originating force has to be equal to the maximum static friction in order to transmit a force to a successive block further down the chain. In this example there would be no force acting on the final block.

The right hand side of the diagram represent the second main school of thought, which basically considers the four blocks to effectively act as a single block due to the rigid connections. The approach in this case is to distribute the static friction forces in the same proportions as the masses of the component blocks. In this case the final component block provides $16/28$ of the total static friction because it has $16/28$ of the total mass.

Below is an exaggerated diagram of the four blocks combined into one rigid block:

Here the combined block is resting on a deformable surface like soft wood or a carpet with underlay. The heavy end digs further into the surface and lifts the front end. The centre of the block effectively acts as a fulcrum reducing the downward force on the front block. This provides an explanation for how force can be transmitted to the rear block without having to exceed the naïve theoretical maximum static friction of the leading component block. I believe the second interpretation is the correct one. Both interpretations will provide the expected answer to this text book question, but the second interpretation will provide the correct answer in cases where the sum of the individual maximum static friction forces doesn't happen to be exactly equal to the applied force.

Answer 2

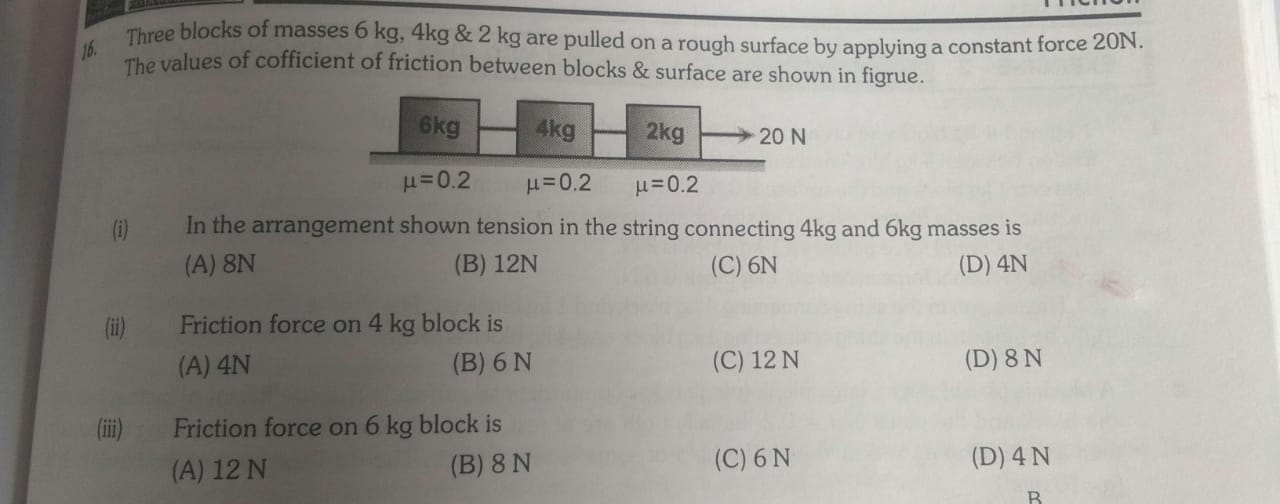
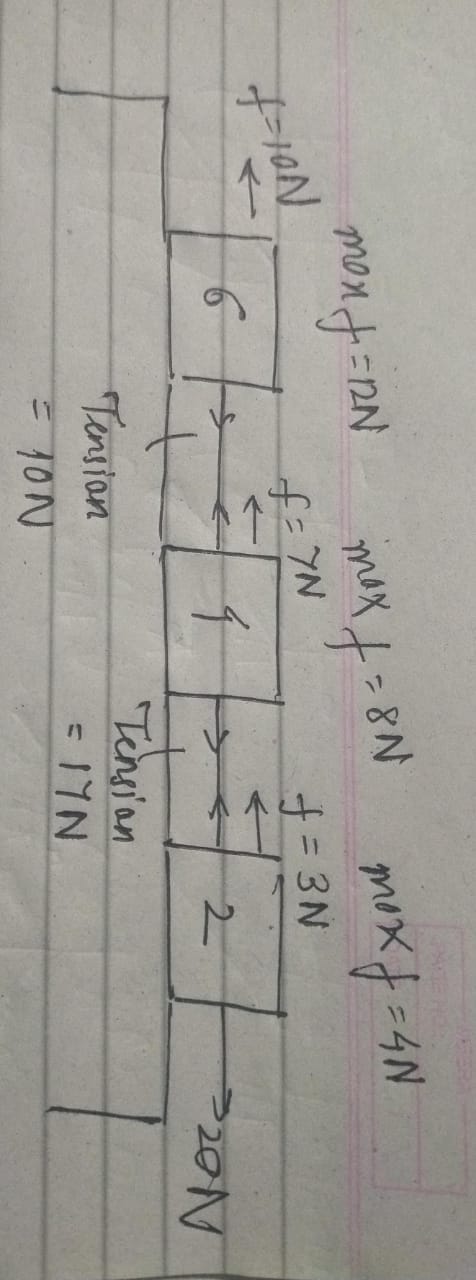
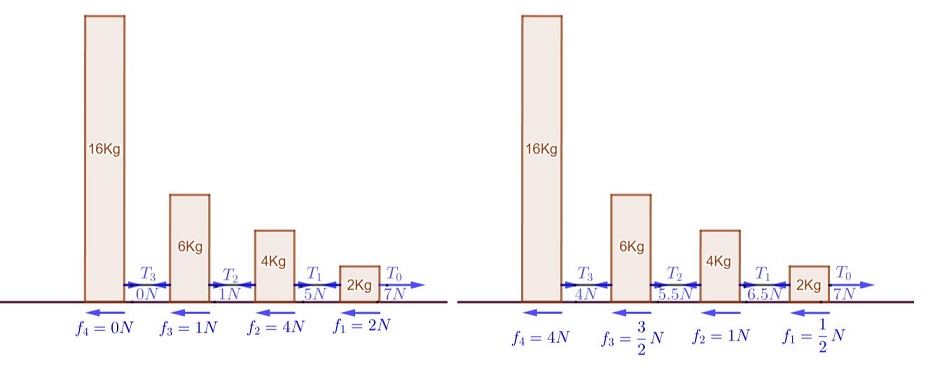
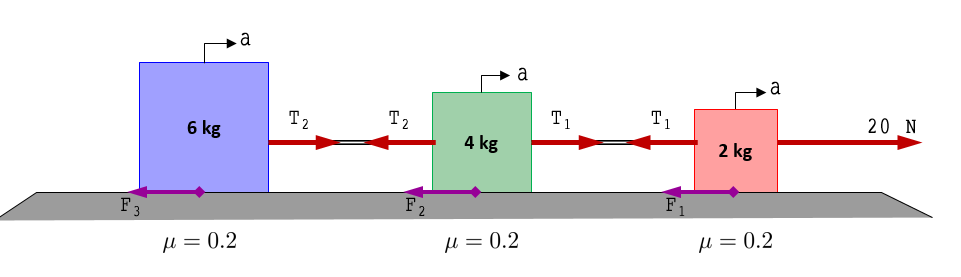
For convenience I will label the blocks as $m_1, m_2, m_3$ from right to left to be consistent with the labeling of the tensions in you diagrams.

For simplicity consider a single block of mass $m$ sitting on a rough surface with a static coefficient of $\mu_s$, with a rope pulling the block to the left with a force of $F_R$ and another rope and an initially slack rope tied to something that is effectively static like a tree. For the block to start moving to the right we require $F_R > \ m \ g \ \mu_d $. When the block starts moving the static friction changes to dynamic friction $\mu_d$. Dynamic friction is usually lower than static friction and the force acting on the rope on the left is now $F_R - \ m \ g \ \mu_d $. As the block moves the rope tied to the tree straightens out and then the tension in the rope starts increasing until it is equal to the total force acting to the right ( $F_R - \ m \ g \ \mu_d $) and the block comes to rest when the net forces on the block are equalised. Now we have $F_R$ acting to the right on the block and $F_L + m g\ \mu_d $ acting to the left and the difference between the two forces is the dynamic friction $\mu_d$. Since the block has come to rest the static friction replaces the dynamic friction and provides the required difference. We can now see when a block with forces either side moves and comes to rest again the static friction is equal to the dynamic friction when the block was moving. This concept is explained in more detail in the answer I gave to the second question linked by Vincent in his answer. This principle applies to all the blocks in the chain that were moving at any point.

Dynamic friction is usually less than static friction and for the sake of argument, let's assume the dynamic friction is half the static friction so $\mu_d = \mu_s/2$.

---

Now let's consider the whole system. When the rightmost block starts moving and the rope to the left straightens out, the forces to the right acting on the middle block are $F_2 = F_R - m_1 g\ \mu_d$. Now by a similar process if there is sufficient force, the middle block starts moving and the total force acting to the right on the last block becomes

$$F_3 = F_R - \ m_1 \ g\ \mu_d - \ m_2 \ g\ \mu_d $$

$$\rightarrow F_3 = F_R - \ m_1 \ g\ \mu_s/2 - \ m_2 \ g\ \mu_s/2. $$

Putting in the numerical values of the question I end up with:

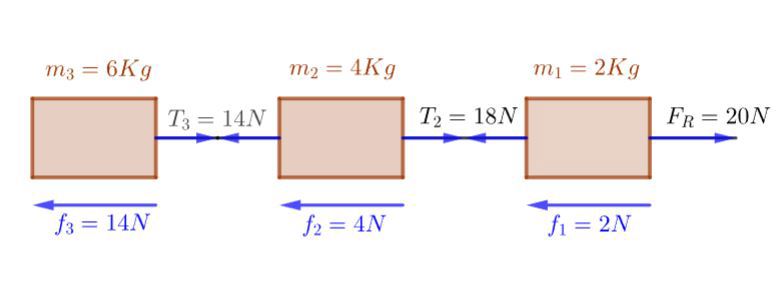
If the last block is heavy enough everything comes to a stop and the static friction of the leftmost block rises to match all the forces to the right. However, you might notice a problem in the diagram above. The last block is too light to provide sufficient static friction and in this case the whole system moves to the right. The reason my answer differs from the text book result, is that I have assumed extensible strings that allow the blocks to move one at time. The textbook seems to have assumed the block are absolutely rigidly connected by rods, (even though it mentions strings in the question and in the real world nothing is absolutely rigid) and that everything effectively behaves as a single rigid block.

In general for non-rigid connections, the difference in tension between the ropes on either side of a block is equal to the dynamic friction. For rigid connections, the difference in tension between the ropes on either side of a block is assumed to be equal to the maximum limiting static friction.

If you want to use infinitely rigid connecting rods and try to apply Young's modulus or Hooke's law to determine the tension in the rods you end up with indeterminate results and infinite rigidity doesn't really make much sense. However, we could imagine that if the blocks bend when a net force is applied to them, they could in principle transfer a force to the next block via a rigid rod without the base moving and in that case the assumed method used in the text book works using those assumptions which were not clearly stated in the text book question.

Answer 3

You are essentially asking about the distribution of static friction when an extended body at rest on a rough surface is pulled from one end. The answer is that it is indeterminate without more information about the stress and strain.

If the strings are completely inextensible, then the system behaves as a single rigid body when pulled. In this case, the strings may be replaced with perfectly rigid rods with perfectly rigid attachments without affecting the behavior of system. The friction acts on the entire system as a whole and there is no unique way to distribute it among the blocks.

However, realistically, if you allow the strings to be extensible, then your intuition is correct; the friction is applied from first to last such that each block reduces the tension by the maximum amount allowed by its friction until the tension reaches zero. In other words, only when the static friction threshold of a given block is exceeded does it pass on the remaining tension to the subsequent block.

See this post and this similar question for more information.

Answer 4

The problem is poorly worded. "Pulled on a rough surface" could be interpreted to mean the blocks are in motion, in which case the coefficients would be for kinetic friction. But that would result in a total kinetic friction force exceeding the 20 N applied force, which is impossible. So we must assume that the blocks are not in motion and that the coefficients are for static friction, even though the problem statement doesn't identify which one it is.

That said, I believe your first solution is correct. However, I wouldn't word the underlying principle as "...the friction is trying to minimize the tension in the ropes", although that is what occurs. Rather, I would word it along the lines of condition (3) discussed below.

First of all, for all the blocks to not be in motion at least three conditions must be met: (1) The sum of the force on each block must equal zero and (2) The limiting static friction force for each least one block cannot be reached and (3) In order for a block in front to transmit tension to a block in back of it, the friction force on the block in front should equal the limiting static friction force so that motion is impending, or imminent, but can't actually occur as long as condition (2) is satisfied.

So we need to start with the first block, i.e., the 2kg block. Applying (3) above, in order for it to transmit tension to the 4 kg block its friction force must equal its limiting static friction force of 4N (using g=10 m/s$^{2}$). Then applying (1) the tension in the string between the 2kg and 4kg block equals 20N-4N=16N.

Repeating the procedure for the 4kg block, in order for tension to be transmitted to the 6kg block per condition (3) above, the friction force on the 4kg block must equal its limiting friction of 8N. Applying condition (1) produces a tension between the 4kg and 16kg block of 8N.

Finally, for the net force on 6kg block to be zero its friction force must equal 8N. Since this is less than the limiting friction force of 12N, there is no impending motion of the 6kg block, satisfying the overall requirement of (3) above.

Hope this helps.

Answer 5

The way to approach friction problems is to consider all the sliding contacts as sticking first and finding the friction force required to enforce the sticking.

1. Assume all bodies have zero acceleration and find the forces needed to do so.

2. If any of the forces is more than the available traction then limit the friction force and allow the accelerations to be non-zero.

3. Find the forces and accelerations for the adjusted system and repeat step #2 if needed.

With the example in the question we have the general free body diagram and corresponding equations of motion as follows

0. FBD

   EQUS $$\begin{aligned} 20 - T_1 - F_1 & = (2) a \\ T_1 - T_2 - F_2 & = (4) a \\ T_2 - F_3 & = (6) a \end{aligned} $$ where each tension $T_i$ acts in equal and opposite measure on each connecting pair, and friction $F_i$ acts in negative (to the left) direction.

   The limits for friction are $$ \begin{aligned} F_1 < 0.2 (2) 10 & = 4 \\ F_2 < 0.2 (4) 10 & = 8 \\ F_3 < 0.2 (6) 10 &= 12 \end{aligned}$$

1. First try $F_1>0$ and $F_2 = F_3 = 0$ while not moving $a=0$. This is $$\left. \begin{aligned} 20 - T_1 - F_1 & = 0 \\ T_1 - T_2 &= 0 \\ T_2 &= 0 \end{aligned} \right\} \begin{aligned} F_1 &= 20 \\ F_2 & = 0 \\ F_3 &= 0 \\ T_1 &= 0 \\ T_2 &= 0 \end{aligned} $$

   since $F_1$ violates the available traction of $4$ we move on to

2. Next try $F_1=4$, $F_2 > 0$, and $F_3 = 0$ while not moving $a=0$. This is $$\left. \begin{aligned} 20 - T_1 - 4 & = 0 \\ T_1 - T_2 - F_2 &= 0 \\ T_2 &= 0 \end{aligned} \right\} \begin{aligned} F_1 &= 4 \\ F_2 &= 16 \\ F_3 &= 0 \\ T_1 &= 16 \\ T_2 &= 0 \end{aligned} $$

   but here $F_2$ violates the available traction of $8$ and we move on to

3. Next try $F_1=4$, $F_2 = 8$, and $F_3 > 0$ while not moving $a=0$. This is $$\left. \begin{aligned} 20 - T_1 - 4 & = 0 \\ T_1 - T_2 - 8 &= 0 \\ T_2-F_3 &= 0 \end{aligned} \right\} \begin{aligned} F_1 &= 4 \\ F_2 &= 8 \\ F_3 &= 8 \\ T_1 &= 16 \\ T_2 &= 8 \end{aligned} $$

   Here we note that indeed friction is valid for the 3rd body $F_3 < 12$ so we stop and do not consider the case where $a>0$

So the full solution is

$$ \boxed{ \begin{aligned} a & = 0\;{\rm m/s} \\ F_1 &= 4\;{\rm N} \\ F_2 &= 8\;{\rm N} \\ F_3 &= 8\;{\rm N} \\ T_1 &= 16\;{\rm N} \\ T_2 &= 8\;{\rm N} \end{aligned} }$$