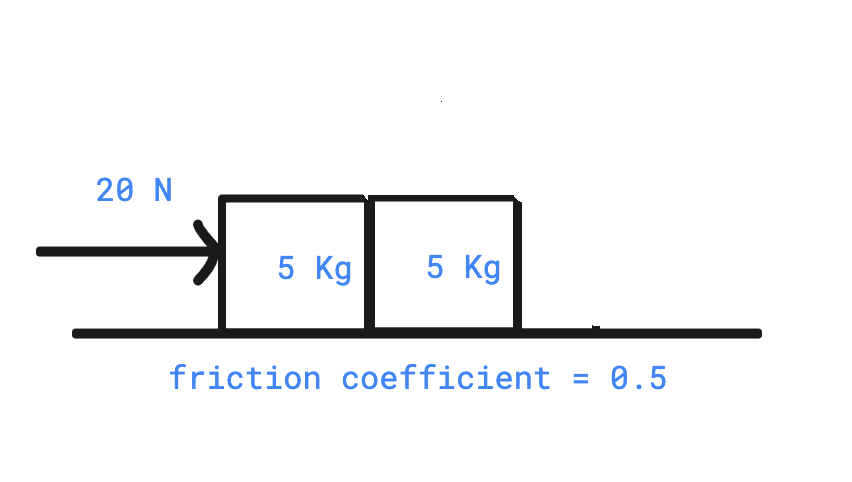
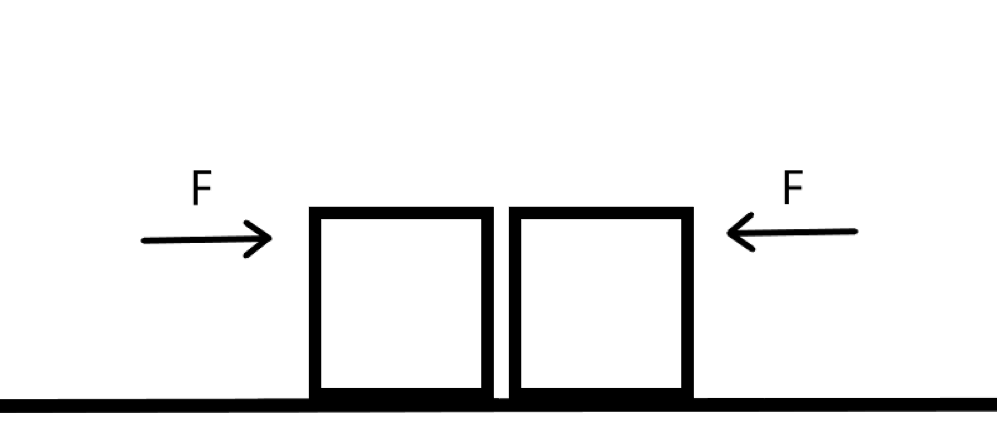
The answer is actually a little more complex than it would seem. It can be either, depending on how exactly they are touching and the atomic structure of the blocks. In order to understand the answer, we have to consider a few things:
First, why does friction happen? Friction is essentially like collisions between the uneven protruding edges between two rough surfaces, like the image below:
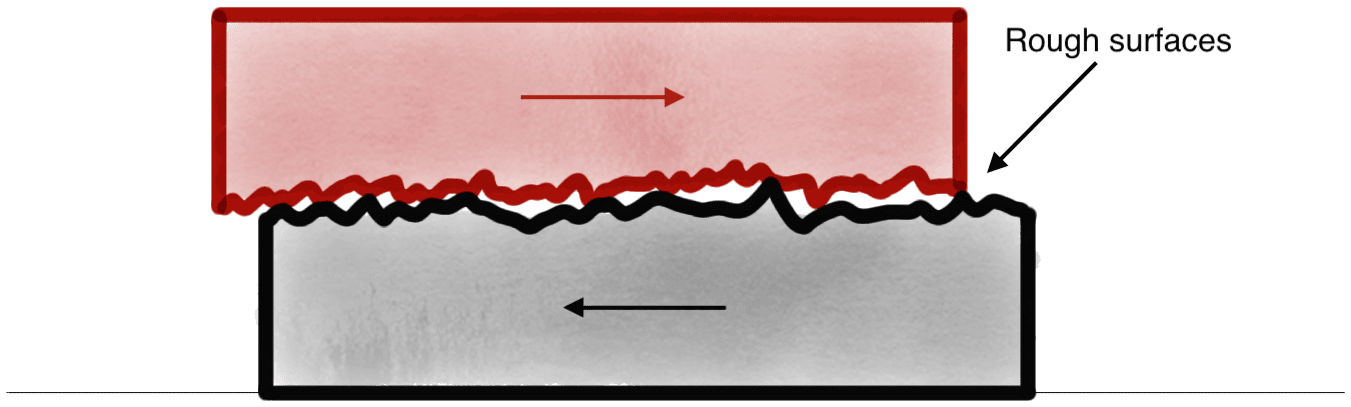
So if there were no intersecting edges at the smaller scales, there would be no friction.
Secondly, considering an example where there is only one block, how is the friction getting distributed in the whole block? It is only the bottom surface where the friction is actually happening, but the whole block seems to be experiencing it. Also the initial force is only being applied in one area, then how are the other areas also experiencing the force? This is because the block is a rigid body. In order to understand this, we have to look at it at the molecular level, which brings us to the third point.
What do you mean by a block, or two blocks? A block can be divided into atoms(or molecules if it is a compound, but I'll just use atoms for simplicity), which are held together by forces of attraction, have small amounts of space in-between, and repel when pushed against each other. So even one block is made up of many tiny blocks i.e. atoms which have gaps between them. So what happens when you apply a force on one area of the block? The atoms only in that particular area experience the force initially, and so they should be on the verge of being displaced, but then 3 things happen:
The atoms are about to be displaced but because there is a force of repulsion when atoms are squeezed against each other, they can't pass through each other, and a minimum gap is maintained. So the force is carried over to the next atoms, and then to the next and so on, in a line along the force. This is how the force gets spread horizontally, parallel to the initial force.
The atoms that are about to be displaced pull on the other atoms surrounding it, attempting to pull them in the direction of the force. Those atoms then pull on the other atoms surrounding them, and so on until atoms at both the bottom and the top experience the initial force. This is how the force gets spread vertically and also diagonally.
Finally when initial force is carried over to the atoms at the bottom, they are about to be displaced, but they experience a frictional force, which prevents them from being displaced. Then just like the 2nd point except this time starting from the bottom, the frictional force also carries over to the surrounding atoms, and this keeps on happening, until all atoms experience the frictional force, and so none of them get displaced.
That was the detailed mechanism of how forces get distributed within one block. Now we can take the same principles and see how it applies to two separate blocks that are 'touching'. As we have already seen, at the atomic scale, the atoms of the two block will have a repulsive force between them and a minimum gap will be maintained. If two blocks are 'touching' such that the gap between the atoms of one block to another are already at a minimum then the forces will get distributed between both blocks. Otherwise not, and only the friction of the first block will apply