2
$\begingroup$

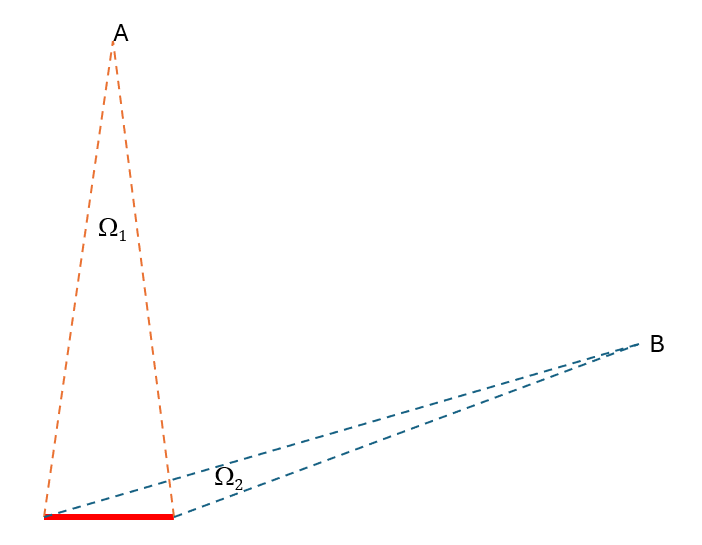
I'm really worried about "Lambert's law": A blackbody emitter is supposed to be "Lambertian" but I know from blackbody radiation that its radiance L (W/m²/Sr) is independent of direction: This means that an observer A measures the same radiance as observer B. However, since the object appears at a smaller solid angle for B than for A, the total power on a detector of same area is smaller for B. This is a purely geometric effect due to the reduced solid angle at which the emitter for B is seen compared to A.

On the other hand, I often read that the radiation density L emanating from a Lambertian surface is already angle dependent according do a cos-law and disappears at an angle of 90°. But isn't that wrong? A Lambertian radiator appears equally bright from different viewing angles and the fact that B receives less power compared to A is only due to the reduced solid angle and not because of physics. I might be wrong...

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

A Lambertiam emitter appears equally bright from all viewing angles. That is, the power per unit perceived area stays the same. Both the power received and the solid angle it arrives from decrease as $\cos\theta$, leaving their ratio unchanged.

The brightness of the surface, as perceived by the observer, is the received power per unit area, at the receiver, divided by the solid angle subtended by the source at the receiver (which is equal to the projected area of the source divided by its distance squared).

This brightness is constant because both the numerator and denominator in the ratio depend on $\cos\theta$.

I can only suspect that any confusion arises from confusion about areas and solid angles measured at the receiver vs areas and solid angles defined at the source.

Improve this answer
$\endgroup$
8
  • $\begingroup$ This is what I said above: But that means, that radiance of an Lambertian emitter is the same for all angles of observation -> it appears equally bright from all angles. But that means, that radiance L is independent of angle. However Lambert's law states, that L must be multiplied by cos $\theta$. This is what I mean. I guess that Lambert's law is not about radiance but another quantity - but which? $\endgroup$
    – MichaelW
    Commented Oct 25 at 6:21
  • $\begingroup$ Radiance being 'watts per square meter per steradian' from a flat surface, means that 'per steradian' part calls for the multiplication by cos theta to get watts per square meter. It's the object shape effect for a flat surface (wouldn't happen for a sphere). $\endgroup$
    – Whit3rd
    Commented Oct 25 at 8:27
  • $\begingroup$ yes, but this is a geometric effect because the solid angle under which my object appears is smaller. Nevertheless the radiation emitted by a radiating surface is isotropic. What I mean ist: Putting the sun for example, its radiance is the same in the whole universe - ist is the solid angle which shrinks by distance. And the measured radiance from the edge of the sun ist approximately the same as the radiance when looking at its center. Otherwise the edge of the sun would be dark, because rays come with phi ~ 90°. This is not what we observe, however. $\endgroup$
    – MichaelW
    Commented Oct 25 at 8:58
  • $\begingroup$ @MichaelW the radiation emitted by a flat surface as you illustrated, is not isotropic. The Sun is not a flat surface. $\endgroup$
    – ProfRob
    Commented Oct 25 at 9:29
  • $\begingroup$ Ok - sun hast limb darkening. It is a black body emitter only in first approximation- bad example. But lets imagine a sphere radiating like a a black body. In this case I wouldn't have darkening at the edge - right? I guess, that the edges would appear as bright as the center: see for example rp-photonics.com/lambertian_emitters_and_scatterers.html first paragraph. They say: "At a first glance, the statement of constant radiance and Lambert's cosine law appear to be contradicting each other". It is exactly this discrepancy which I want to address, but I don't understand the answer $\endgroup$
    – MichaelW
    Commented Oct 25 at 9:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.