Black body radiation and second law

Question

I'm struggling on finding what's wrong with my model of the following system which seems to break the second law:

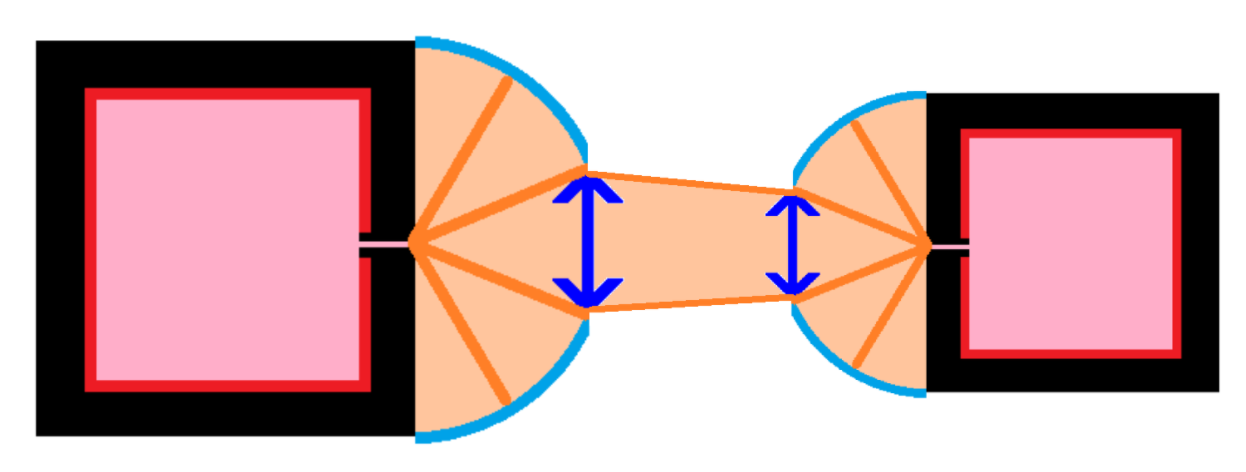
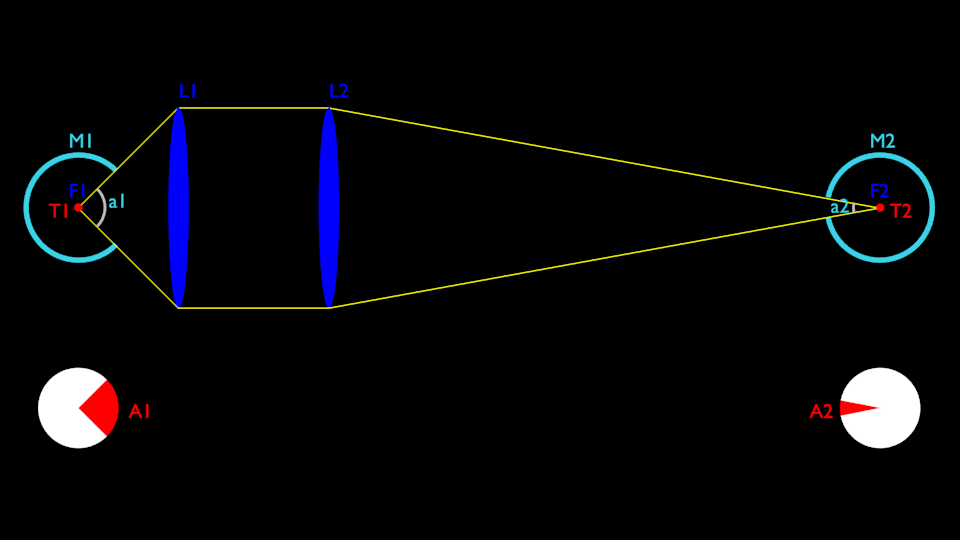
Imagine two punctual perfect black bodies at two temperature $T1 $ and $T2$. Each black body is perfectly isolated at the center of a spherical mirror which reflect all the emitted radiation on it. In each spherical mirror, there is a hole defined by the difference between the sphere and a cone of angle $a1 $ or $a2 $ whose summit is on the corresponding black body. The 2 hole face each other, and lengths duct all the rays escaping from one black body to the other one:

At the equilibrium, the power exchange between the two black bodies are the same:

$P1 = P2$

the Stefan–Boltzmann law gives us:

$ \sigma T1^4 S1 = \sigma T2^4.S2$ with S1 and S2 the surface of the spherical hole

$ T1^4.R²/2*a1 = T2^4.R²/2*a2$ with $R $ the radius of the spherical mirrors

$ T1/T2 = (a2/a1)^{1/4}$ $T1 = k.T2 $ with k in R+

This result shows that with the good set of value for $a1 $ and $a2$, the system can reach an equilibrium for any (relative) values for $T1 $ and $T2$. This is really disturbing for me. For instance I could have a configuration where at beginning T1=T2 and at the equilibrium $T1>>T2$, which seems to break the second thermodynamic law... (if this is real, I put a thermal engin between the two black bodies to generate unlimited power ^^)

What's wrong with this approach? I'm suspecting that the Stefan–Boltzmann law doesn't apply like that if the black body already receive radiations, but I have no clue about this

Answer 1

The Stefan Boltzmann law is the wrong law to use for this problem. The Stefan Boltzmann law describes the total power radiated by a black body, not the power transferred between two black bodies. The correct law is the radiative heat transfer law which can be derived from the Stefan Boltzmann law restricted appropriately for the geometry: $$\dot Q_{1\rightarrow 2}=\sigma A_1 F_{1\rightarrow 2}(T_1^4-T_2^4)$$ where the main thing you were missing in your expression is $F_{1\rightarrow 2}$ which is the view factor from object 1 to object 2.

Since $A_1 F_{1\rightarrow 2}=A_2 F_{2\rightarrow 1}$ it doesn’t matter which object you consider. You only get $\dot Q=0$ for $T_1=T_2$

Answer 2

Here is a more detailed shema, especially with the optical peering system detailed:

Lets call A the area of a black body (the 2 are identical), reciprocity rule gives us:

$A.F_{1\rightarrow 2} = A.F_{2\rightarrow 1}$

Also:

$F_{1\rightarrow 2} + F_{1\rightarrow M1} = 1$

$F_{2\rightarrow 1} + F_{2\rightarrow M2} = 1$

After substituation:

$F_{1\rightarrow M1} = F_{2\rightarrow M2}$

So, as the two black bodies have the same area, this would means the two mirrors receive the same amount of incoming power from their black body, whereas their surface exposition are largely different from each other, while the configuration is the same.

This shows there is a flaw somewhere. It highlights that their must be another power source/drain for our mirror explaining this equality.

For me the issue comes from the ponctual sources. If they have an emitting outter area, this means they are not punctual (as pointed by @Dale). This implies that the image of one black body is not perfectly the image of the other one (bigger or smaller). Thus, some rays may miss the pseudo punctual black body, reaching the mirror and probably coming back to the emitter, which explains the previous results and why the modelisation of the system is wrong (can't use punctual source here)

Thank you @Dale for your help, working with view factors helped me to figure out this