Computing how a force splits between different force-carrying structures

Question

This is similar to a related question that I asked a while ago.

I am trying to understand how forces are transmitted between point-like masses that are lodged in or adjacent to rigid bodies. I am not even sure that the question that I am asking is well defined, by the way.

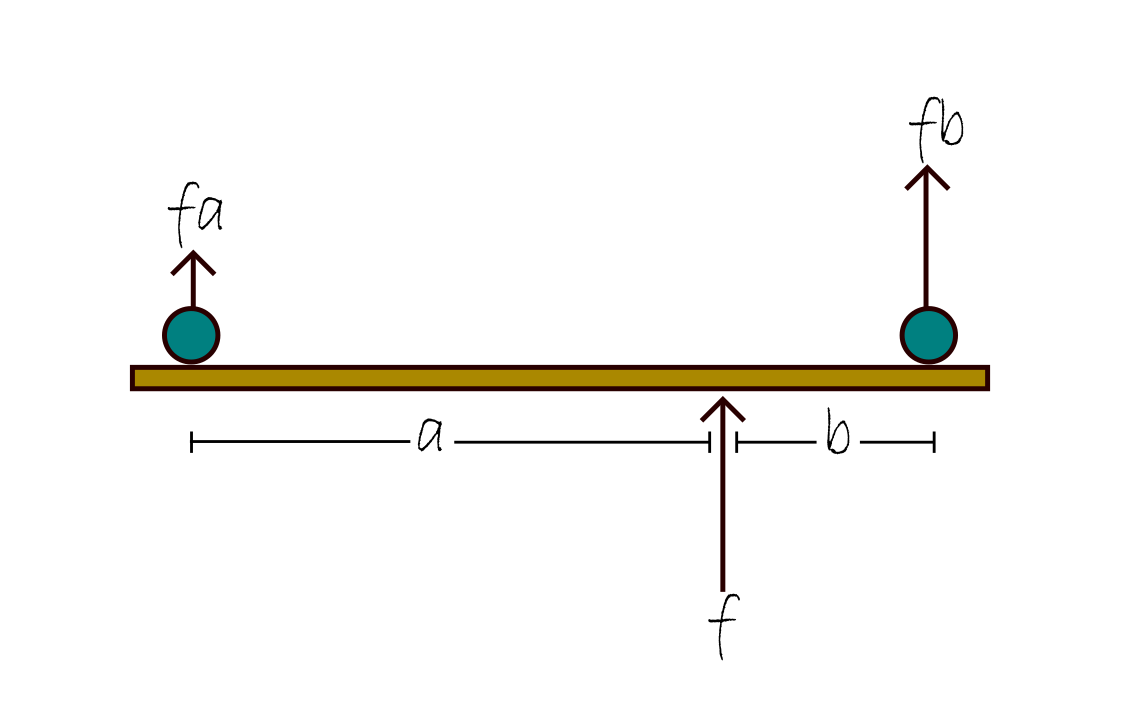
In the system below, a force $f$ is applied to a massless rod, that touches two "force sensors". The sensors are spaced $a$ and $b$ to the left and to the right of the point of application of force $f$. What vector forces will the sensors detect?

My guess is $$f_a = b \ \frac{f}{a + b} \quad \text{and} \quad f_b = a \ \frac{f}{a + b}.$$ It's motivated by the expectation that if you apply the force directly on a sensor, the other sensor sees nothing. Also, it seems to provide a sensible result when $a = b$. Finally, if I were to approach this as a static analysis, I would write down the moments, balance them, and probably find a similar result.

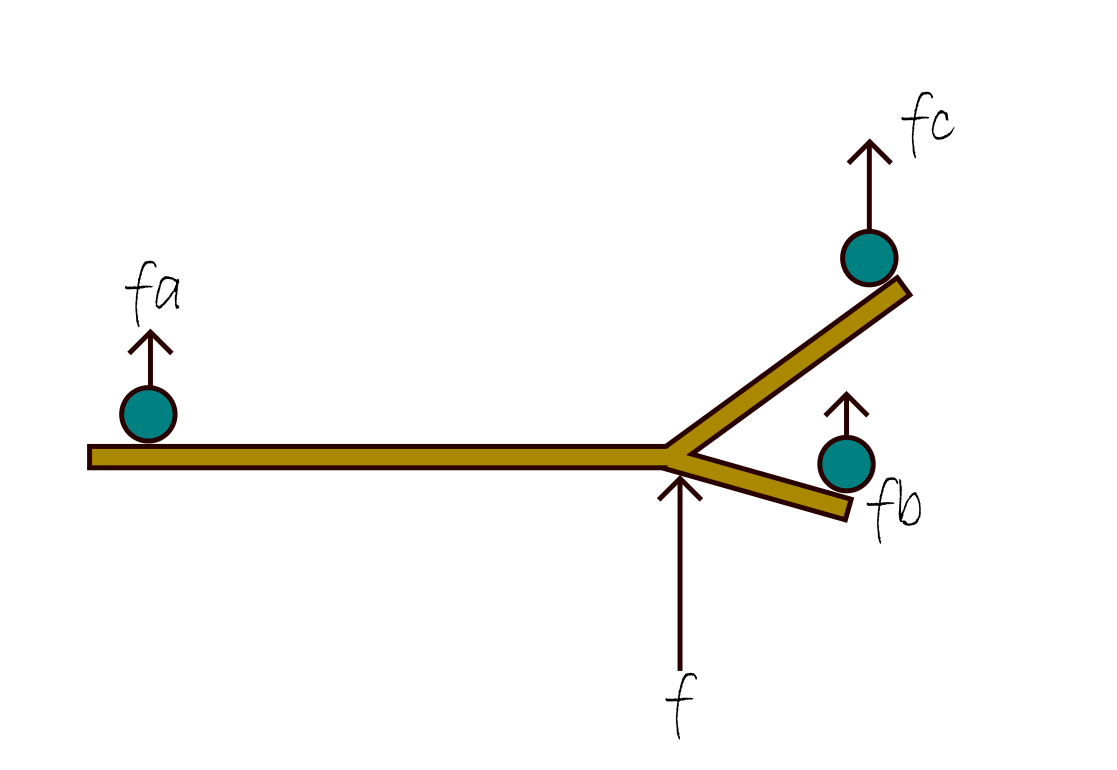
But the question is about whether there is a way to compute these forces in arbitrary situations, and without resorting explicitly to moments. For instance, using only Newton's laws and the geometry of the system, is it possible to compute how $f$ is split in $f_a, \ f_b$ and $f_c$?

Answer 1

Using only Newton's laws, we derive the constraint: $f = f_a+f_b+f_c$

This equation has one known and three unknowns, and cannot be used to compute unique values of $f_a$, $f_b$, and $f_c$. Such an equation is called indeterminate.

The only way we can get more information is by using the law of conservation of angular momentum. This law cannot be derived from only Newton's laws. The next equation would be: $f_a \cdot a = f_b \cdot b + f_c \cdot c$

Here, $a$, $b$, and $c$ are the distances between the lines of action of $f$ and $f_a$, $f_b$, and $f_c$ respectively.

Still, we have two equations for three unknowns. This is where rigid body mechanics ends, and we must resort to writing an equation that accounts for the material and cross section properties of the body.

These matters are specific to the exact structure you are studying. I'd recommend you to read more about statically indeterminate structures and the mechanics of solids.