0
$\begingroup$

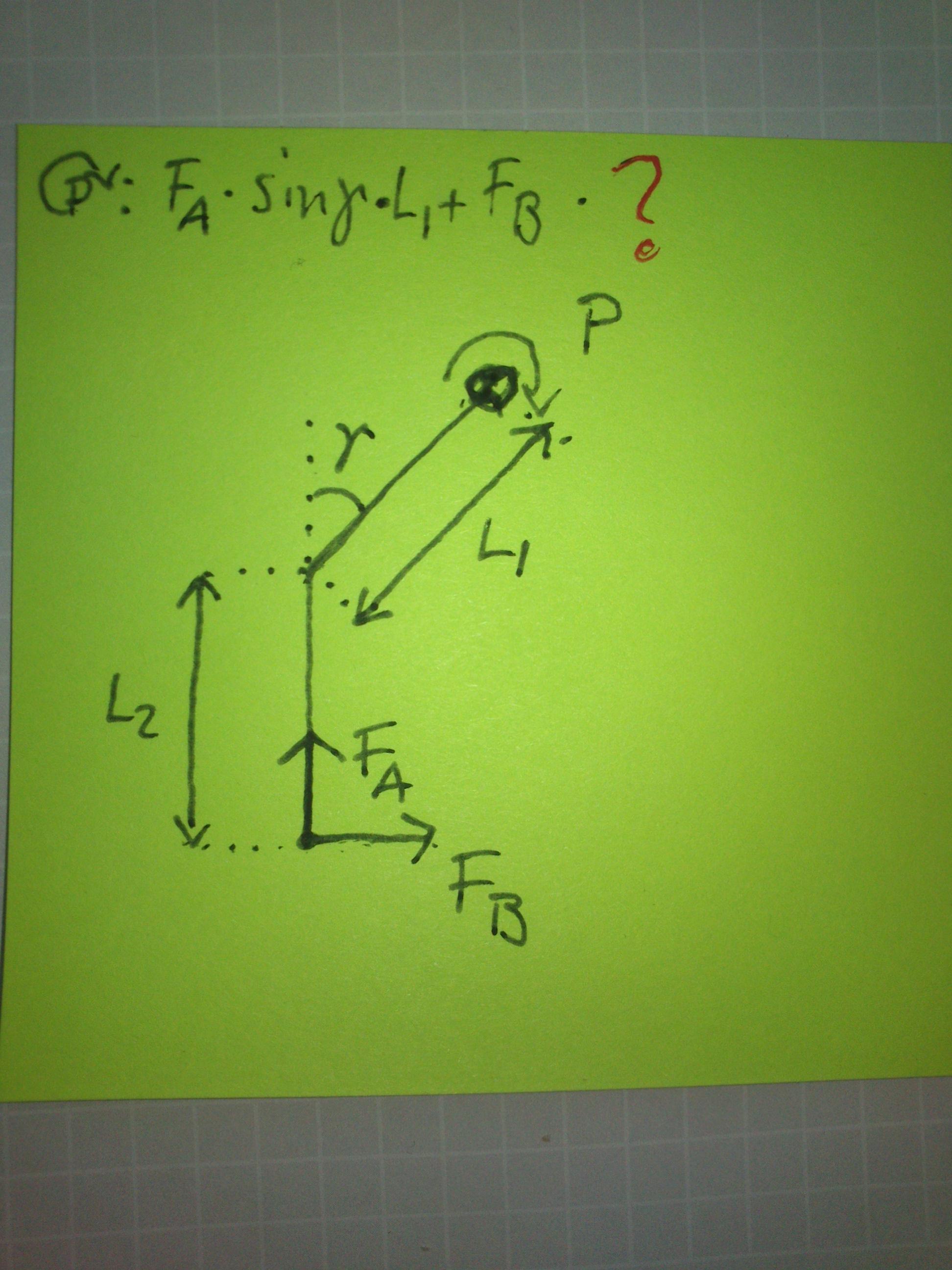
I have two rods with lengths $L_1$ and $L_2$, connected with a joint in the middle and I would like to express the moment(torque) around a point mass (P) at the far end of rod 1. The relation between the rods is expressed by the angle $\gamma$. Two forces act upon the far end of rod 2, $F_A$ and $F_B$, the latter orthogonal to the rod and the former along the rod in the heading of the joint. For the force $F_A$ the moment should be $F_A * L_1 * \sin{\gamma}$. What I'm not sure of is how the force $F_B$ should act, will it be a torque in the joint that can be translated to the point mass, i.e. $- F_B * L_2 $ or will it not affect the point P?

Thank youImage describing the problem

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
0
$\begingroup$

Draw a line from P to the tail of F(b). Now, the length of that line multiplied by the component of F(b) perpendicular to that line gives the torque due to F(b) about P. Nothing more.

Improve this answer
$\endgroup$
0
$\begingroup$

See, by definition torque is the product of force and the perpendicular distance between the line of action of the force and the axis from where the torque is being measured . By your figure it is $F_B ×(L_2+L_1cos \gamma)$

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.