0
$\begingroup$

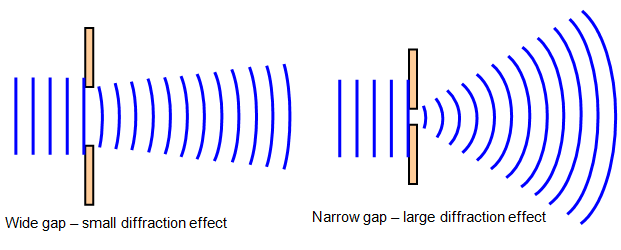
I've read quite a few posts asking a similar question but I suppose I'm still not quite satisfied with the explanations (hence the question). I'm aware that diffraction always occurs and I know the Huygens-Fresnel principle (other answers have referred to it but I don't see how it helps), but for my own intuition I want to ask in particular about the following image as this is the famous one I see everywhere and I want to try to make sense of what's going on.

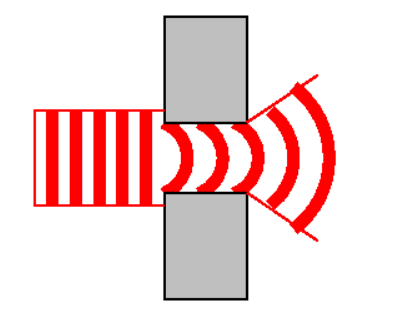
enter image description here

Knowing that each vertical line corresponds to the crest of a wave, and thus the distance between them corresponds to the wavelength, I want to (pictorially if possible) understand why the slit must be on the order of this separation for the radial wave pattern to be most apparent? It does not appear obvious to me why this matters, as in my mind I could understand if one vertical line entered the split and became radial by way of the image below, where one of the previous answers said, "If the hole is smaller than λ , the beam will "bend" in order to keep its length and fit into the hole, as is sketched on the following picture, zoomed on the hole (I have drawn the beam as a train of stems that bend when entering the hole)".

enter image description here

However, if there's a dependence on the wavelength, then there's a dependence on other vertical lines coming in to become radial and it's this that I want to understand. Maybe I have misunderstood something else which is confusing me, so if anyone could help give me an intuition of this then that would be greatly appreciated.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Your illustrations aren't quite right because diffraction isn't something that a slit does, it's something that the edges of a slit do. Each edge is a separate source of diffracted light. When the edges are very close to each other (when the slit is narrow) then (A) you get a macro-scale interference pattern from the diffracted light, and (B) very little un-diffracted light gets through the slit. When the edges are far apart, then the interference fringes are much smaller, and they may be overwhelmed by the much brighter, undiffracted light that passes through the wide opening. $\endgroup$
    – Solomon Slow
    Commented Sep 23 at 20:28
  • $\begingroup$ Thanks for the comment Solomon, maybe I'll reword it. $\endgroup$
    – Aidan
    Commented Sep 23 at 20:36
  • $\begingroup$ Also, if we take the Huygen-Fresnel principle, then every point on the wavefront acts like a source, so why would the edges of the slit acting as sources produce this radial pattern? $\endgroup$
    – Aidan
    Commented Sep 23 at 20:42
  • $\begingroup$ If you arrange a continuum of coherent, point sources along an infinite straight line, the sum of their wave functions gives parallel wave fronts moving perpendicular to the line. Now, cut off half of the line. Make it extend in one direction from an end point. The source at that end point has no neighbors on the one side to which its wave function can be added. Waves from that source radiate out in all directions, not just perpendicular to the line. That's what diffraction is. It's the edge of a slit cutting off half of a line of sources. physics.stackexchange.com/q/554972/74763 $\endgroup$
    – Solomon Slow
    Commented Sep 24 at 12:58

1 Answer 1

Reset to default
0
$\begingroup$

You should view the narrow slit as the "default" behavior. The narrow slit, if sufficiently narrow, acts like a point source. Waves propagate equally in all directions from it. That is how waves work and should not be surprising.

Viewed this way, the interesting question is why a wide slit does not act like a point source. And the Huygens-Fresnel principle explains this.

The wide slit acts like an array of emitters, all in phase with each other. A distant viewer directly in front of the slit sees all the emitters in phase or nearly so, because the distance to all of them is almost the same. Thus the viewer directly in front of the slit sees a bright signal.

However, for a viewer at a large angle, some of the emitters are further away than others. This means that they are seen with a time delay. The time delay can cause the emitters to be out of phase and therefore cancel each other out. This viewer receives a much fainter signal than the one directly in front of the slit.

Depending on the exact angle, the cancellation can be total (each emitter exactly cancels with another emitter 180 degrees out of phase with it) or partial (most emitters cancel but a few are left over). This causes the apparent brightness to change with angle, producing an alternating pattern of light and dark, i.e. "diffraction fringes." (This behavior is not shown in the highly simplified diagrams you've included in your question.)

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.