0
$\begingroup$

I know that the electric potential inside a conductor is always constant, regardless of anything surrounding the conductor.

Mathematically, I don't see how this makes sense. Please consider the following example:

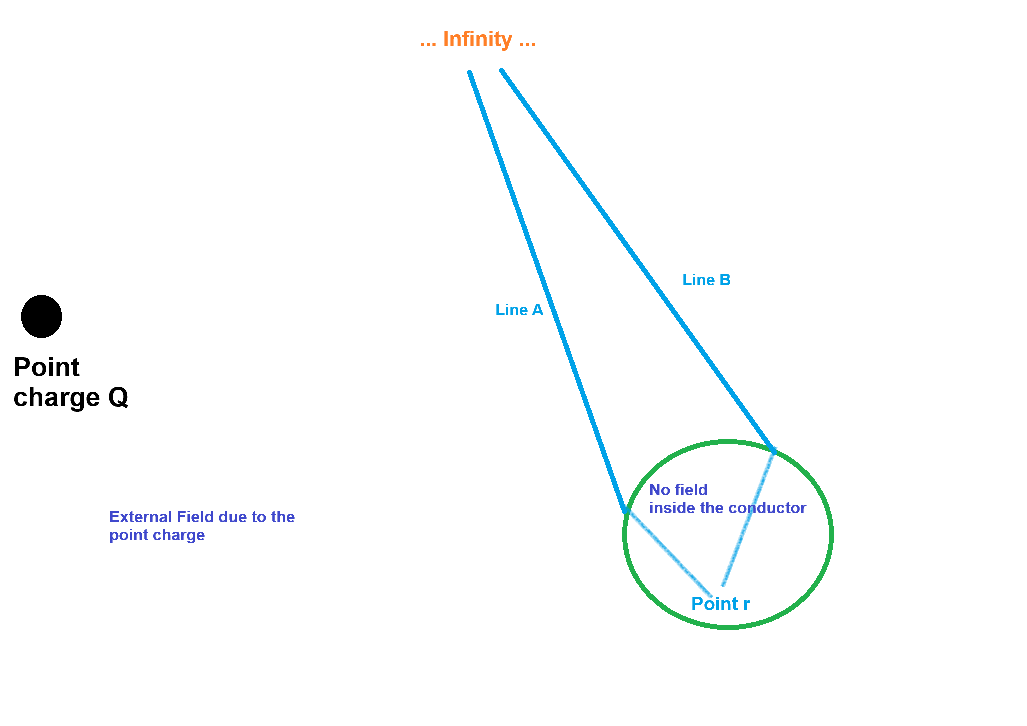
In the picture we have a conducting ball, and a point charge to its side.

The potential at point r, inside the ball, is given by a line integral from infinity to r. The value of the line integral in an electric field shouldn't depend on the line taken, as long as the start and end points are the same.

However - here I have taken two lines for example, line A and line B. Clearly the value of these line integrals can be different, because they do not finish at the same point, as far as the external field is concerned.

And in the internal 0 field of the conductor, of course no value is added to these integrals.

So how is it possible for the potential inside the conductor to be constant?

enter image description here

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ And what is the surface charge distribution doing in all this? $\endgroup$
    – Jon Custer
    Commented Sep 16 at 17:29
  • 2
    $\begingroup$ @JonCuster So the surface charge aligns itself in such a way not only to make the internal field 0, but also to make any line integral from infinity to the surface be equal some constant C? $\endgroup$
    – Aviv Cohn
    Commented Sep 16 at 17:34

1 Answer 1

Reset to default
0
$\begingroup$

I suspect that you're forgetting the induction by the point charge of surface charges on the conductor. The net charge on the conductor will remain zero, but, roughly speaking, the face of the conductor facing the point charge will become negatively charged and the opposite face, positive. These induced charges must be taken into account when calculating your external work integrals, and will ensure their equality.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So does this mean that any line integral from infinity to any point on the surface of the conductor will be equal to some constant A? $\endgroup$
    – Aviv Cohn
    Commented Sep 16 at 17:38
  • $\begingroup$ Yes its does indeed. $\endgroup$
    – Philip Wood
    Commented Sep 16 at 17:49

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.