0
$\begingroup$

I'm working on a version of coherance scanning interferometry (CSI) - very similar optically to a Michelson interferometer with a sample and reference beam combined to produce an interferance pattern - and in my setup would like to introduce a linear optical path difference across one spatial dimension (x) in my reference beam.

The illumination would be a light emitting diode (LED) with a full width half maximum (FWHM) of ~25nm. With high spatial coherence (that I would like to conserve) but low temporal coherance.

Is there a way to do this? I can only think to use a stepped reference mirror - but these can get very expensive and I'm sure there is a better way.

Essentially the question boils down to: how do you introduce an optical path difference in x to a low (temporal) coherence plane wave travelling in z, while maintaining the spatial coherence? Is this possible?

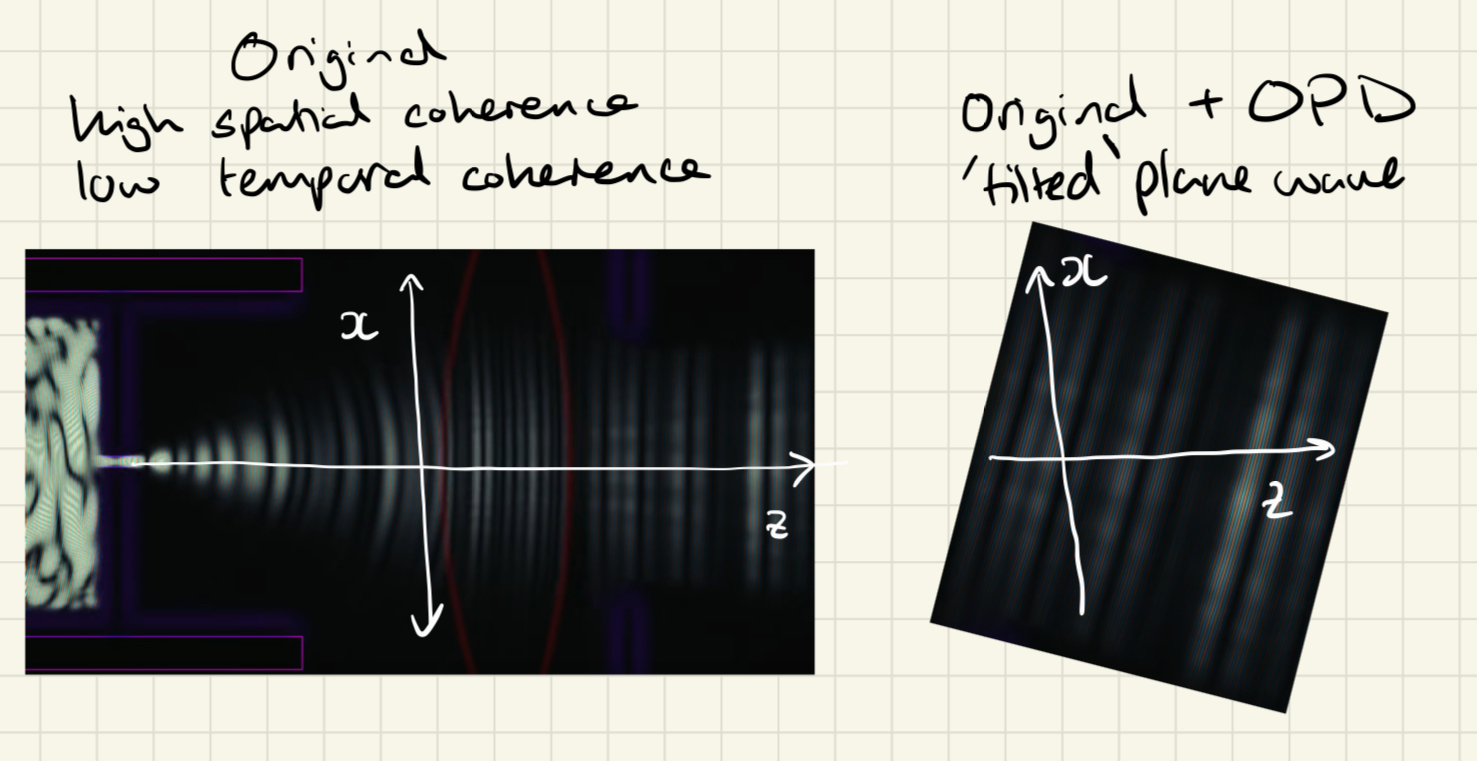Adapted from Hugens Optics, Youtube : https://www.youtube.com/watch?v=yMeRAg61_Wc Image adapted from Hugens Optics, Youtube : https://www.youtube.com/watch?v=yMeRAg61_Wc

Thanks in advance :)

Improve this question
$\endgroup$
1
  • $\begingroup$ Consider to spell out acronyms. $\endgroup$
    – Qmechanic ♦
    Commented Sep 10 at 16:44

1 Answer 1

Reset to default
0
$\begingroup$

Choose a plane tilted slightly with respect to the beam and you have a slightly longer path at one edge. One way to do this is with wedge prisms.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Perhaps I was ambiguous with the term coherence (question edited for clarity). Wouldn't a prism or grating change the temporal coherence? $\endgroup$
    – Jack Atkinson
    Commented Sep 11 at 8:37
  • $\begingroup$ I made a guess that you wanted to introduce a phase shift of < $2\pi$ across the beam and the temporal coherence length was longer than a wavelength. A pair of wedge prisms can make a window with parallel sides. This would delay the whole wavefront by the same amount and leave temporal coherence intact. If you twist one slightly, it forms a window with almost parallel sides. This delays one side of the beam slightly more than the other. The angle of a single wedge is only a few degrees. $\endgroup$
    – mmesser314
    Commented Sep 11 at 14:43

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.