0
$\begingroup$

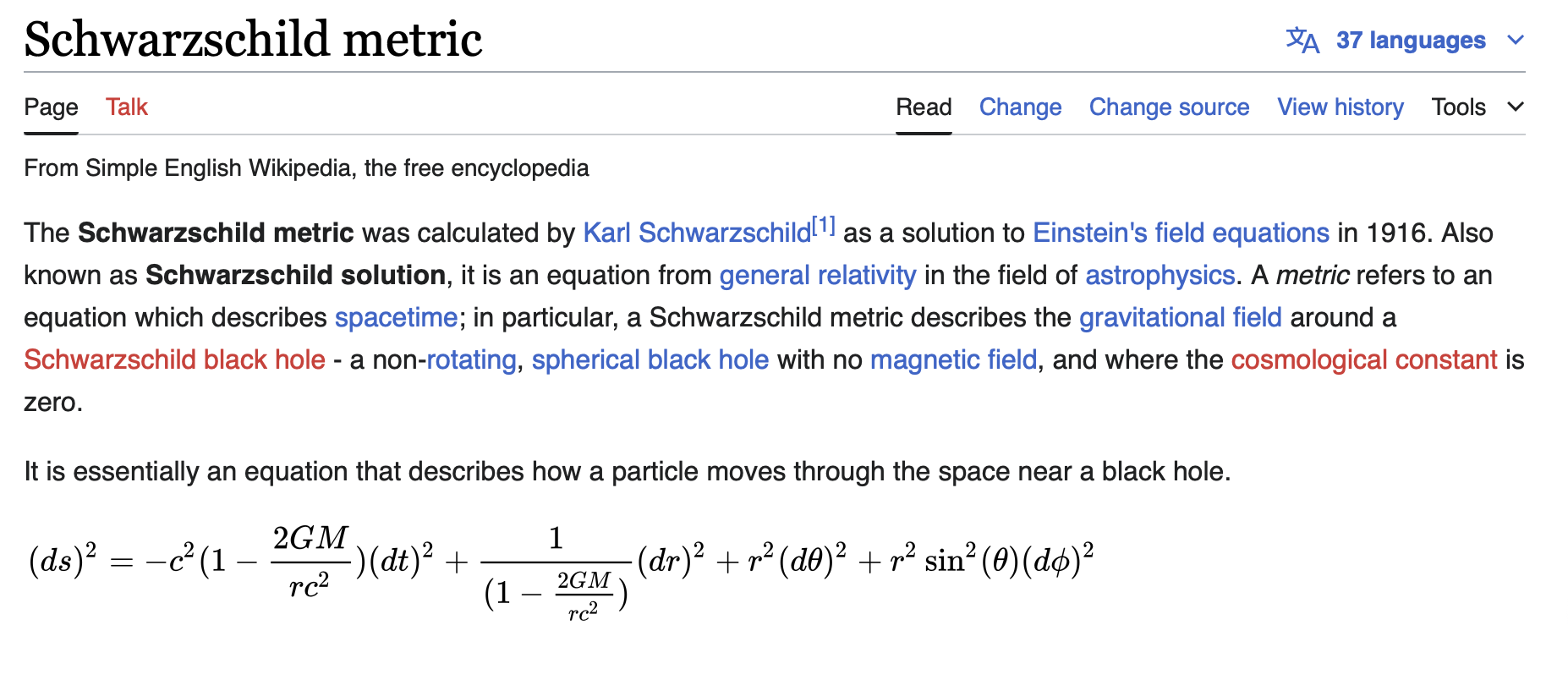
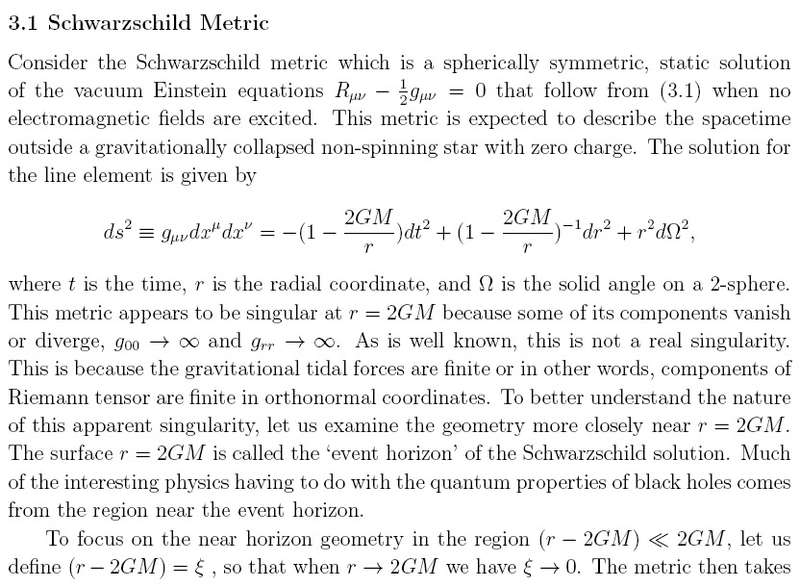
I started studying the Schwarzschild metric and have seen multiple versions of the metric online; specifically different versions of the Schwarzschild radius. In some cases it is $\frac{2GM}{c^2}$, other cases $2M$, and still other cases $2GM$. Even if they're interchangeable I'm curious how that could be since the units don't match.

To be fair I'm a bit out of my depth here so probably missing something simple.

I'll attach screenshots for reference, below:

enter image description here

enter image description here

enter image description here

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
4
$\begingroup$

The Schwarzschild radius is $$R= \frac{2GM}{c^2}$$ where $M$ describes the specific black hole we are analyzing and $G$ and $c$ are universal constants.

All of them are dimensionful units, so their values depend on the units selected. Physicists often choose units to simplify the calculations. For instance, in units where $c=1$ we get $$R= 2GM$$ and in units where both $c=G=1$ then $$R= 2M$$ In fact, it is always possible to choose units such that $$R=1$$

Any physical scenario is described by the dimensionless ratio $$\frac{r}{R}$$ This does not change regardless of the change of units used to simplify $c$, $G$, or $R$.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Thanks for the quick reply Dale, that makes sense. $\endgroup$
    – Fulfilmaker
    Commented Aug 4 at 14:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.