I have a background in aerospace engineering and, therefore, have dealt with the concept of a "center of pressure" for some time and have always found it quite intuitive and helpful. Recently, however, I have come across an example where my definition does not seem to work out anymore and I would argue that there is no center of pressure for that case at all which makes me question its applicability to 3D bodies altogether.
The respective wikipedia article (accessed 2024-07-26) starts out with:
In fluid mechanics, the center of pressure is the point on a body where a single force acting at that point can represent the total effect of the pressure field acting on the body.
The phrase "can represent the total effect of the pressure field acting on the body" means to me that a force acting at the center of pressure will affect the motion of the body (translational and rotational part) in the same way as the entire pressure field does.
From the translational equivalence, I would conclude that this force must be equal to the net force of the pressure field (to cause the same translational acceleration of the center of mass of the body). And from the rotational part, I would conclude that the center of pressure must be positioned such that this force causes a torque on the body that is equal to the torque created by the pressure field (to cause the same rotational acceleration of the body around the center of mass).
Now, imagine a windmill. A two-bladed one (like this one (accessed 2024-07-26)) suffices as an example. The following picture shows a simplified version of the rotor.
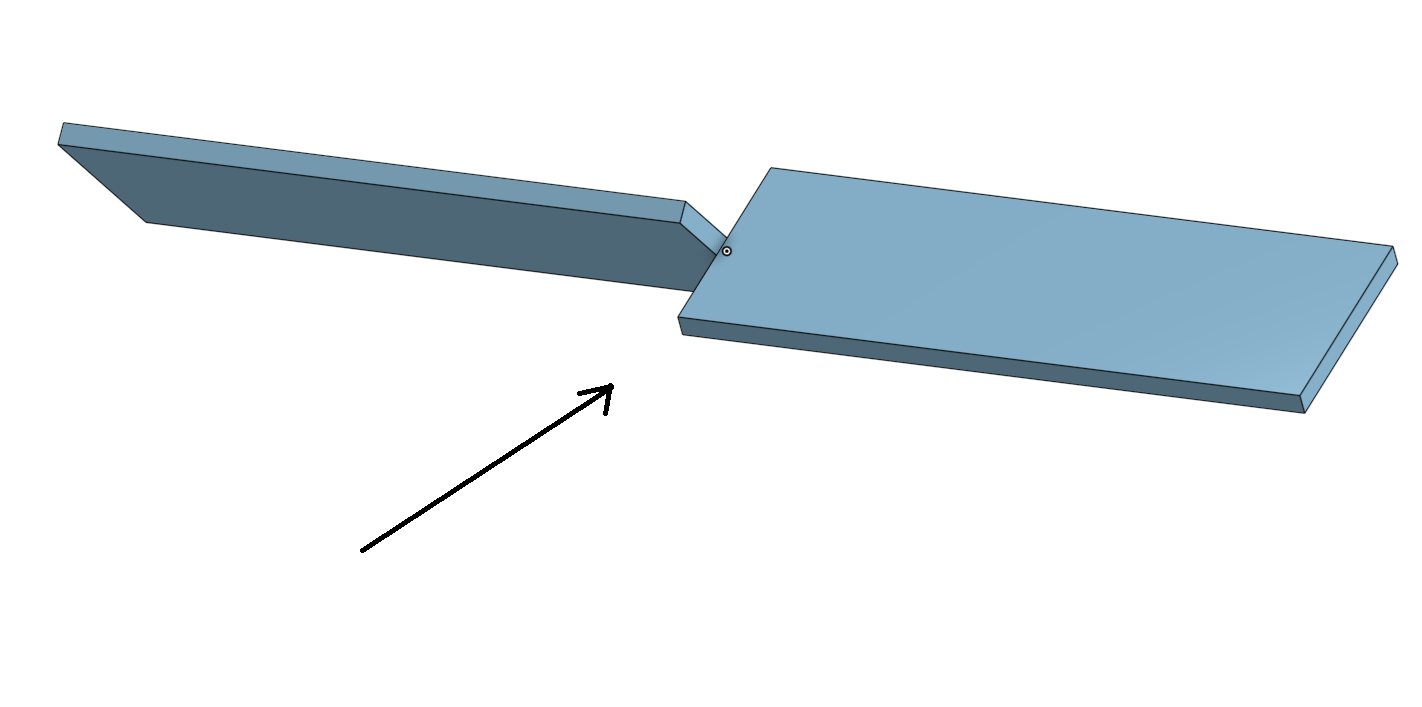
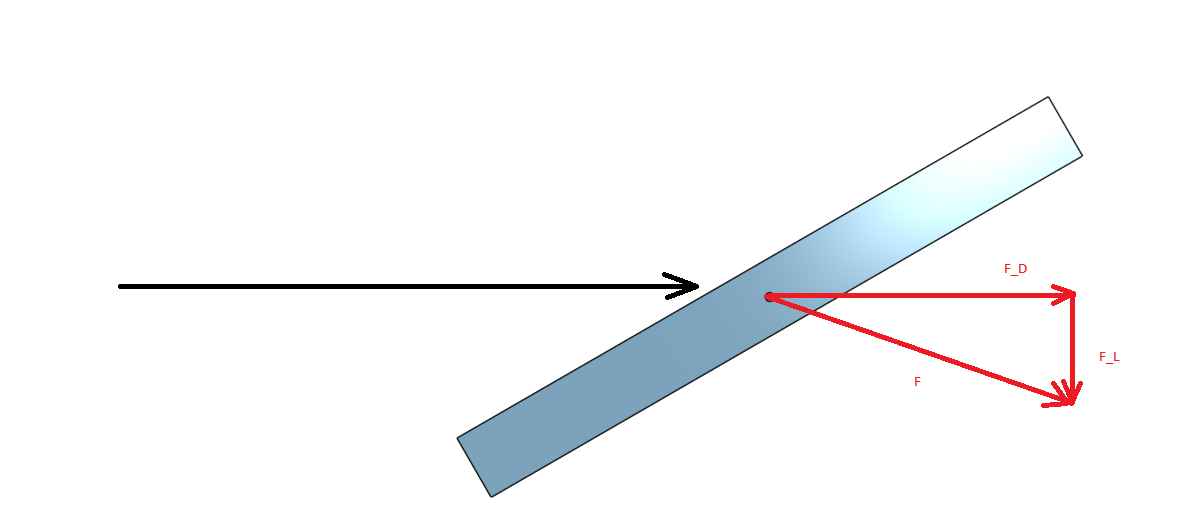
If air is blown at the rotor from the direction perpendicular to the plane of rotation and the rotor has not yet started moving (simplifies the analysis since the relative velocity of air w.r.t blade is equal to the wind velocity), this air flow will create a pressure field around the blade that causes a net force with a part parallel to the air flow (drag force) and a part perpendicular to the air flow (lift force). This is shown qualitatively in the following picture.
If we now look at the net force on the entire rotor, we can see that, due to the rotational symmetry, the lift force of one blade is oriented in the opposite direction of the lift force of the other blade. This symmtery has two consequences:
- The pair of forces causes the torque that would make the rotor spin in the plane perpendicular to the wind direction. So, the torque must be parallel to the wind direction.
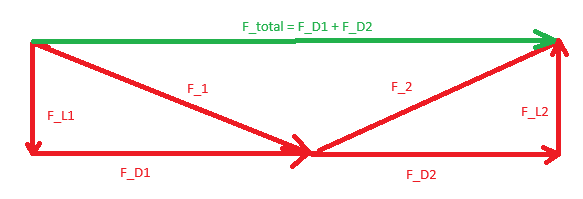
- The net force of the pressure field on the entire rotor has no portion perpendicular to the wind direction because the two lift forces cancel each other out in a sum (as shown in the following picture).
In summary, we can conclude that the net torque and the net force of the pressure field on the entire rotor both are parallel to the wind direction.
According to the aforementioned definition of the center of pressure we should be able to find a point $r_\text{cop}$ where the net force $F_\text{net}$ could act on the body so that it creates the net torque $\tau_\text{net}$:
$$\tau_\text{net} = r_\text{cop} \times F_\text{net}$$
A property of the above cross product is that the resulting vector $\tau_\text{net}$ is always perpendicular to both $r_\text{cop}$ and $F_\text{net}$. But we concluded that $\tau_\text{net}$ and $F_\text{net}$ are parallel for the windmill.
This leads me to conclude that there is no point where we could move the net force so that it would create the net torque. So, there is no center of pressure according to the definition mentioned in the beginning.
I would be surprised if windmills are the only bodies where one could make an argument like this.
Question: Is this conclusion correct? So, what is the set of all bodies for which the concept of a center of pressure is applicable? Those, where the net torque is perpendicular to the net force?
Further thoughts:
I have been introduced to the concept of a center of pressure in the context of airfoils. Here, we typically look at the 2D cross section of an airfoil with the wind direction in the same plane. Here, the above problem does not occur because all forces are in the same plane and thus the rotation takes place in the same plane. The torque is consequently perpendicular to this plane and can never have a portion parallel to the net force.
However, I have never seen a warning that the center of pressure is only a valid concept in 2D. On the contrary, the wikipedia article talks about bodies which implies the applicability to the 3D objects. Furthermore, I have read papers on satellite aerodynamics (M. A. Frik, “Attitude stability of satellites subjected to gravity gradient and aerodynamic torques,” AIAA Journal, vol. 8, no. 10, pp. 1780–1785, Oct. 1970, doi: 10.2514/3.5990. ) that argue with the position of center of pressure of an entire satellity. So, it is used in 3D.
Edit 1: replaced links with those from internet archive to preserve state for future generations Edit 2: made the question more focused