Calculate quadrotor propeller torque due to aerodynamic drag

Question

I'd like to know the right formula to calculate torque of a quadrotor propeller (propeller pitch is constant) due to aerodynamic drag in terms of angular velocity $ \omega $. For the sake of my simulation I'm assuming APC 10x4,7 propellers. I'd like to refer to UIUC Propeller Data Site, so please assume only the set of data given there is available while answering. I know really little of aerodynamics, I'm planning to design a control system in Simulink later on, but would like to derive a non-linear mathematical model first.

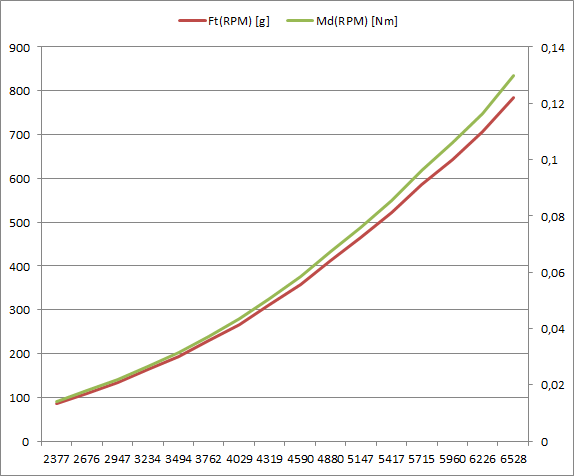
Please note I need to use data from UIUC Propeller Data Site for APC 10x4,7, which means the propeller below. I'm guessing all is there to derive a formula $\tau_d(\omega)$, I just don't understand how to do it.

EDIT: By now I figured out, lift force of a wing can be calculated as:

$ F_T = C_T \rho A \frac{V^2}{2} $,

where $C_T$ is lift coefficient, $\rho$ means air density, $A$ is wing total area and $V$ is relative air speed. I also asked a friend who told me, for a helicopter propeller this transforms into:

$F_T(\omega) = C_T \rho A r^2 \omega^2 = C_T \rho \pi r^4 \omega^2 $,

with $A = \pi r^2$ being the area of the rotor, $r$ being its radius and $\omega$ being the propeller's rotational velocity. I guess, assuming the latter formula is true, one could derive one for drag force from it substituting $C_D$ for $C_T$ and rotor blade's frontal area (its projection, rather) for $A$. Could this be right?

Thrust and drag coefficients from UIUC:

RPM    CT       CP
2377   0.1039   0.0431
2676   0.1058   0.0437
2947   0.1059   0.0437
3234   0.1083   0.0444
3494   0.1096   0.0450
3762   0.1121   0.0460
4029   0.1136   0.0466
4319   0.1155   0.0474
4590   0.1177   0.0484
4880   0.1199   0.0494
5147   0.1213   0.0500
5417   0.1228   0.0508
5715   0.1239   0.0513
5960   0.1253   0.0520
6226   0.1261   0.0524
6528   0.1274   0.0531

Geometry data from UIUC below. I believe, $c$ is aerodynamic chord length, $R = 0.127m$ (propeller radius), $\beta$ is propeller's pitch at the respective point. I believe I can calculate propeller's side area using simple quadratic integration rule. But first I need to know my reasoning is right.

r/R    c/R     beta
0.15   0.109   21.11
0.20   0.132   23.90
0.25   0.156   24.65
0.30   0.176   24.11
0.35   0.193   22.78
0.40   0.206   21.01
0.45   0.216   19.00
0.50   0.223   17.06
0.55   0.226   15.33
0.60   0.225   13.82
0.65   0.219   12.51
0.70   0.210   11.36
0.75   0.197   10.27
0.80   0.179   9.32
0.85   0.157   8.36
0.90   0.130   7.27
0.95   0.087   6.15
1.00   0.042   5.04

Answer 1

OK, I figured out all my mistakes and the results seem sensible now.

I was using wrong formulas and, first of all, was not using imperial units which is necessary with UIUC's data. The right formulas are:

$F_T(RPS) = C_T \rho n^2 D^4$,

where: $\rho [\frac{slugs}{ft^3}] = 0.0023769$ is air density, $n[RPS]$ is prop angular speed in revolutions per seconds, $D[ft] = \frac{10}{12}$ is prop diameter. For calculating torque, the below formula must be used:

$\tau(RPS) = \frac{C_P \rho n^2 D^5}{2 \pi}$.

The results obtained seem sensible and in synch with e.g. Drive Calculator data. Some graphs and screenshots below. Of course, the results are all in imperial units, I converted them to SI for graphs.

Answer 2

Just a shot in the dark. Assuming each propeller blade is a reasonably efficient airfoil, it should have a lift-to-drag ratio of (pick a number out of the air) 5. OK, so take the weight of the aircraft, divide by 5, and that should be the total drag on all the blades, at a radius of maybe 3/4 of the blade length. That should give a rough estimate of the torque.