In Black Hole Spectroscopy, it is well known that the Pöschl-Teller (PT) potential behaves approximately, or similarly to the more complicated Regge-Wheeler (RW) Potential.
The WKB Approximation has been extensively applied to RW to calculate its quasi-normal mode spectrum, but I have not seen anything in the literature applying it to the PT potential.
Has this been done, if so what does the approximation yield, and if not - is there some technical challenge that prevents its solution?
Schrödinger operators with reflectionless Pöschl-Teller potentials arise in the study of SUSY chains of one-dimensional non-linear field theories labeled by $N$. The two first members of the family are the sine-Gordon model and the $\phi^4$-theory. The spectrum consists of $N-1$ bound states and a tower of continious modes. Such models are usually soved using the WKB-method. It also arises as Tachyon potential in the (guessing closed) bosonic string theory.
Barton Zwiebach JHEP09(2000)028 equation 2.15 is the chain I talked about.
A. Alonso-Izquierdo, J. Mateos Guilarte, On a family of (1+1)-dimensional scalar field theory models: Kinks, stability, one-loop mass shifts, Annals of Physics, Volume 327, Issue 9,2012
Okay so this question was really bugging me so I spent the afternoon learning how to do the approximation.
The general Poschl-Teller Potential is $V(x) = \frac{V_o}{\cosh^2(\alpha \cdot (x-x_0))} $
I take $ V_0 = 1, \alpha =1, x_0 = 0 \ \ \ \ \rightarrow \ \ \ \ V(x) = \frac{1}{\cosh^2(x)} = \rm{sech}^2(x)$
Schutz and Will derive a general first order WKB approximation for QNMs:
$ \frac{Q_0}{\sqrt{2Q_0''}} = i(n+\frac{1}{2})$
This expands to:
$ \frac{w^2-\text{sech}^2(x)}{\sqrt{2} \sqrt{2 \text{sech}^4(x)-4 \tanh ^2(x) \text{sech}^2(x)}} = i(n+\frac{1}{2}) $
I evaluate at the peak of the potential, $x=0$, and solve for w taking the negative solution:
$ w = -\sqrt[4]{-1} \sqrt{2 n+(1-i)} $
I also reflect it across the imaginary axis to get the following spectrum, (vertical axis is the Im(w), and horizontal axis is Re(w)):
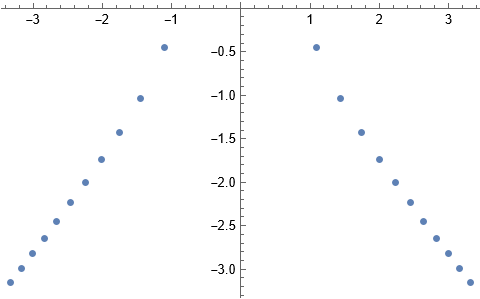
Compare this against the analytical derivation included by Berti, Cardoso, and Starinets:
$ \omega = \sqrt{1-\frac{1}{4}}-\frac{1}{2} i (2 n+1) $
Reflecting this as well yields the following spectrum, (again vertical axis is the Im(w), and horizontal axis is Re(w)):
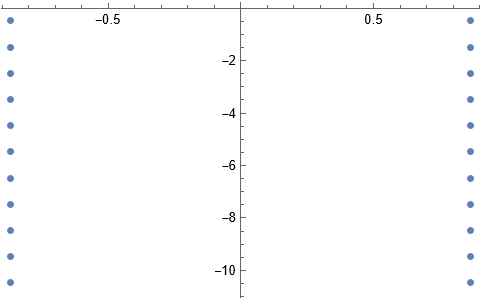
As is known the first order WKB is not terribly acurate, the fundamental mode is close, $-1.09868 - 0.45509i$ vs $-0.866025 - 0.5i$, but the asymptotic behavior is different, and there is significant deviation in the real value for the overtones.
