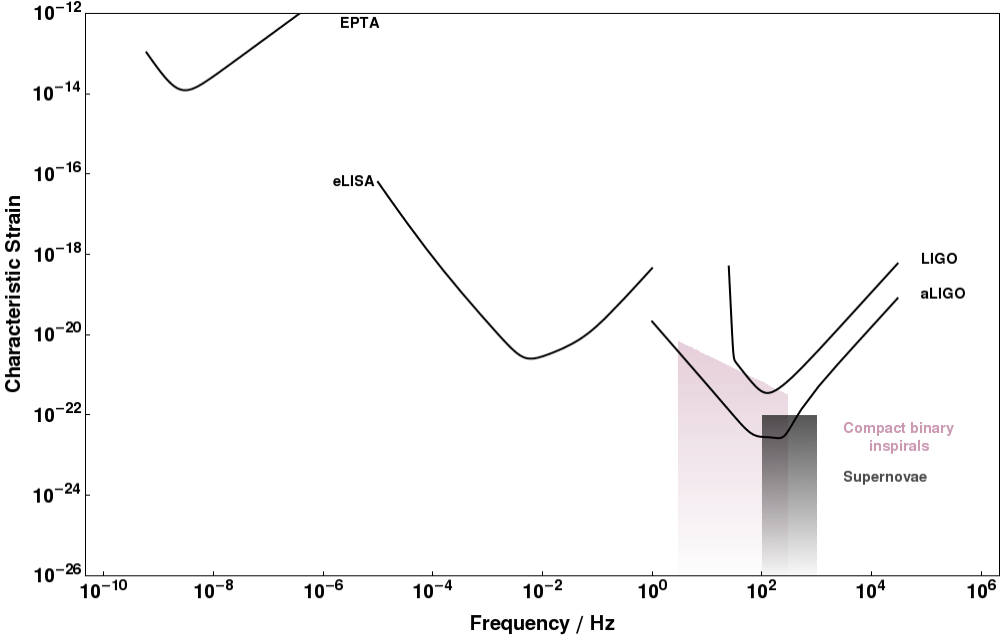The amplitude of the strongest gravitational wave signal detected by LIGO sofar can maybe be expressed as an acceleration? If so, what would the numerical value be (in m/s^2)? I would like to compare it to the effect of moving masses near the detector, just to have a better reference than the usual 'displacement of a fraction of an atom's radius'. Reference: Do Gravitational Waves cause a 'Rate of change of Acceleration'?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
The best I can do is a crude estimate.
Wikipedia's LIGO article shows this graph.
From this, you can say LIGO is most sensitive at about 100 Hz, and the limit of detection is when $\Delta l/l \approx 10^{-23}$.
Since $l = 4000$ m, $\Delta l = 4 \cdot 10^{-20}$ m.
LIGO can detect a wave if its mirror oscillates in a sine wave with max acceleration $4 \cdot 10^{-18}$ m/s$^2$, or $4 \cdot 10^{-19}$ g.
But Characterstic strain is only loosely related to the acceleration of the mirror. See Gravitational-wave sensitivity curves
-
$\begingroup$ You seem to be arguing in the opposite direction: What is the smallest acceleration that LIGO can measure. $\endgroup$– TimRiasCommented May 29 at 7:57
-
$\begingroup$ @TimRias - True. But you can see from the shaded areas of the graph that is near the upper end of the gravitational waves we expect to see. $\endgroup$ Commented May 29 at 13:22