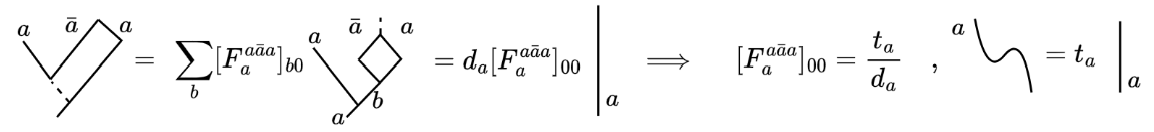TL;DR: The "rigidity equation" you have stated is redundant in the physical setting. It is guaranteed because your $F$-matrices are unitary solutions to the pentagon equation.
Notation warnings: (1) I'm going to use $\bar{a}$ to denote the 'antiparticle' of $a$ (instead of the $\hat{a}$ notation used in your original post). (2) I'll use $0$ to denote the vacuum particle (instead of the $1$ notation you've used). Sorry about that, it's only so that I can be consistent with the diagrams I've already got lying around (which I'll use below).
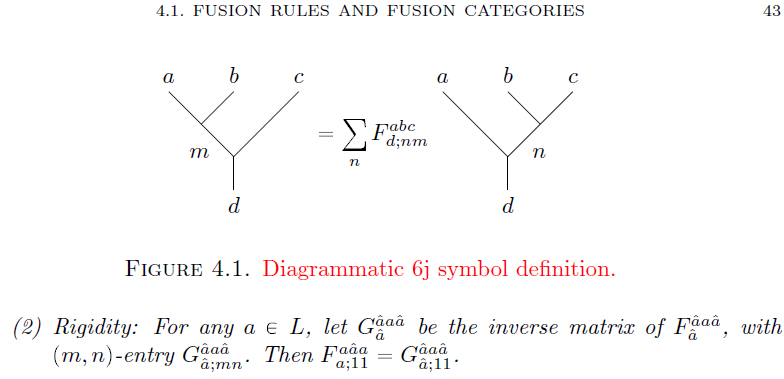
Let's take a closer look at that 6j-symbol.
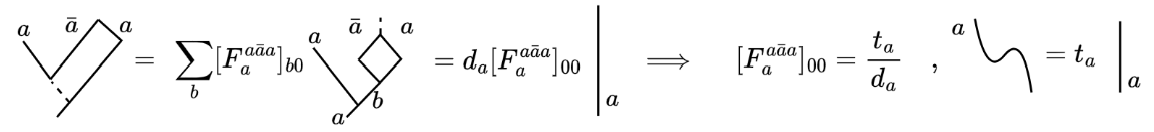We have $d_{a}[F^{a\bar{a}a}_{a}]_{00}=t_{a}$, where $d_{a}$ is the value of the loop labelled by $a$, and $t_{a}\in \mathrm{Hom}(a,a)$ is the cost of straightening out the illustrated zigzag. The number $d_{a}$ is called the quantum dimension of $a$, and the number $t_{a}$ is called the pivotal coefficient of $a$.
In the physical setting where our labels correspond to types of anyons, all of the Hom-spaces are state spaces of particles. We know from the postulates of quantum mechanics that all state spaces must carry a Hermitian inner product. To use some terminology from Wang's book, this means that all 'triangular spaces' are equipped with such an inner product. Notice that the quantity $d_{a}$ can be viewed as the squared norm of a nonzero vector in $V^{\bar{a}a}_{0}$, whence we know $d_{a}>0$. Since $d_{a}$ is nonzero, we can safely divide by $d_{a}$ on both sides (as we do in the $\implies$ step in the image above).
Recall that the quantum dimension satisfies $d_{a}=d_{\bar{a}}$. This can be seen in two different ways:
(1) Every unitary 6j fusion system is 'spherical' (i.e. left and right traces coincide): so here, just apply this sphericality property to the identity strand $\mathrm{id}_{a}$. I believe Wang talks about sphericality in his book. In the language of fusion categories, we're using the fact that every unitary fusion category has a canonical spherical structure. This machinery is perhaps a little overpowered for showing $d_{a}=d_{\bar{a}}$, so let me provide an alternative perspective in (2).
(2) In the unitary setting (which we assume because we're working with particles here), $d_{a}$ is the largest positive eigenvalue (a.k.a Frobenius-Perron eigenvalue) of the fusion matrix $N^{a}$ (not sure if you've seen this?). To clarify, for labels $a,b,c$, we define $N^{ab}_{c}:=\dim(\mathrm{Hom}(a\otimes b,c))$ and $[N^{a}]_{bc}:=N^{ab}_{c}$. Using some properties of the fusion coefficients (exercise!), we see that $N^{\bar{a}}=(N^{a})^{T}$, which gives us $d_{a}=d_{\bar{a}}$.
Now let's look at that "rigidity condition". This demands that
$[G^{\bar{a}a\bar{a}}_{\bar{a}}]_{00}=[F^{a\bar{a}a}_{a}]_{00}$
which by unitary (since we're in physics land here) of the $F$-matrix is the same as demanding
$[F^{\bar{a}a\bar{a}}_{\bar{a}}]^{*}_{00}=[F^{a\bar{a}a}_{a}]_{00}$
where I use $z^{*}$ to denote the complex conjugate of $z\in\mathbb{C}$. Using our formula from above, we have converted the rigidity condition into
$ t_{\bar{a}}^{*}=t_{a} \tag{1} $
This condition already turns out to be true in the unitary setting! Let's outline why this is so. Kitaev proves (Theorem E.6 in this paper) that whenever you have some unitary $F$-matrices associated to a label set that satisfy the pentagon/triangle axioms, an equation known as the "pivotal identity" (see Fig 16 in that paper) holds, and that any 'leg-bending' operators (defined at the start of Sec. E.2.3. in that paper) are unitary. Using the unitarity of the leg-bending operators, you can check that $|t_{a}|=1$. Then using the pivotal identity, you can also deduce that $t_{\bar{a}}t_{a}=1$. This is equation (1) above!
Other notes:
(i) This is what Cui means when he says the rigidity condition is redundant if $[F^{a\bar{a}a}_{a}]_{00}\neq0$. If this 6j-symbol vanishes, we are working with a 6j fusion system where the triangular spaces do not admit an inner product (i.e. the F-matrices of the planar algebra cannot be made unitary).
(ii) Vague comments: I think the term 'rigidity' might be out of vogue in this context? Nowadays, rigidity typically refers to a monoidal category whose objects all have left/right duals satisfying the 'snake equations'. The snake equations tell us that we can pull certain kinds of zigzags straight. Wang's rigidity equation relates to pulling straight other kinds of zigzags (which instead relies on the existence of a natural isomorphism between an object and its double dual) that exist in 'pivotal' categories. This so-called pivotal structure is an extra piece of structure that a rigid category may or may not have. The categories that describe anyon theories always possess this 'pivotal structure' (which means that anyons satisfy CPT symmetry!).
