This link suggests that one cannot make a free theory out of anyons, because of its Lorentz representation.
How exactly does the $SO(2,1)$ representation enforce the $\pm1$ eigenvalues?
How can one make anyons experimentally then, with interactions to make the $SO(2,1)$ representation not applicable?
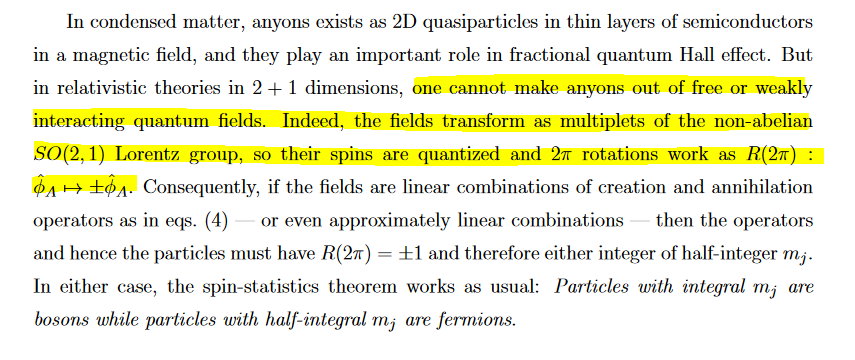
In quantum theory free, particles correspond to irreducible representations of the Poincaré group. In $2+1$ dimensions, it is the group $SO(2,1) \rtimes \mathbb{R}^3$ (The senond component is the translation part).
For a classical system with a symmetry group $G$, its quantum counterpart allows representations of the universal covering group $\bar{G}$. This is the reason of the existence of half integer spin, where the rotation group $SO(3)$ is enhanced in quantum mechanics to $SU(2)$ having spinor representations.
The universal covering of the Lorentz group is the spin group:
$$\overline{SO(2,1)} = \mathrm{Spin}(2,1)$$
The group $\mathrm{Spin}(2,1)$ is semisimple and thus allows only for integer and half integer spin finite dimensional representations.
However, in the classical limit the Lorentz group is contracted to the Euclidean group:
$$SO(2,1) \rightarrow SO(2)\rtimes\mathbb{R}^2$$
$$\overline{SO(2)} = \mathbb{R}$$
where the first factor is the rotation and the second factor is the boosts. But here the universal covering of the rotation group is the whole real axis, which allows noninteger representations.
Thus in nonrelativistic $2+1$ quantum mechanics fractional and even irrational spin is allowed for free particles.
Please see also a previous answer of mine, where a similar problem was addressed.
