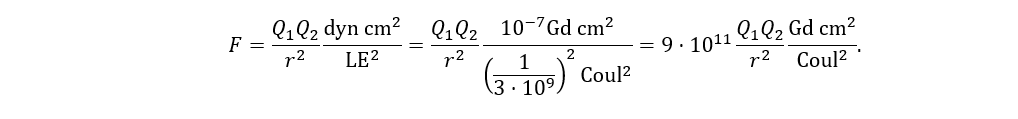I recently translated the appendix to an electromagnetics text from 1945 into English. Now the client is asking me to update the formulas (I studied electrical engineering). The formulas use CGS units and I want to convert to SI units.
I am not very familiar with the CGS system and am baffled by this equation (Coulomb's law). For example, why does $Coul^2$ appear in the bottom, but $Q_1Q_2$ is in the top?
Here is a little more context for this formula:
Any suggestions?
Coulomb's law gives the force between two charges. This force is proportional to each of the two charges, and inversely proportional to the square of the distance between them. So, we will have some expression like the following:
$$F=k\frac{Qq}{r^2}$$
Where $k$ is some proportionality constant whose value depends on the system of units. It is a constant of nature and its value must be determined experimentally. Recall that Coulomb's law tells us the force experienced by the charges. The LHS clearly already has dimensions of force ($[\mathrm{MLT}^{-2}]$). The RHS has dimensions $[\mathrm{X}][\mathrm{T}^2\mathrm{I}^2\mathrm{L}^{-2}]$, where $[\mathrm{X}]$ are the unknown dimensions of $k$. Because the RHS must also have dimensions of force, we can find what the dimensions of $k$ must be: $$[\mathrm{X}]=\frac{[\mathrm{MLT}^{-2}]}{[\mathrm{T}^2\mathrm{I}^2\mathrm{L}^{-2}]}=\frac{[\mathrm{MLT}^{-2}][\mathrm{L}^2]}{[\mathrm{T}^2\mathrm{I}^2]}$$ which are the dimensions of the units you see in the text.
This is all to say that the units you see in the text are the units of the proportionality constant - in the specific case from your question, it is telling you how many force units $\mathrm{Gd}$ the charges experience per unit $\mathrm{C}^2$ of the product of their charges (as well as how it scales down per unit $\mathrm{cm}^2$ of the square of the distance between them).
