Electric field intensity outside a hollow conducting sphere with charge at the center

Question

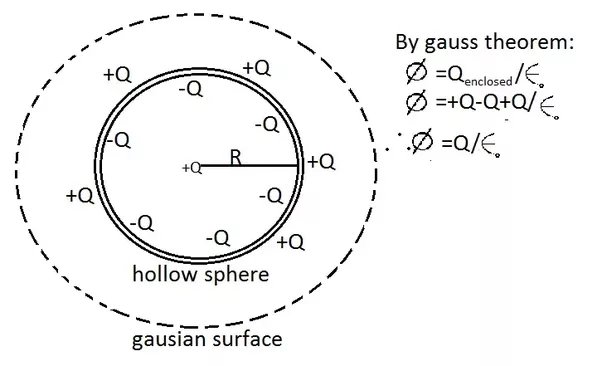
I'm trying to understand the electric field intensity outside a hollow conducting sphere with a charge +Q placed at the center. The sphere has an inner radius an and an outer radius b.

My instructor mentioned that the electric field intensity "picks up" at r = b right where it "fell off" at r = a. However, I'm having trouble understanding why the electric field intensity isn't greater at r > b than at r = a. The +Q charge appears much closer at say r = b+0.00001 m than at r = a.

Since a +Q charge is induced at the outer surface (r = b), I would expect the electric field intensity to be greater than at r = a beyond r = b. Even after considering the -Q and +Q charges at the center, I can't see why it wouldn't be greater than at r = a.

Can someone help clarify this concept for me?

Answer 1

In the diagram the flux $\phi = \frac Q \epsilon$ everywhere except inside the conduction shell where the flux is zero.

$E\, A = \phi$ where E is the electric field passing through a spherical area $A$ centered on charge $+Q$ at the center and of radius $r$.
At $r=a$ the inner surface of the conduction shell $E_{\rm inner} \cdot 4 \pi a^2 = \frac Q \epsilon$ and at $r=b$ the outer surface of the conduction shell $E_{\rm outer} \cdot 4 \pi b^2 = \frac Q \epsilon$.
As $b>a$ then $E_{\rm inner}> E_{\rm outer}$

I assume that the instructor said that the field "fell off" to zero inside the conduction shell and then "picks up" from zero outside the shell but with no comparison made between the field inside the shell and the field outside the shell.

The +Q charge appears much closer at say r = b+0.00001 m than at r = a.
You have not considered the effect of the "close" induced negative charges on the inner surface of the shell.

Answer 2

For a quick solution:

In any given electric field in vacuum it is possible to introduce a metallic thin sheet along an equipotential surface without changing the field outside the metal volume.

This is merely a rephrasing of Maxwells definition of the $D$-field at a conductor as the surface charge density. By its fixed linear coupling the force field $E$, Maxwell concluded, that it exists everywhere in vacuum, too, together with $E$.

The physical effect is simply, that on both surfaces of the metal the directed normal of the vacuum $D$-field is cut off by the boundary charge density.

The adaption of the surface charge density to the external field is coming at energy cost of zero in the metallic conduction band of free electrons, at least in the limit of time independent fields.