Origin at center of mass
For a single particle, angular momentum is defined similarly to torque, as the moment-of-momentum, just as torque is moment-of-force. Both correspond to a measurement of something done with respect to the origin.
$$\begin{array}{c|c}
\text{Quantity} & \text{Definition} \\
\hline
\text{torque} & \boldsymbol{\tau} = \boldsymbol{r} \times \boldsymbol{F} \\
\text{ang. momentum} & \boldsymbol{L} = \boldsymbol{r} \times \boldsymbol{p} \\
\end{array}$$
where $\boldsymbol{r}$ is the position vector of the thing measured relative to the origin, $\boldsymbol{F}$ is a force applied somewhere, and $\boldsymbol{p}$ is the (translational) momentum of a particle.
In a similar fashion to considering the torque as a result of a distributed force as
$$ \boldsymbol{\tau}_{\rm net} = \sum_{i} \boldsymbol{r}_i \times \boldsymbol{F}_i \tag{1}$$
angular momentum also can be summed up when dealing with a collection of particles
$$ \boldsymbol{L}_{\rm net} = \sum_{i} \boldsymbol{r}_i \times \boldsymbol{p}_i = \sum_i \boldsymbol{r}_i \times (m_i \boldsymbol{v}_i) \tag{2}$$
where $m_i$ is the mass of the particle and $\boldsymbol{v}_i$ is the (translational) velocity of the particle.
Now consider the kinematics of a general rotating rigid body consisting of multiple particles fixed with each other.
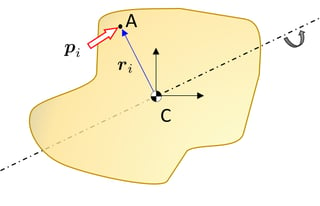
The velocity of each particle can be expressed as
$$\boldsymbol{v}_i = \boldsymbol{\omega} \times \boldsymbol{r}_i \tag{3}$$
which makes the summing of angular momentum be
$$\boldsymbol{L}_{\rm COM} = \sum_i \boldsymbol{r}_i \times m_i ( \boldsymbol{\omega}\times \boldsymbol{r}_i ) \tag{4}$$
Here the vector triple product identity $a\times(b\times c) = b (a\cdot c) - c (a\cdot b)$ is used to factor out the common term $\boldsymbol{\omega}$ since it is shared among all particles.
$$\boldsymbol{L}_{{\rm COM}}=\left[\sum_{i}m_{i}\left(\left(\boldsymbol{r}_{i}\cdot\boldsymbol{r}_{i}\right){\bf 1}-\left(\boldsymbol{r}_{i}\odot\boldsymbol{r}_{i}\right)\right)\right]\boldsymbol{\omega} \tag{5}$$
where $\bf 1$ is the identity matrix, and $\odot$ the outer product operator
Commonly the big sum is pre-evaluated since it depends on the geometry only and not on the operational circumstances of the problem. This gives rise to the definition of the mass moment of inertia tensor.
$$ {\rm I}_{{\rm COM}}=\sum_{i}m_{i}\left(\left(\boldsymbol{r}_{i}\cdot\boldsymbol{r}_{i}\right){\bf 1}-\left(\boldsymbol{r}_{i}\odot\boldsymbol{r}_{i}\right)\right) \tag{6}$$
which is applied as
$$\boldsymbol{L}_{{\rm COM}}={\rm I}_{{\rm COM}}\boldsymbol{\omega} \tag{7}$$
Origin at an arbitrary place
When doing the above sum, but about an origin not at the center of mass, then there is an additional term that comes out, relating to the total (translational) momentum
$$\boldsymbol{p} = \sum_i m_i \boldsymbol{v}_i = {\rm M}\, \boldsymbol{v}_{\rm COM} \tag{8} $$
when going step by step you find that angular momentum summed about the origin (at the arbitrary point A) is
$$\boldsymbol{L}_{A}={\rm I}_{{\rm COM}}\boldsymbol{\omega}+\boldsymbol{r}_{{\rm COM}}\times\boldsymbol{p} \tag{9}$$
It is worth going through this derivation on your own in order to really understand it on your own terms.