0
$\begingroup$

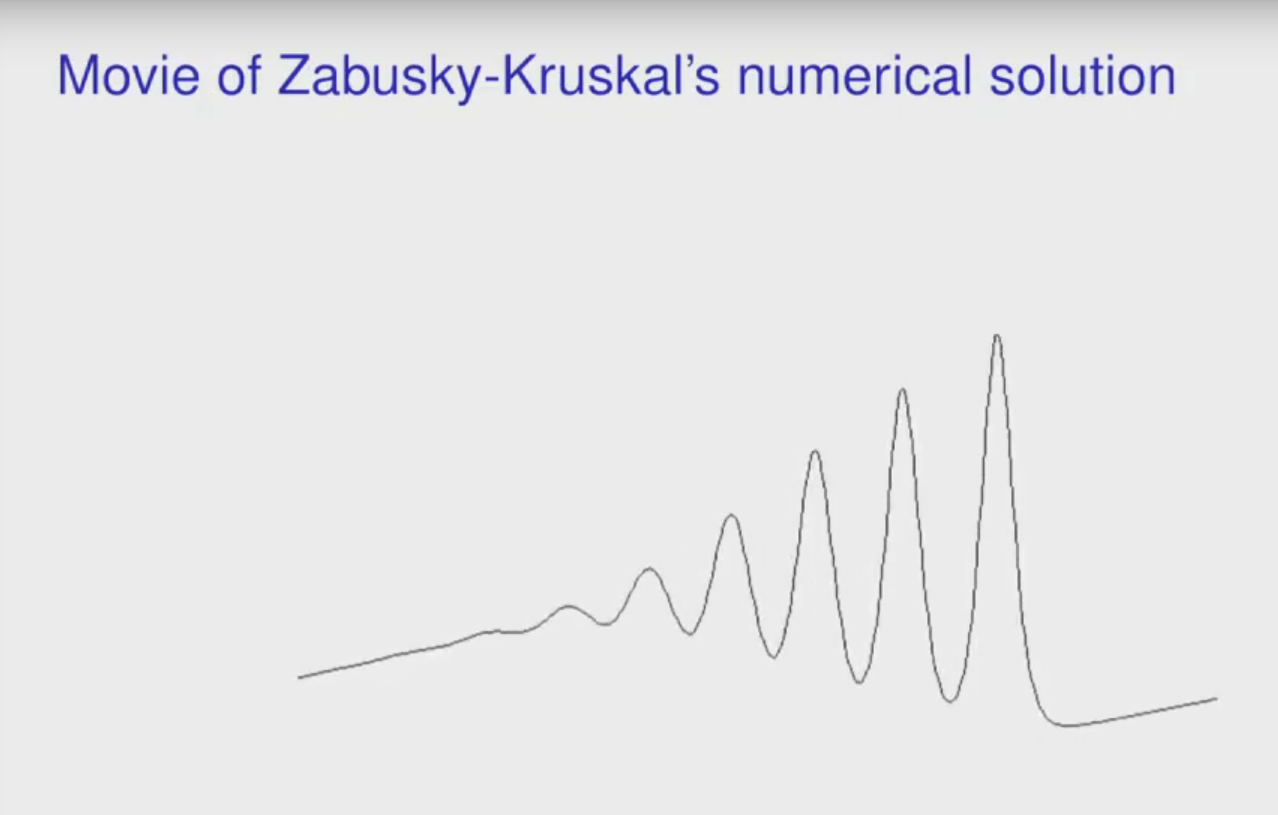
There are lots of examples of oscillatory phenomena in nature the description of which boils down to simple harmonic behavior, i.e. to Cosine/Sine/Complex Exp. This answer explains that we use sines and cosines because of their convenience and ease of mathematical description of phenomena. For example, complex exponentials are eigenfunctions of the differentiation operator, so linear differential equations turn into algebraic ones. In other words, we usually describe the complex vibration of a drum as a sum of sinusoidal modes, because it is convenient for us. But these drum vibrations could also be described as the sum of triangular or rectangular modes or any other modes (as long as the corresponding functions form a complete basis).

But my question is different: are there such physical systems in which harmonic waves, described by sines and cosines, cannot exist? Let me rephrase it. For example, we consider an oscillating system. It can be linear, and then its dynamics are described by a linear differential equation. It can also be nonlinear, then we can linearize the system and consider the stability of the stationary state. In any case, we arrive at eigenvalues and eigenfunctions. Is it possible that sines and cosines are not proper functions for this system? Maybe you know at least a couple of examples of such systems? Occasionally, in the literature on mechanics, I come across that there is a class of phenomena, the description of which is not limited to the study of the properties of individual harmonic waves. But no examples are given...

Improve this question
$\endgroup$
4
  • $\begingroup$ Would this work: solving the multidimensional Laplace equation on finite rectangular domains necessitates using a combination of both trigonometric and hyperbolic trigonometric functions. Another might be the quantum harmonic oscillator Schrödinger equation. $\endgroup$
    – Cameron Williams
    Commented Mar 24 at 16:12
  • $\begingroup$ Please clarify your specific problem or provide additional details to highlight exactly what you need. As it's currently written, it's hard to tell exactly what you're asking. $\endgroup$
    – Community Bot
    Commented Mar 24 at 16:15
  • $\begingroup$ @CameronWilliams Do you mean that the domain, in other words, the boundary conditions can affect the fact that the sine and cosine functions of the linear Laplace equation may not be solutions? $\endgroup$
    – shamil khal
    Commented Mar 24 at 16:21
  • $\begingroup$ Yes, that is correct. $\endgroup$
    – Cameron Williams
    Commented Mar 24 at 16:42

1 Answer 1

Reset to default
0
$\begingroup$

Any reasonable wave can be decomposed into a series of sinusiodal waves. Reasonable means things like having only a finite number of discontinuities.

But only for a linear phenomenon does the behavior of the wave match what you get from adding up behaviors of individual sinusoids.

A soliton is an example that is non linear.

I get different opinions on whether tidal bores are examples of solitons. The most common example seems to be the Severn bore. E.G. Soliton. But according to Chasing the silver dragon: The physics of tidal bores, tidal bores are shock wave.

Nevertheless, there are soliton water waves in shallow channels. The waves interact with the bottom. Larger waves reach deeper and have a stronger bottom interaction, and therefore behave differently, than shorter waves. Simply adding the height of sinusoidal waves as time passes does not give the height of the total wave.

Here are a couple screenshots from Chuu-LianTerng: Solitons in Geometry, starting about 11 minutes in. This is a rather mathematical lecture on solitons with various examples. Initial state:

enter image description here

Later:

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ But my question is this: are there linear or linearized nonlinear systems for which an ordinary sine wave is not a solution? If so, then I'm interested in existing examples of physical systems and the wave process. $\endgroup$
    – shamil khal
    Commented Mar 24 at 19:07

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.