Exact analytical solution for the surface gravity of an oblate spheroid

Question

I would like to know if an exact solution for the surface gravity force components of an oblate spheroid has been published and if not can anyone derive it here?

Assume an ideal rigid oblate sphere of uniform homogenous density, that is not rotating. We can always add in centripetal forces later if required.

The place to start would seem to be this exact general solution for the gravitational potential:

$$P = \frac{3 M}{(2 a e)} \left[B \left(1 - \frac{r^2}{(2 a^2 e^2)} + \frac{z^2}{(a^2 e^2}\right) + \frac{r^2}{(2 a^2 e^2)} \sin(B) \ \cos(B) - \frac{z^2}{(2 a^2 e^2)} \tan(B) \right] \ \ $$

where $$B = \frac 1 2 \arccos \left( \sqrt{\frac{4 a^2 e^2}{r^2} + \left(1+\frac{a^2 e^2 + z^2}{r^2}\right)^2} - \ (a^2 e^2 + z^2)/r^2 \right),$$

$M$ is the mass, $a$ is the semi-major axis of the elliptical cross section of the oblate, "e" is the eccentricity of the oblate, $z$ is the vertical distance from the equatorial plane and $r$ is the horizontal distance from the vertical axis of rotational symmetry, of the point on the surface of the oblate.

This equation for the external potential of an oblate spheroid of homogenous density was derived by Gauss and Dirichlet. See this 2018 paper by Hofmeister et al that was linked to by @ProfRob in a question about the gravity of an oblate sphere in the Astronomy forum.

I do not want solutions that depend on $J_2$ harmonic approximations as these are only generally valid for low eccentricity bodies. I am looking for exact general solutions that can be safely applied to bodies with high eccentricity

Answer 1

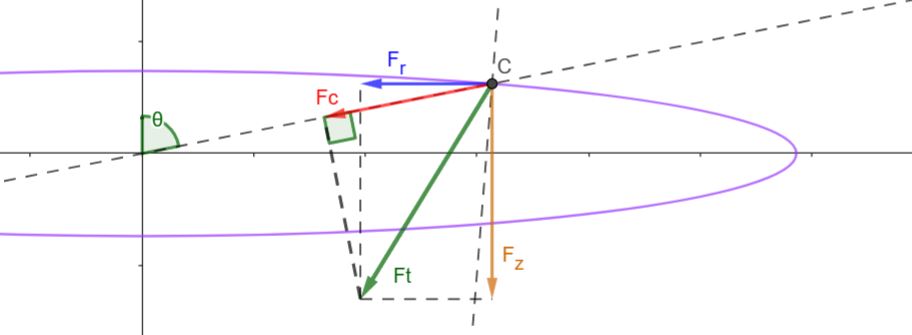
Using Mathematica to differentiate the exterior potential — after first verifying that it satisfies Laplace’s equation — I found that at the surface, where

$$z^2 = (1-e^2)(a^2-r^2),$$

the exact components of the gravitational force simplify to

$$F_r\big|_\text{surface} = -C_r\,\frac{r}{a}\,\frac{GMm}{a^2}$$

$$F_z\big|_\text{surface} = \mp C_z\,\frac{\sqrt{a^2-r^2}}{a}\,\frac{GMm}{a^2},$$

where $C_r$ and $C_z$ are dimensionless constants that depend on the eccentricity $e$ of the oblate spheroid:

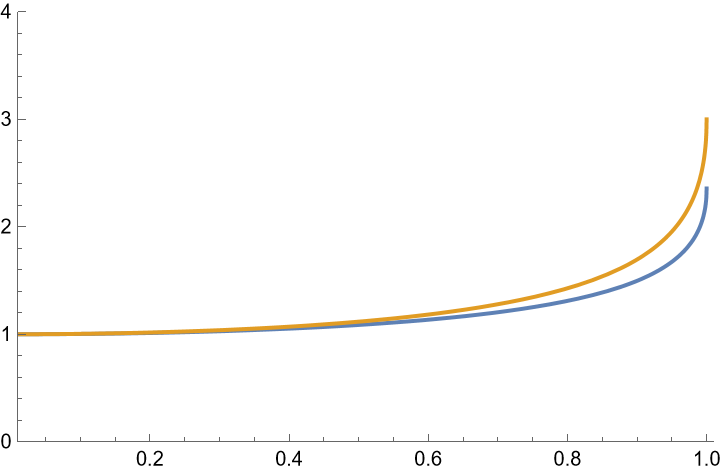
$$C_r = \frac32 \frac{\sin^{-1}e-e\sqrt{1-e^2}}{e^3} = 1 + \frac{3}{10}e^2+O(e^4)$$

$$C_z = 3\,\frac{e-\sqrt{1-e^2}\sin^{-1}e}{e^3} = 1 + \frac25e^2+O(e^4).$$

Note that $r\equiv\sqrt{x^2+y^2}$ is the cylindrical radial coordinate.

In the formula for $F_z$, the negative sign is for the upper half (i.e., $z>0$) of the spheroid and the positive sign is for the lower half.

No approximations were involved in this calculation. The point of the series expansions is to show that the expected result follows in the spherical case when $e=0$.

In the opposite limit $e\to 1$, one has $C_r \to 3\pi/4$ and $C_z \to 3$.

I have no idea whether these results for the surface gravity are previously known, but I'd guess that they have been published somewhere.

The following graph shows the dependence of these constants on the eccentricity. The blue curve is $C_r$ and the gold curve is $C_z$.

I've expressed both $F_r$ and $F_z$ at the surface in terms of the radial coordinate $r$. But one could just as well express them in terms of $z$ (or a polar angle $\theta$).

If one uses the equation for the surface to express $F_z$ there in terms of $z$ instead of $r$, one finds that $F_z$ is simply proportional to $z$, in the same way that $F_r$ is proportional to $r$.

At first I was surprised by the fact that such a complicated external potential gives rise to simple linear relations for the surface force components. However, once I looked at the internal potential, I realized that it's obvious!

As the Hofmeister paper mentions, the internal potential is much simpler: $B$ is just a constant inside! (It's equal to $\sin^{-1}e$.) This means that the internal potential is simply quadratic in both $r$ and $z$, so the internal force components are linear.

The gravitational force does not change discontinuously as one goes from outside to inside. (For proof, walk down to your basement. Do you feel a discontinuity in gravity? A spheroidal shell would cause a discontinuity.) Therefore to compute the surface gravity one can use the much simpler interior potential. This way is so simple that you can do it by hand rather than using a computer algebra system.

As a visualization of an oblate spheroid’s gravity, the following image shows the contour lines of the potential — both interior and exterior — and the vectors for surface gravity, for a spheroid with eccentricity 0.9:

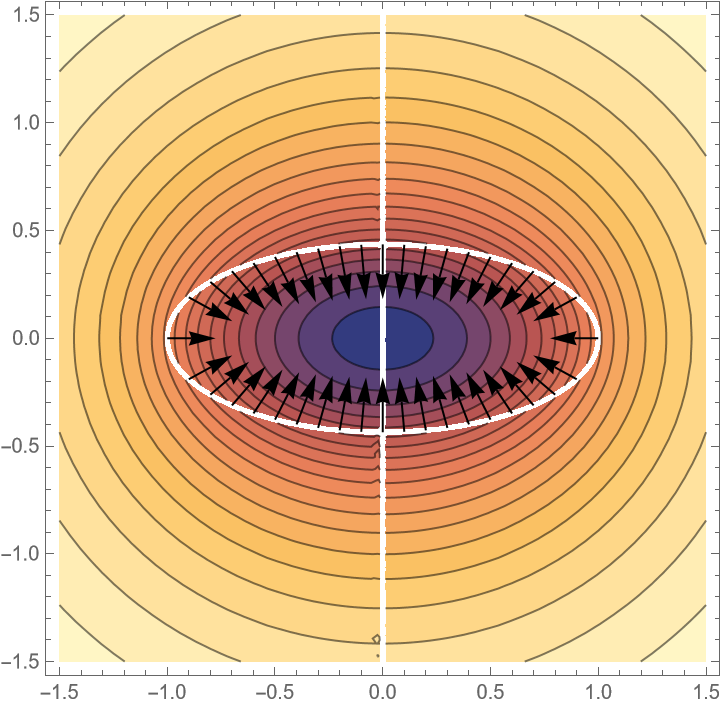
The white ellipse is the surface of the spheroid. The vertical white line is an artifact of not taking sufficient care when evaluating the potential on the $z$-axis.

Note that the force vectors are orthogonal to the equipotential surfaces, not to the surface of the spheroid. And note that in general they don't point toward the center of the spheroid.

Answer 2

The first step is to find the partial derivative with respect to $r$ of the exterior gravitational potential of the oblate, derived by Gauss and Dirichlet.

The next step is to substitute the value of $z$ (The vertical coordinate) as a function of $r$ (the horizontal coordinate).

$$z(r) = \sqrt{(a^2 - a^2 e^2) \cdot (1 - r^2/a^2)}$$

which is readily obtained from the definition of an ellipse. Here $a$ is the usual semi-major axis of the ellipse and $e$ is the eccentricity. $r$ is the horizontal distance parallel to the $x$-axis, from the central rotation axis to the surface point.

A similar procedure to find the partial derivative with respect to $z$ is used to find the vertical component $F_z$ of the force.

The exact result for the horizontal component $F_r$ of the surface gravitational force can be seen in this Mathematica worksheet, but I recommend the simplified equations found by @Ghoster in his answer.

In short $$F_z \propto M \sqrt{a^2-r^2} \quad \text{and} \quad F_r \propto M r.$$

While it may be initially surprising to see that the horizontal component (blue curve below) increases with distance from the centre, it becomes obvious this must be the case, since centrifugal force ($\text{CF}$) also increases linearly with increasing distance from the centre as per $\text{CF} \propto \omega^2 r$ and a fluid oblate rotating body is the result of the equilibrium of those forces.

The resultant total force $F_t$ is easily found using Pythagoras:

$$F_t = \sqrt{F_r^2 +F_z^2}.$$

Below is a plot of $F_r, \ F_z, \ F_t, \ F_c$ and $z$, from $r=0$ to $r=a$.

This plot is colour coded the same as the force vectors in the diagram above it. The magenta curve ($z$) is the elliptical profile of the oblate at $e = 0.99$. The gold curve ($F_z$) is the vertical component of the surface force and drops off to zero going towards the equator. The blue curve is the horizontal component ($F_r$) that points at the vertical $z$-axis. This component steadily increases going from the centre to the equator. The green curve ($F_t$) is the resultant for $F_r$ and $F_z$ and only drops off by about $20\%$ going from the centre out to the equator. The red curve ($F_c$) is the component of the total surface force that points directly at the centre and after an initial drop off starts to increase linearly going outwards.

If we naively assumed the central force was proportional to $\dfrac{G\ M \ m}{R^2}$ (where $R = \sqrt{r^2+z^2}$), then the required balancing centrifugal force would require a velocity of $u = \sqrt{\dfrac{G \ M \ m}{R}}$, while using the central pointing force $F_c$ derived above, the required velocity to produce the required balancing centrifugal force is given by $v = \sqrt{ F_c \ \ R}$ and these two velocity curve profiles are plotted below for comparison:

The classic assumption (blue) for stable orbital velocities, drops off with increasing distance from the centre, while the exact solution presented here, shows the required balancing velocities increase with increasing distance from the centre. Note that this fact cannot eliminate the requirement for invoking dark matter to account for the flat orbital velocity curves that are seen in galaxies, because the flat curves extend far beyond the visible radius of the galaxy (sometimes by orders of magnitude) and these equation only apply to the visible radius. Beyond the visible radius these equations would expect the rotation velocities to drop off with increasing distance from the visible matter of the galaxy if there is no dark matter.

A typical elliptical galaxy with a central bulge could be modelled by adding the above equations for the flattened disk, to the usual $F = \dfrac{G \ M \ m}{r^2}$ to allow for the extra mass of the bulge at the centre modelled as a sphere. .