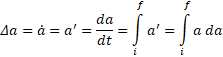Are these all equivalent and is there an extension of this to other notation? Does anyone have a clear and concise chart equating the different notation dialects?
I am also curious if there are more nuances to this or if it can be taken at face value.
The equality you write in your post is most certainly not true. Arguably the only pair for which equality holds is:
$$\dot{a} = \frac{\mathrm{d}a}{\mathrm{d}t}$$
Which is true by definition of the "dot" notation.
The next closest is $a' = \dot{a}$ but in most cases there is a different connotation to these, at least in physics. In physics, the "prime" notation almost always denotes differentiation with respect to a position variable, i.e.
$$a'\equiv{a}'(x)\equiv\frac{\mathrm{d}a}{\mathrm{d}x}$$
The $\Delta$ notation is only tangentially related to differentiation. In particular, you can say that:
$$\frac{\mathrm{d}a}{\mathrm{d}t}=\lim_{\Delta{t}\to{0}}~\frac{\Delta{a}}{\Delta{t}}$$
You might recognize that this is basically just the limit definition of a derivative. A small change in $a$ would be denoted:
$$\Delta{a}=a_{\text{final}}-a_{\text{initial}}=a(t_0+\Delta{t})-a(t_0)$$
Then we can see that the definition of the derivative is:
$$\frac{\mathrm{d}a}{\mathrm{d}t}\equiv\lim_{\Delta{t}\to{0}}~\frac{a(t+\Delta{t})-a(t)}{\Delta{t}}$$
The integrals are where this goes really wrong. In particular $\int_i^fa$ doesn't have obvious meaning or utility since it doesn't have a differential like $\mathrm{d}t$. The final integral:
$$\int_i^f~a~\mathrm{d}a$$
Is, by the fundamental theorem of calculus, equal to the anti-derivative of $a$ with respect to $a$ (i.e., this is asking "what function $A$ is such that if I differentiate it with respect to $a$ as $A'(a)$ returns the function $a$?") evaluated from $a = i$ to $a =f$. So:
$$\int_i^fa~\mathrm{d}a=A(f)-A(i)$$
The anti-derivative function $A$ is itself easy to calculate, it can be checked to be $a^2/2$, because:
$$\frac{d}{da}~(\frac{a^2}{2}) = \frac{2a^{2-1}}{2} = a$$
So finally:
$$\int_i^fa~\mathrm{d}a=\frac{f^2}{2}-\frac{i^2}{2}$$
Hope this answer helps. As far as there being a table or something similar, you could probably find one online. However, as you go forward in physics and math, this sort of thing will become second nature to you. Just stick with it!
In physics the most common notations for differentiation are, $\frac{da}{dt} = \dot{a} = a'$. The notation $\frac{da}{dt}$ is due to Leibniz, the notation $\dot a$ is due to Newton, and the notation $a'$ is due to Lagrange. The dot notation, $\dot a$, almost always refers to a time derivative. The prime notation, $a'$, may refer to a derivative with respect to time or any other variable, typically made clear by the context, or by the authors explicit definition, for example
$$ \frac{da}{dx} = a'.$$
Typically, the quantity $\Delta a$ is not a derivative, but simply refers to the change in a itself,
$$ \Delta a = a_{final} - a_{initial}. $$
Knowing that, you can perhaps recall that $\Delta a$ is related to the definition of the derivative in the following way,
$$ \lim_{{t \to 0}} \frac{{\Delta a}}{{\Delta t}} = \frac{da}{dt} .$$
The fourth notation you've written, $\int_{i}^{f} a'$, is not a derivative and is not equal to the other expressions. It is also meaningless as an integral without the integration variable, and should look something like this,
$$ \int_{i}^{f} a' dt. $$
The fifth notation you've written, $\int_{i}^{f} a da$ is also not a derivative and is also not equal to the other expressions. It is a definite integral which can actually be solved. Perhaps if we wrote x instead of a this would be more clear,
$$\int_{i}^{f} x dx = \frac{x^2}{2} = \left. \frac{x^2}{2} \right|_{i}^{f} = \frac{f^2-i^2}{2}.$$
For a more comprehensive discussion of this topic there is a good article on Wikipedia. However, most of the article is beyond the scope of your question, so I would recommend you only refer to it to read about specific notations you've seen in class or in your textbooks that you're curious about.
Note: There is one additional notation for differentiation that is quite common in physics, however it refers to a related, but different concept called partial differentiation. For completeness it looks like this,
$$ \frac{\partial a}{\partial t} = \text{"partial derivative of } \textit{a} \text{ with respect to } \textit{t} \text{."}$$
