There are some answers here that do a good job of exhibiting the curves that Kerr has pointed out, but I don't think any have outlined his core argument in a faithful manner, and most have missed key observations at the heart of his point, so I feel compelled to chime in here a bit late. While I would say that Kerr's writing in this paper is sometimes overzealous, overly contrarian, and reads a bit like a rant, his central point has much more merit and content than some other answers would lead one to believe.
Kerr's Point
The first thing to say is that Kerr has, of course, not contradicted Penrose's theorem (or variations thereof due to Hawking, etc.), and despite his bombastic language, I don't think he purports to do so. The technical, rigorous statement of the theorem that you'll find in a GR textbook (e.g. Wald, O'Neill) is entirely correct. Moreover, Kerr has not made any earth-shattering remarks; his essential point is something that most dedicated relativists that have studied the singularity theorems have recognized themselves. Kerr has rightly observed, though, that Penrose's theorem is often strongly over-interpreted in the broader physics community.
Penrose's theorem guarantees, under certain hypotheses, that spacetime is null geodesically incomplete. In particular, it states that if a spacetime
(1) satisfies the null energy condition,
(2) is globally hyperbolic with noncompact Cauchy hypersurface, and
(3) contains a trapped surface,
then it contains at least one incomplete null geodesic, a lightlike trajectory that "ends early". This theorem is a beautiful result that made a large impact on the GR community, as it established that incompleteness is not tied to symmetries of the spacetime. Even so, Kerr has rightly pointed out that this result is actually a good deal weaker than it is sometimes given credit for.
The primary point of Kerr's paper is that this theorem has nothing to do with the central ring singularity of his namesake spacetime. The theorem does not tell us that the ring singularity is there: the singularity could, in principle, be excised from the spacetime and replaced with a self-supporting stationary matter distribution without contradicting the theorem in any way. The essential sticking point with the objections raised in some other answers (and this point is not made well by Kerr, if he intended to make it at all), is that the maximally extended version of the Kerr spacetime does not satisfy the theorem's hypotheses: condition (2) fails, as the maximal extension is not globally hyperbolic. This means that Penrose's theorem tells us nothing at all about the maximal extension-- in particular, it does not tell us that the maximal extension is null incomplete.
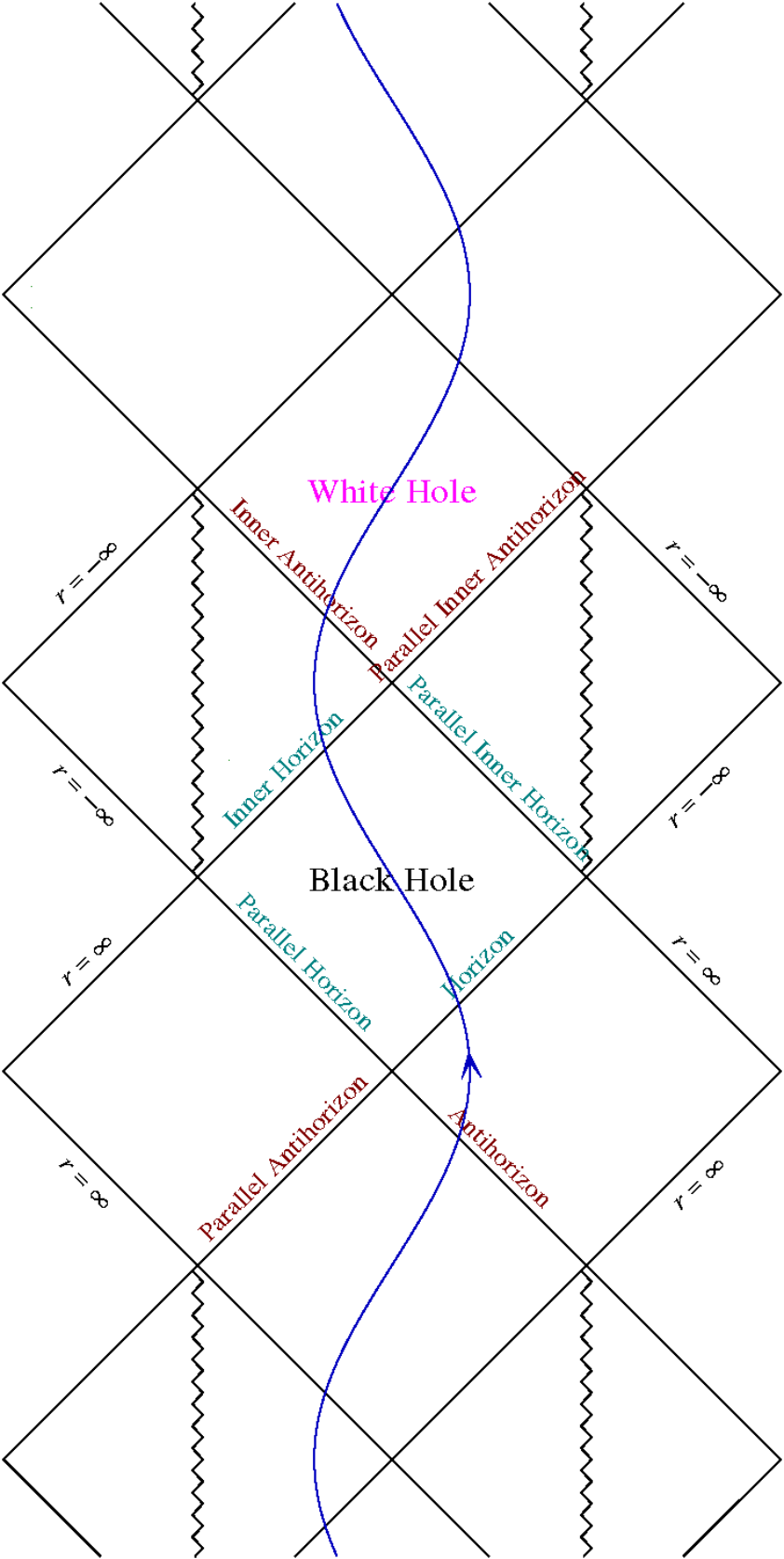
To illustrate, consider the following portion of the Penrose diagram of the $\theta = 0$ cross-section of the maximally extended Kerr spacetime.
$\hspace{3cm}$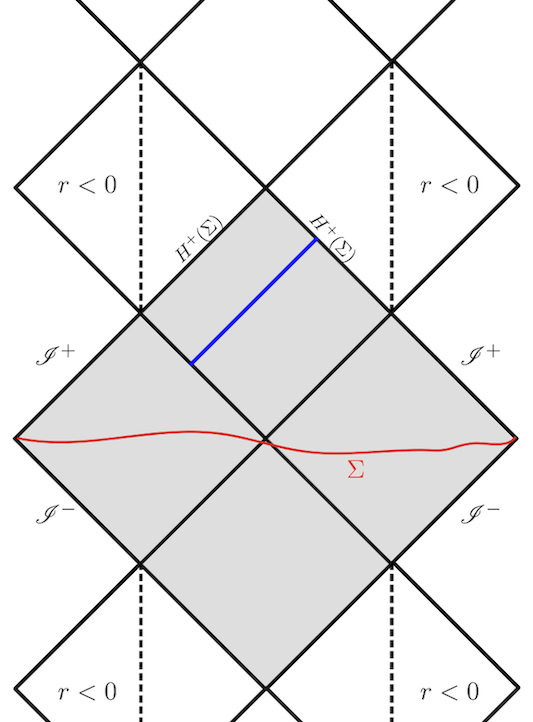
The red hypersurface $\Sigma$ cuts across the exterior asymptotically flat region, and its Cauchy development (the portion of the spacetime determined by $\Sigma$ from the vacuum Einstein equation) is shaded in gray. The inner horizon is the future Cauchy horizon $H^+(\Sigma)$ of $\Sigma$. One of the example curves that Kerr has identified is shown in blue.
The significance of this curve is that, since the full maximal extension is not globally hyperbolic, curves like this are all one can get from Penrose's theorem. Indeed, if one restricts their attention to the gray region, this subset is globally hyperbolic, and the theorem does apply here: it tells us the gray region is null geodesically incomplete as a spacetime in its own right. Of course, that this region is incomplete is nothing new since we already know we can extend the metric beyond it, and the incompleteness of the gray region has nothing to do with any singular behavior that may or may not arise beyond the Cauchy horizon under such an extension. Since they are incomplete in the gray region, Kerr's curves provide explicit examples of the null geodesics guaranteed by the theorem. These curves, however, do not demonstrate any kind of singular behavior-- that we know they can be extended in a larger spacetime means there's nothing singular about them. All curvature scalars are finite, etc. All Penrose's theorem tells us, then, is that null curves like Kerr's (which are decidedly nonsingular) exist in the gray region, and they are incomplete as geodesics within this globally hyperbolic set. As soon as you extend the spacetime beyond this subset by any amount whatsoever (perhaps to try to include the ring singularity), then Penrose's theorem is simply inapplicable, telling us nothing about the extension.
The upshot, then, is that Penrose's theorem does not offer any definitive proof that the interior of a stationary, axially symmetric black hole must be singular-- the incomplete null geodesics it can give us are only incomplete as geodesics restricted to the globally hyperbolic region, and this incompleteness has nothing to do with the ring singularity that may exist beyond the Cauchy horizon. While Kerr has not explicitly provided an alternative extension beyond the Cauchy horizon that is nonsingular, there is no solid reason to believe one doesn't exist. Note that the possibility of replacing the singularity here does not at all necessitate one's going so far as to model the dynamical collapse of a rotating star; it may well be the case that the ring singularity can be replaced by a stationary matter distribution beyond the Cauchy horizon while leaving the gray region entirely unchanged.
The SCC Caveat
A significant caveat to the above discussion is that Cauchy horizons are generally thought to be unstable; this is physically expected due to the infinite blueshift of signals from matter in the exterior that an observer would experience upon crossing $H^+(\Sigma)$. This hypothesis is formalized in the (unproven) Strong Cosmic Censorship Conjecture, which states that the Cauchy development of generic physical initial data (e.g. data specified on $\Sigma$) will be inextendible to a larger (physically meaningful) spacetime. If one accepts this as true, in our diagram it would mean that arbitrarily small perturbations to Kerr initial data on $\Sigma$ would essentially result in the full maximal extension collapsing to just the gray region. In this case, any incomplete curves like Kerr's examples actually would terminate in an inextendible manner, a scenario many relativists would readily call singular.
Even if one accepts Strong Cosmic Censorship, however, Penrose's theorem is still quite limited. It does not ensure that its incomplete null curves experience curvature divergence, and it still doesn't ensure that any massive matter, which follows timelike curves, collapses along singular trajectories.