Why do we need the resonance condition in a cyclotron?

Question

I am currently reading Physics by Halliday, Resnick, and Krane.

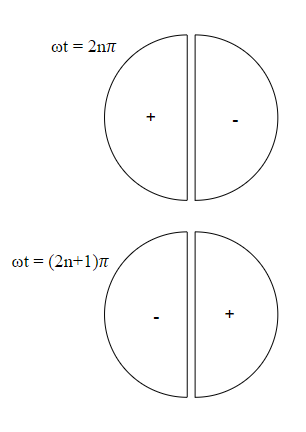
In Chapter 22 about elementary magnetism, there is a discussion about cyclotrons. In particular, it mentions the importance of the resonance condition, where the oscillator must be tuned to the cyclotron frequency $f = \frac{p}{|q|B}$.

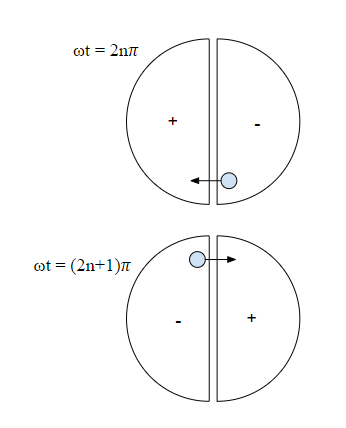
The frequency of the electric oscillator must be adjusted to be equal to the cyclotron frequency (determined by the magnetic field and the charge and mass of the particle to be accelerated according to Eq. 32-12); this equality of frequencies is called the resonance condition. If the resonance condition is satisfied, particles continue to accelerate in the gap and “coast” around the semicircles, gaining a small increment of energy in each circuit, until they are deflected out of the accelerator.

Why do we need this resonance condition in the first place? Even if they were slightly out of step, would the electric field in the gap between the dees not accelerate the particle and give it enough energy to eventually get deflected anyway?

I am trying to understand what would happen if this condition were removed.

Answer 1

In a cyclotron there is an alternating voltage $V(t) = V_0 \sin(\omega t)$ applied between the Ds so the polarity looks like this:

If we are accelerating (for example) electrons then we need the electron to be in the correct position as the voltage alternates:

So the time taken for the electron to do one complete circuit must be the same as the time taken for the applied alternating voltage to complete one cycle. This is the resonance condition referred to in the book.