Linear version of Faraday Paradox: different results in different inertial frames [closed]

Question

Setup:

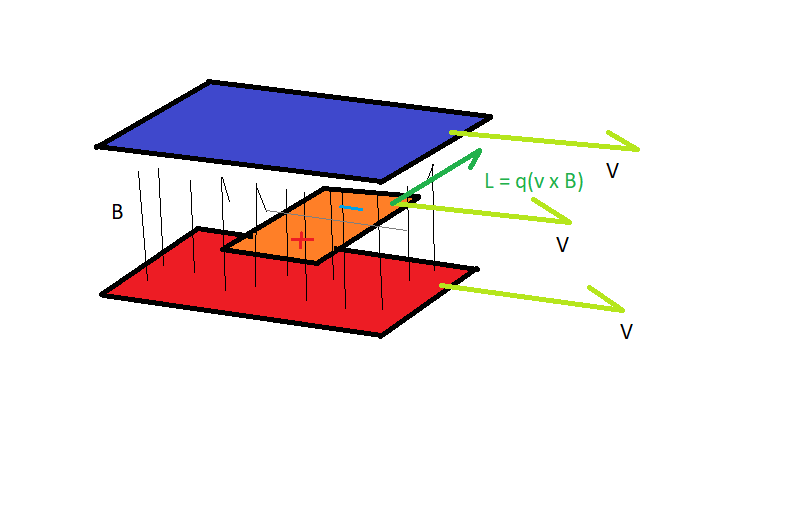
Consider the following setup:

A copper plate is fixed in a uniform constant paralel magnetic field. The whole setup is moved to the right with the uniform velocity $\vec{v}$. You may assume that the magnet is slightly larger that the copper plate, but bounded nevertheless.

Expected results

Similar to the Faraday Unipolar Generator, considering the magnetic field fixed, a Lorenz force is expected as shown. This will "push" the electrons in the conduction band of copper to the sides as shown. Some additional reason for thinkin this way (although I can see the theoretical expectations to be different) is:

In the Unipolar Generator, even if the field is rotated with the disc, a voltage is measured. If we replace the "infinite" disc, with an "infinite"rod, then isolate a small portion at the tip, that would be the device proposed here.

Question

Is this correct? Has this experiment been done?

Edit:

I managed to find a paper discussing some consequences of the above: the possibility to differentiate between inertial frames. Of course, if the above experiment is as thought, one can easily (by measuring the voltage) observe if the frame is moving or not.

Answer 1

There is no net force on the charged particles and no charge separation. If there were then you would have a way of invalidating Special Relativity because you would have a way of defining an absolute velocity for an inertial frame.

If you move your apparatus in that way, then what is a uniform magnetic field in the frame of reference of the copper plate becomes both a magnetic and an electric field in the "laboratory" frame of reference. e.g. See here.

The observed electric field would be $$\vec{E}' = \gamma \vec{v} \times \vec{B}\ ,$$ where $\gamma$ is the usual Lorentz factor.

The observed magnetic field in the laboratory frame would be $$ \vec{B}' = \gamma \vec{B}\ . $$

In the rest frame of the plate, the net force on a charged particle is zero, since the particle would be at rest with respect to the uniform B-field and $\vec{E}=0$.

In the laboratory frame, the force is the sum of that due to $\vec{E}'$ and that due to the motion of the charged particles, moving at $-\vec{v}$ with respect to $\vec{B}'$. Thus $$ \vec{F}' = q(\vec{E}' + (-\vec{v})\times \vec{B'}) = q( \gamma \vec{v} \times \vec{B} - \gamma \vec{v}\times \vec{B}) = 0\ . $$

There would be no separation of the charged particles in either frame of reference.

The "Faraday paradox", where a Lorentz force appears to be induced as if the magnetic field were stationary with respect to a conductor, even when the magnet is moved with the conductor, appears to be confined to rotational motion (e.g., Baumgartel & Maher 2022). It is discussed extensively there, where they point out that a similar phenomenon is not observed for rectilinear motion (the experiment has been done, e.g. Muller 2014). This is fortunate, otherwise Special Relativity would have to be abandoned, since your experiment would offer a way of determining an absolute velocity. The analysis above cannot be applied to rotating frames since they are not inertial.