For a conductor in an external field, I would like to know whether the electron clouds of each atom, just shift slightly (resulting in positive charges just on one edge, and negative charges just on the other with all internal area remaining neutral).
Or, whether the electrons fully dissociate, leaving positive ions throughout the conductor volume but with all electrons on one side.
If this second case, then how would the electric field work out as zero inside?
For a conductor in an external field I would like to know whether the electron clouds of each atom just shift slightly (resulting in positive charges just on one edge, and negative charges just on the other with all internal area remaining neutral) or whether the electrons fully dissociate,
They shift slightly.
But you should realize that the outer "shell" of electrons in a metallic conductor actually form a "band" of states that are already dissociated from any individual atomic nucleus. This happens under normal circumstances and has nothing to do with applied external fields.
That means we can't really talk about the individual atoms within the metal being neutral or ionized, only about the object as a whole being neutral or charged.
This isn't an easy concept to explain or grasp. A college course in solid state physics will spend many weeks covering why this is.
resulting in positive charges just on one edge, and negative charges just on the other with all internal area remaining neutral
Yes, this is the result of an external field applied to an isolated metallic object. But you do seem to have some misconceptions about the intermediate steps.
The density of free electrons in a metal is enormous compared to the charge imbalance induced by any field that won't destroy your laboratory. So, the "sea" of electrons in the metal is almost perfectly unaffected by applied fields.
On the other hand, in a semiconductor, the densities of free carriers (electrons and holes) are orders of magnitude smaller than in a metal. It is thus possible for electric fields to sweep all of the carriers away, producing "depletion regions". The semiconductor switches in your computer's logic work by controlling where the depletion regions are.
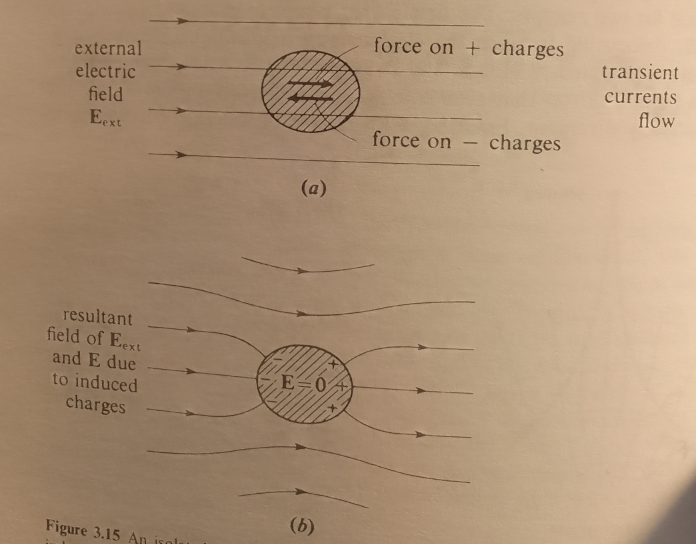
The electrons in a conductor do not fully dissociate and produce positive ions throughout the conductor's volume in response to an external electric field.
Instead, they experience a force due to the field and shift slightly within the lattice. This shift results in a slight separation of positive and negative charges within the conductor, creating an electric potential difference. However, the electrons remain within the conductor, and the material as a whole remains electrically neutral.
So, the charges redistribute in a way that neutralizes the external field within the conductor, resulting in a net electric field of zero inside the conductor.
From "Electricity and Magnetism" by Duffin 1980, Section 3.8:
"[For a conductor isolated in an external electric field (see figure below)], as soon as E is established, one part of the conductor is at a higher potential than another and any free positive charges will move in the direction of E (and any negative charges in the opposite direction). Whatever the sign of the moving charges, the result is the same: charges reach the surface of the conductor and can go no further. They collect and produce a field within the conductor which opposes the applied field. This process continues until within the material, there is no resultant field. Static condition will then again prevail."
Basic law of Gauss, Maxwell: The area density of charge in $As/m^2$ at the plane surface of a metallic conductor is the definition of the field D. The Maxell equation $\text{div} D (A s / m^3) = \rho (A s /m^3)$ degenerates to $D(x>0) - D(x<0) = \sigma(x=0)$, the surface density.
Division by $\varepsilon_0$ yields the electric force field $E (W s/(A s m = V/m)) =\frac{1}{\varepsilon_0 } \ D( A s/m^2)$
It follows, by the thermodynamic basic laws of minimizing field energy, external plus the thin electron surface layer, that exactly as many electrons needed to shield the inner space of the conductor completely from the external field move to surface states. The density of the surface states falls off again so slow, that the always positive quantum concentration energy measured by $|\partial_x \psi|^2 dx$ does not grow to large.
An internal field cannot live inside a conductor, it decays by its induced a current $j=\sigma E $, that rerdiributes charges until $E=0$.
