Is it possible, and if so how should we remodel thought experiments in classical SR in the context of the Scharnhorst Effect?

Question

I was thinking of the following thought experiment which is related to the ideas user David Elm asked here

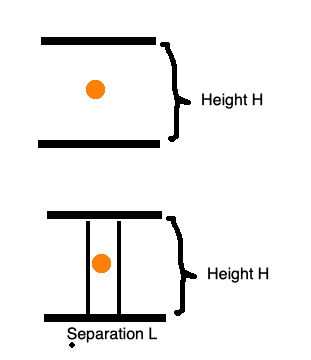
Suppose you have a pair of photon mirror clocks as is often used in SR, where one of the clocks is the usual configuration height separated by a distance $H$, and the other clock has its mirrors in the same configuration but also has two parallel conducting plates with a very small separation $L$ apart. This is sketched below:

So the first clock would tick according every $\frac{2H}{c_0}$ seconds (where $c_0$ is the speed of light in vaccum). And the second clock would tick at a rate of $ \frac{2H}{\left(1 + \frac{11e^4 }{2^6 (45)^2 (m_e L)^4} \right)c_0 } $ according to the formula of Scharnhorst which is reproduced on page 19 of The Scharnhorst Effect: Superluminality and Causality in Effective Field Theories by Sybil Gertrude de Clark here.

So now consider some observer moving in at a velocity $v$ parallel to the motion of the two photons.

So classical SR tells us the speed of light in the vacuum is the same for all observers, so no matter how fast the observer moves the photon in the first mirror configuration should always be seen moving at the same speed either away or towards our observer.

Now classical SR also tells us the same thing should be true for the second mirror as well (after all it is a vacuum between those two conducting plates).

Now according to the observer the second mirror's photon CLEARLY moves faster than the first's if we assume the validity of the Scharnhorst effect. And no matter how fast the observer moves in the direction parallel to both photons, BOTH of the configurations should appear to continue moving at the same velocity.

Is this the right way to think about this? The problem is just taken at face value we can consider some of Einstein's old thought experiments and then derive two different time dilation formulas (one each for each of the clocks), and similarly two different length contraction formulas etc etc... which (I think?) cannot both be simultaneously valid.

So how should we correctly model what our observer sees?

Answer 1

The second postulate of SR is that there is one particular speed that is invariant for all observers in inertial frames. This happens to correspond with the classical speed of light in vacuum (which you denoted by $c_0$). Something moving at a speed other than $c_0$ will not be observed to have the same speed by all inertial observers: for example, a light beam in a medium, or under the hypothetical influence of the Scharnhorst effect.

Answer 2

This is probably getting dangerously close to philosophy or pseudoscience (whichever is considered worse) but here are some thoughts.

1. If we assume the Scharnhorst effect is real and experimentally realizable AND
2. We insist that speed of light in the vacuum has to be the same for all observers

Then

The only way the apparent speed of light could be faster between the plates is if either the distances parallel to the plates or flow of time between the plates was altered.

Saying the same with more words: That is a ruler outside the region between the plates and the same ruler inside the region would report different lengths. In other words the ruler between the plates would report a smaller distance than the ruler outside the plates. So while the speed of light is not altered, external observers would claim that “light seems to travel faster between the plates the way Scharnhorst predicts” while internal observers would say “light runs at the normal speed here, those external folks rulers are messed up!”

OR:

A clock in the region outside the plates would be reported as running slower than the identical clock placed between the plates. This is already not an alien concept given the notion of gravitational time dilation being less in regions of less gravitational potential. Instead we would have a concept of electrodynamic time dilation in vacua that have less virtual photon activity.

OR: some combination of the two effects.

Answer 3

The clocks in this section are ill posed, the scharnhorst effect's relative speed up of light only applies in the direction NORMAL to the two conducting plates. There are still versions of this question that can be asked but its different than the exact version here. See page 2038 of: "1993 J. Phys. A: Math. Gen. 26 2037" QED between parallel mirrors: light signals faster than c, or amplified by the vacuum by Barton and Scharnhorst.

Now here are some observations where things get strange. In SR there is an unambiguous notion of "photon clock". Two perfect mirrors spaced some fixed distance $d$ apart with a single particle of light bouncing between them. If you reduce the distance $d$ by $2$ then the light bounces twice as frequently (as it maintains the same speed), so that if you measure time elapsed by measuring distance travelled by that bouncing photon, you always get an unambiguous measure of proper time, regardless of how far away $d$ the mirrors are arranged.

What QED basically proposes via the Scharnhorst effect is that as the mirrors get closer and closer the rate of bouncing between the photons accelerates at a rate coupled to $d^4$. So photon clocks cannot be used to measure proper time. To pick a particular photon clock distance $d$ and say "this is how we measure time" is an extremely arbitrary choice and the rate of oscillation couples in a non-linear fashion to the separation $d$ so the way proper time is measured has to be different than this.

The next important thing to point out is that if we have our photon clocks with photons bouncing and all the mirrors are parallel to each other, we do still have the question of "how do the apparent speeds of all of these apparent clocks change as an observer accelerates and approaches $c$". That's still an interesting and strange question but i'll ask it at a later time.