Electric field of a conducting plane and a charge?

Question

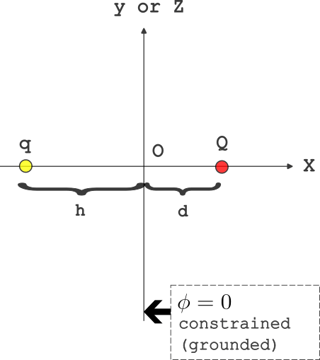
I have a conducting plane positioned in x=0 that infinitely extends in y and z. The plane is earthed, so its potential is 0, and at a distance d>0 on the x axis I have a charge Q. I'm trying to understand what would happen if I put a charge on the other side of the plane (x<0). Since the plane is infinite, it should act like an electrostatic shield, right? So it's like all the space in x<0 is inside the shield, therefore the potential should remain constantly 0. Does this mean that the electric field is 0 too, so a charge put there would stay still? My other idea is that the plane "hides" the charge Q, so it's like we don't have it, and we have to consider the elecrtric field generated by the plane only, but I guess it's field is not simply $$E=\frac{\sigma}{\epsilon_0}$$ since the plane just has an inducted charge which is not uniformely distributed. Can these two visions coexist, or is it just one of them that is correct? If so, why is the other one incorrect? Or are they both wrong?

Answer 1

This text may not contain all the answers you are looking for. Sorry and be patient.

The problem illustration is something below.

The center plate thickness is assumed to be 0 and grounded.

The right side, i.e., the region such that $x>0$, the solution is of the form: \begin{equation*} \phi_R(x,y,z)=\frac{Q}{4\pi\epsilon_0}\left(\frac{1}{\sqrt{(x-d)^2+y^2+z^2}}-\frac{1}{\sqrt{(x+d)^2+y^2+z^2}}\right)1(x),\tag{1} \end{equation*} here the Heaviside step function, \begin{equation} 1(x)= \begin{cases} 0 \text{ for }x<0 \\ 1 \text{ for }0\leq x, \end{cases} \tag{2} \end{equation} is used. The solution is derived from the image method. This solution satisfies $\phi_R=0$ on the plate. Similarly, the left side solution is given as, \begin{align*} &\phi_L(x,y,z)=\\ &\frac{q}{4\pi\epsilon_0}\left(-\frac{1}{\sqrt{(x-h)^2+y^2+z^2}}+\frac{1}{\sqrt{(x+h)^2+y^2+z^2}}\right)1(-x).\tag{3} \end{align*} The entire solution is the sum of two terms: \begin{equation*} \phi(x,y,z)=\phi_L(x,y,z)+\phi_R(x,y,z).\tag{4} \end{equation*} The solution is computed and added independently on the left and right sides. Therefore, the right area does not affect the left area. In other words, it is electrically shielded. It should be noted, regarding electrical shielding, it depends on whether the center conductor is grounded or isolated. If it is isolated and the conductor is not supplemented with electrical charge from the outside (ground), for example, under the condition that isolated and the total electrical charge is zero, then no electrical shielding occurs. An example of no schielding is in here.

For the electric field corresponding to the solution (4), there arises an additional term. For example, $E_x^R$, the x-component of the electric field on the right side, becomes \begin{align*} &\frac{4\pi\epsilon_0}{Q}E_x^R=\frac{4\pi\epsilon_0}{Q}\left[-\frac{\partial \phi_R(x,y,z)}{\partial x}\right]\\ &=-\left(\frac{\partial}{\partial x}\frac{1}{\sqrt{(x-d)^2+y^2+z^2}}-\frac{\partial}{\partial x}\frac{1}{\sqrt{(x+d)^2+y^2+z^2}}\right)1(x) \\ &-\left(\frac{1}{\sqrt{(x-d)^2+y^2+z^2}}-\frac{1}{\sqrt{(x+d)^2+y^2+z^2}}\right)\delta(x). \tag{5} \end{align*} The second term involving Dirac's delta function could be the electric dipole moment distribution on the central conductor. Please do not ask about this delta function term because my brain is empty.