Drawing Electric field lines

Question

Suppose there is a medium filled by a charge with the volume density $\rho = \frac{\alpha}{r}$ where $\alpha$ is a positive constant and r is the distance from origin.

Now here if we calculate electric field at any point in the space then it will come out to be constant and magnitude will be $\ 2Kπ\alpha$ and direction will be along the radial vector.

Now if we want to draw the electric field lines in the medium then I can't follow one of the rules which is:

The electric field lines density crossing any particular area represents magnitude of field; means mores density means more field.

I would like to know why is that so.

Answer 1

Now if we want to draw the electric field lines in the medium then I can't follow one of the rules which is:

The electric field lines density crossing any particular area represents magnitude of field; means mores density means more field.

Actually you can draw the field lines and follow this rule. There are charges in some distance around the origin. And hence there are field lines beginning at these charges. Just remember, positive charges, if present, are places where electric field lines begin (and negative charges, if present, are places where they end).

Answer 2

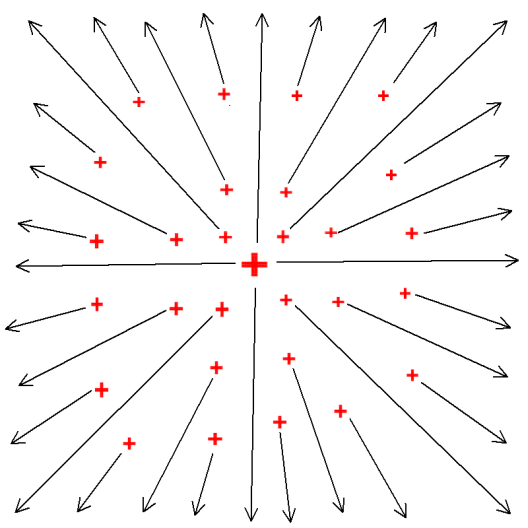
Lines of electric field are used now as a visual aid but in the past, they were "counted" and electric flux was a measure of the number of lines passing through a given area.
In your example, electric field lines start on a positive charge and move radially outwards from the centre of the charge distribution (and end up at infinity).
Since the radial electric field has a constant magnitude the electric flux (number of field lines) through a given area is proportional to the area.
Let me try and illustrate what happens visually but the problems are that your example requires one to consider three dimensional space and I am not so good at drawing diagrams.

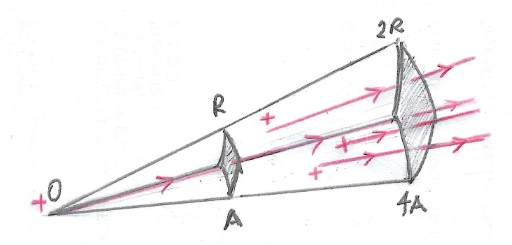
What my diagram is trying to show is two areas which are part of spheres with radii $R$ and $2R$ and hence areas $A$ and $4A$.

If one field line, starting somewhere in the sphere of charge of radius $R$ passes through area $A$ then four field lines must pass through the area which is four times larger, $4A$.
Thus the field line which passed through area $A$ would continue on and pass through area $4A$ and then three extra field lines, starting on positive charges between the two areas, would pass through area $4A$.

In terms of Gauss's law, there is three times more charge between $R$ and $2R$ as there is between $0$ and $R$.
Hence field lines equivalent to the four units worth of charge pass through area $4A$ whereas field lines equivalent to one unit worth of charge pass through area $A$.

Perhaps you can get further visualization by imagining that starting with the one field line passing through area $A$, two field lines will pass through area $2A$ at $\sqrt 2R$, and three field lines will pass through area $3A$ at $\sqrt 3R$.
Then you might start with two field lines passing through $A$ and subdivide the volume between $R$ and $2R$ appropriately as well as the volume between $0$ and $R$?