1
$\begingroup$

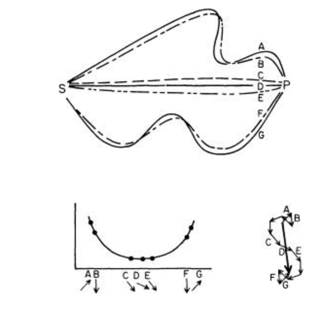
In QED we look at all possible path a photon could go from S to P, and I understand the most significant contributions to the final arrow are the few near straight paths connecting S and P while other crazy curved paths cancel out. But does the initial direction the photon is emitted from the source S come into play at all? I mean, if I point a flash light (that's capable of emitting a single photon) 180 deg away from detector P, surely it's not likely to reach the detector, but I can't see how this is used in the "all path" calculation. If the photon indeed takes all path wouldn't the most probably path the one where photon upon leaving source immediately turns around 180 deg and then goes more or less straight to P?

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ I think this is mostly a misinterpretation of the path integral formalism. The path integral doesn't evaluate all the possible classical paths of "a photon". It integrates the complex exponential of the classical action over all possible paths to arrive at the propagator, i.e. it is a quantization procedure. Photons don't have paths. They are not objects. The probability to find a photon simply tells us where an irreversible interaction of the field with an external system might deposit energy in that system. $\endgroup$
    – FlatterMann
    Commented Jun 6, 2023 at 23:57
  • 1
    $\begingroup$ The OP did not make a mistake of asserting that the path integral is evaluating over classical paths. Why do you feel the need to plaster your mistaken ideas over all questions? $\endgroup$
    – naturallyInconsistent
    Commented Jun 7, 2023 at 0:06
  • $\begingroup$ "In QED we look at all possible path a photon could go"... Do we? We simply give a new quantization procedure for the electromagnetic field that takes a classical Lagrangian to a quantum mechanical propagator. In reality photons don't go anywhere. They are phenomenological endpoints. $\endgroup$
    – FlatterMann
    Commented Jun 7, 2023 at 0:13

3 Answers 3

Reset to default
0
$\begingroup$

But does the initial direction the photon is emitted from the source S come into play at all?

In the Newtonian (and Hamiltonian) framework, you are very familiar with specifying the initial position and momentum, and then evolving the system forward in time.

Feynman reported that when you are doing Lagrangian mechanics, the integral of the action seems to be needing a specification of the boundary conditions at both ends. You can specify both positions, or both momenta, or any mixture, but you cannot choose to give the position and momentum at one end, and expect to get the result correct. You have to specify one quantity at each end, and then after solving for the trajectory, invert the relations to match the other condition you wish to have, in order to get the answer you want.

surely it's not likely to reach the detector, but I can't see how this is used in the "all path" calculation.

Yes, it is not likely, but you will only get this result after you first derive the semi-classical trajectories for the general case, and then integrate over the wavefront that is leaving the torch. You must have enough of a wave to destructively interfere elsewhere, so that then you will have the usual beam properties that you come to expect. The intermediate work before that will not necessarily have the expected properties.

Improve this answer
$\endgroup$
2
  • $\begingroup$ A photon follows a singular straight path, capable of diffracting or scattering, but reversing its direction by 180 degrees requires an extraordinary occurrence. The original post clearly focuses on this specific direction, yet your second paragraph either disregards or is ignorant to the physical implications sought by the original poster. $\endgroup$
    – Bill Alsept
    Commented Jun 7, 2023 at 3:45
  • $\begingroup$ No, path integration takes non-straight paths. I specifically treated the reason why it does not reverse direction. $\endgroup$
    – naturallyInconsistent
    Commented Jun 7, 2023 at 6:27
0
$\begingroup$

You are talking about Feynman's path integral (PI). Usually this refers to 2 points were there is a straight line path between the source and detector. Once we consider the straight line he then adds additional paths for the purpose of computing "interference" ... i.e. is a photon probable to travel here or not.

It is easy to compute the PI with a computer ... for example in the double slit we could calculate a thousand or more rays starting at the source passing at 500 points spread evenly over slit 1 and 500 over slit 2. Once the path length is computed the phase at the screen is calculated .... by summing/squaring amplitude of 1000 paths from source to a point on the screen a relative probability is available.

In the DSE bright areas have all the photons (high probability), dark areas none (low probability). One does not need to compute a billion paths or any curved paths .... enough straight line paths are sufficient as long as some symmetry is kept. In the DSE all the most probable paths tend to be ones where the primary path has a path that is an integer multiple of the wavelength ... one can say light/photons like to travel full wavelengths!

Improve this answer
$\endgroup$
3
  • $\begingroup$ Now you are adding insult to injury. The path integral is an infinite dimensional integral that does not even converge in its naive form. The full single-quantum propagator K would compute all of the near field of the doble-slit in addition to the far field in Frauenhofer approximation. That is not even an easy computation in Maxwell, let alone quantum mechanically. Moreover, the prescription of the path integral doesn't say that you can arbitrarily chose your integration domain to reflect certain classical boundary conditions. Matter in QED means that we have to throw fermions in... $\endgroup$
    – FlatterMann
    Commented Jun 7, 2023 at 0:20
  • $\begingroup$ @FlatterMann you're implying the PI is unusable? The full PI integral also includes an additional probability interaction term .... likely this is why geometric approximations work. (Note classicalism is not the same as geometric). $\endgroup$
    – PhysicsDave
    Commented Jun 7, 2023 at 23:27
  • $\begingroup$ I am implying that you should take a good look at the definition of a path integral. $\endgroup$
    – FlatterMann
    Commented Jun 8, 2023 at 5:04
-2
$\begingroup$

A photon traveling from S to P moves along a straight path, except when its trajectory is affected by diffraction, scattering, or gravity. When discussing reflection, it's important to note that each instance involves a new photon.

Improve this answer
$\endgroup$
2
  • $\begingroup$ This is not even attempting to make sense in the context of the question. The OP even included pictures obviously signifying the Feynman path integral is being meant, and so it is inexcusable that your answer seems to be ignorant of it. $\endgroup$
    – naturallyInconsistent
    Commented Jun 7, 2023 at 0:03
  • $\begingroup$ Clearly, the topic revolves around Feynman's path integral. I was answering the title question regarding the direction of a photon's emission and addressing the OPs final sentence, to point out that individual photons do not take all conceivable paths. The arrows in the discussion symbolize the Straight paths taken by individual photons, and it was the overall trajectory of light that appears to take a straight path. Your enthusiasm to go all quantum may have caused you to overlook the main point raised by the OP. $\endgroup$
    – Bill Alsept
    Commented Jun 7, 2023 at 4:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.