2
$\begingroup$

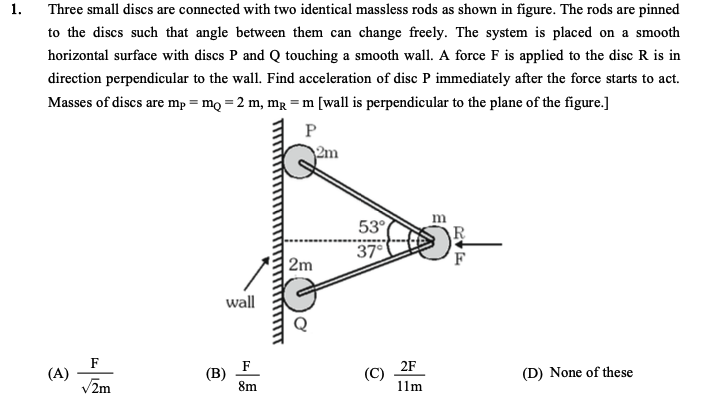
enter image description here

I am trying to obtain the equations of motion using the euler-lagrange equation. First, let $x$ be the distance of disc R from the wall. Let $y_p$ and $y_q$ be the distance of disc P and disc Q from the dotted line in the diagram. Since F is a conservative force, the equation of potential energy will be $V = Fx$. Then for kinetic energy, $T = \frac{1}{2}m\dot{x}^2 + \frac{1}{2}2m(\dot{y_p}^2 + \dot{y_q}^2)$.

After that, I applied the euler-lagrange equation on $L = T - V$ for all three coordinates and I obtained the following equations,

$m\ddot{x} = -F$

$\ddot{y_p} = 0$

$\ddot{y_q} = 0$

But the answer for the question is not $0$, I am quite new to using lagrangian mechanics so I need a detailed explanation of how to solve these kind of problems.

Improve this question
$\endgroup$
3
  • $\begingroup$ How many degrees of freedom are in this problem? $\endgroup$
    – Mario Figueroa
    Commented May 30, 2023 at 9:51
  • $\begingroup$ You have to account for the constraints betweencoordinates. The rods are rigid so $x^2 + y_j^2 = d^2$ for $j=p,q$, where $d$ is the length of the rod. What does this mean to the number of degrees of freedom for this system? $\endgroup$
    – agaminon
    Commented May 30, 2023 at 11:43
  • $\begingroup$ Just to expand on @agaminon, you need to incorporate holonomic constraints as explained here. $\endgroup$
    – J.G.
    Commented May 30, 2023 at 12:30

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.