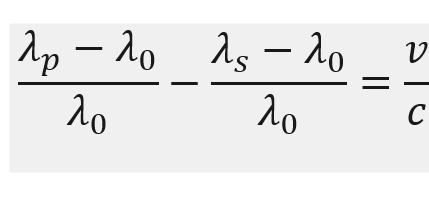Methods for calculating the orbital speed of a star in a binary system

Question

As I understand it, to calculate the speed of rotation of a star in a binary system that is receeding, that is in the same plane as us (inclined at 0 degrees) the method is use the Doppler formula: Δλ/λ=v/c

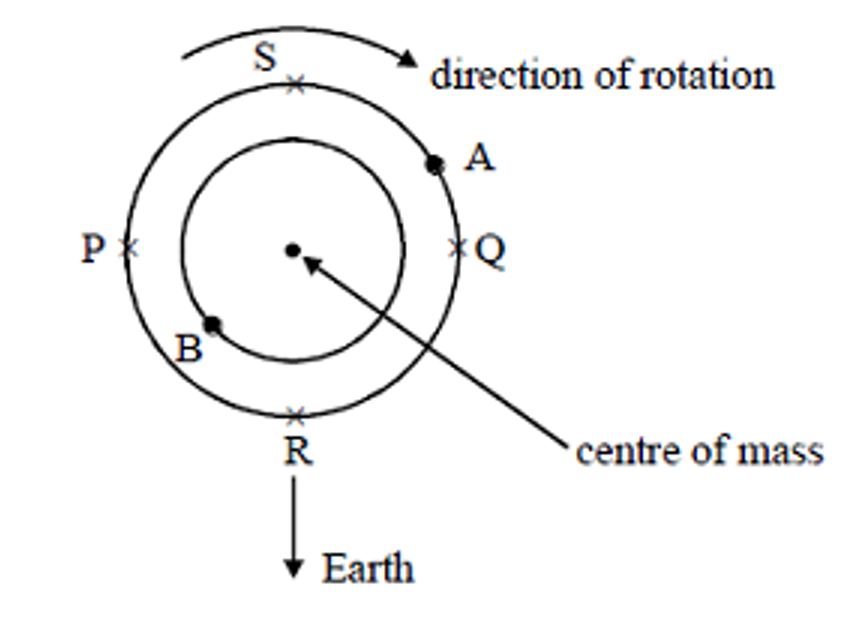
In the diagram below A and B are stars. If we wanted to calculate star A's orbital speed, Δλ would be the difference in a spectral line's wavelength at P subtracted from that same spectral line's wavelength at S, this would then be divided by the wavelength of the spectral line at S. Using this we can calculate the orbital speed using the formula above.

However, what I do not understand is why I could not find the orbital speed of star A at point P by:

where λp = wavelength of spectral line at p, λs = wavelength of same spectral line at s and λ0 = spectral line in the lab frame of reference

To me the first term in the equation gives me the orbital speed + recessional speed, the second term is the recessional speed. Subtracting the two should therefore give me the orbital speed. This is not the case as the maths is not the same as the correct method above but I cannot work out what I am missing. Any help would be much appreciated.

Answer 1

Your first method is incorrect; or at least is only an approximation that assumes $\lambda_p \simeq \lambda_0$. In practice that won't be the case because the centre of mass will have some line-of-sight velocity with respect to the observer. The second method takes that into account - as you explain yourself.

$$ \frac{v}{c} = \frac{v_p - v_s}{c} = \frac{\lambda_p - \lambda_0}{\lambda_0} - \frac{\lambda_s - \lambda_0}{\lambda_0}\ .$$

Your first method is trying to use $v$ as the Doppler shift due to the relative velocity between P and S, which is ok, but is using definitions of the wavelengths as seen by an observer on Earth rather than at P.