Consider a rigid body $\mathcal{B}$ modeled by a system of $n$ point masses $B_1,B_2,\dots, B_n$ constrained to keep constant distance from each other. I wonder how it is possible to mathematically explain why, for any set of external forces applied on this system, there is always some configuration of the internal forces which maintains its rigidity.
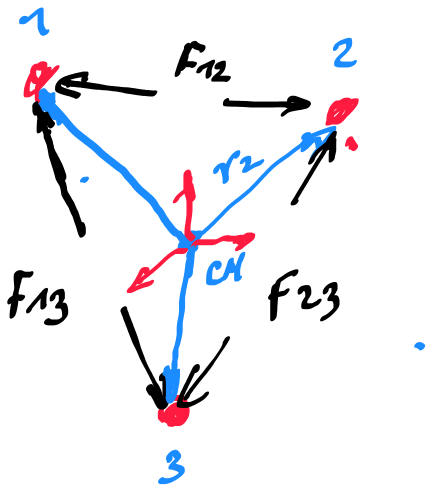
More explicitly, suppose that on each mass point $B_i$, an external force $\mathbf{F_i}$ is applied. For simplicity, suppose that the net force is $0$ and that the net torque is $0$: $$\sum_{i=1}^{n} \mathbf{F_i} =0, \\\ \sum_{i=1}^{n} \mathbf{r_i}\times\mathbf{F_i} =0. $$
In this case, with the suitable initial conditions, the rigid body should remain motionless. This means that the internal forces between the point masses perfectly cancel the external forces. This is the mathematical statement that the following system of equations has a solution. The variables are the vectors $\mathbf{F_{ij}}$ for $i\neq j$, and the equations are:
- $ \mathbf{F_{ji}} + \mathbf{F_{ij}}=0$ (third law).
- $ (\mathbf{r_i}-\mathbf{r_j})\times\mathbf{F_{ij}}=0$ (forces are central, required for angluar momentum conservation).
- $ \sum_{j:j\neq i} \mathbf{F_{ij}} =- \mathbf{F_i} $ (forces cancel out).
I cannot see mathematically why this system of equations must have a solution.