Interpreting Probability Density Functions in a Physical Context

Question

A probability density function (PDF) is a function whose integral value corresponds to a probability range. Considering the PDF f(x), where x is a continuous variable over the domain $0$ to $\infty$, I know that:

\begin{equation} f(x)dx \end{equation}

corresponds to the probability that of finding something between the interval $x$ to $x+dx$. I also know that the mathematical probability of any specific event happening is exactly zero.

However, I fail to grasp the physical interpretation of PDFs. If $f(x)dx$ is the probability, what is $f(x)$?

Let's take a simple example. The Boltzmann Distribution, $p(\epsilon)$, can be used to model the probability of finding gas particles in thermal equilibrium at certain energy levels. We have that:

\begin{equation} P(E_1 \leq \epsilon \leq E_2)=\int_{E_1}^{E_2}p(\epsilon)d\epsilon \end{equation}

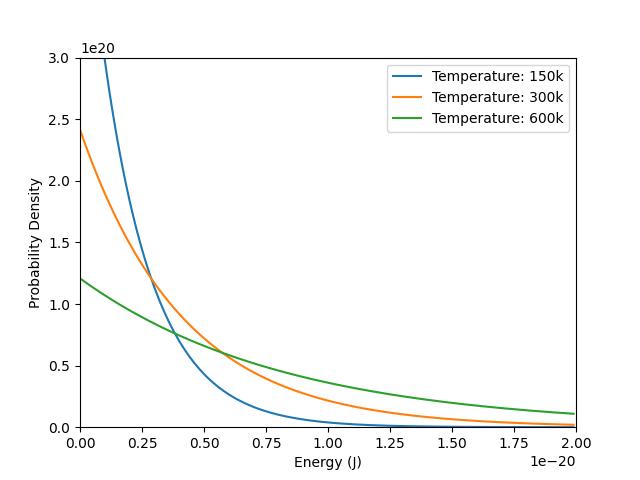
The probability that a gas particle have zero energy is exactly zero. Yet, the Boltzmann distribution has highest value at absciss $\epsilon=0$ at every temperature, as we can see in the following graph.

Thus, here are my questions:

1. Is there a physical interpretation to the value $p(\epsilon)$ or is this value purely conceptual?
2. What does it mean to have a higher value of PDF at $\epsilon=0$ in the Boltzmann distribution?
3. If the value in the Boltzmann distribution are simply conceptual, do they still have a unit?

Thanks a lot.

Answer 1

One way to sidestep all these pesky issues is to write it not as $f(x)$ or $p(\epsilon)$. Instead, they are $\frac{\mathrm d P}{\mathrm d x}$ and $\frac{\mathrm d P}{\mathrm d \epsilon}$, where $P$ is the cumulative probability distribution function.

This is particularly enlightening in the context of Planck's distribution, where you have different versions depending upon whether you are looking at the distribution in wavelength or in frequency. In reality, the crazy transformation law for them is because $$ f(\lambda) = f(\nu) \left | \frac{\mathrm d \nu}{\mathrm d \lambda} \right | \qquad \text{is really} \qquad \frac{\mathrm d P}{\mathrm d \lambda} = \frac{\mathrm d P}{\mathrm d \nu} \frac{\mathrm d \nu}{\mathrm d \lambda}$$ Because CDF are unitless in order to express a definite probability between 0 and 1, this means that these derivatives of CDF, which is nice to plot as PDF, these PDF must have as units the reciprocal of whatever is being differentiated.

It is perfectly ok for PDF to go to infinity, as long as the CDF is still sensible. i.e. as long as the integral of the PDF over its entire domain gives 1, there is no problems whatsoever. We like to plot PDF because the visual representation of the area underneath the PDF is directly related to how we think of where the likelihoods are.