How to identify the composition of sun if it is treated like a blackbody? [duplicate]

Question

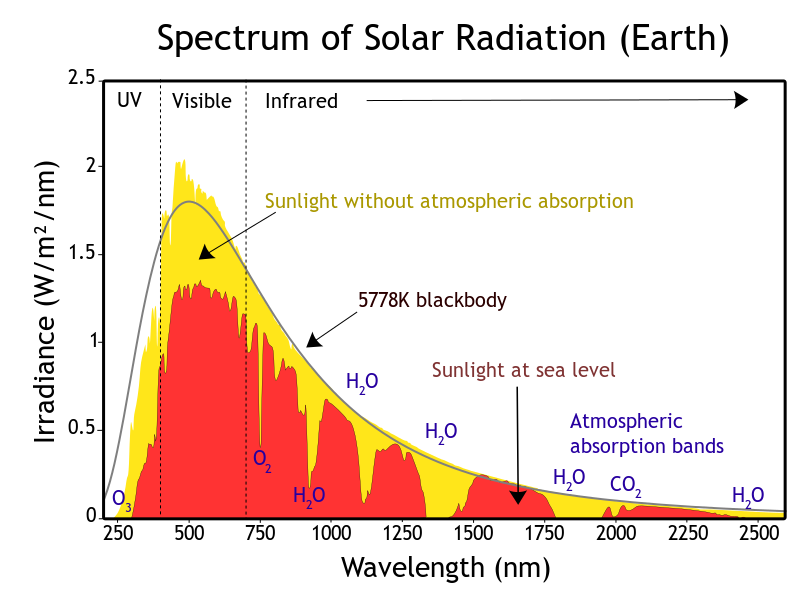
The sun is usually assumed to be approximately very close to a blackbody, but using spectroscopy it is concluded that the sun is made up of Hydrogen and Helium because the lines corresponding to those elements can be seen in the spectrum. If the sun is a blackbody shouldn't the peak of the spectrum be determined by Planck's law? If so how can we conclude that the sun is made of Helium and Hydrogen?

If those are the only lines visible how can we conclude that the sun is approximately a blackbody?

Edit: My question is not about why it can behave as blackbody, I was trying to understand this: How can it simultaneously behave as blackbody radiation, where the peak is dependent on temperature and at the same time spectroscopically tell us about it's composition where the peaks are dependent on the emmission spectra of its constituents

My question is not about why it can behave as blackbody, I was trying to understand this: How can it simultaneously behave as blackbody radiation, where the peak is dependent on temperature and at the same time spectroscopically tell us about it's composition where the peaks are dependent on the emmission spectra of its constituents.

Answer 1

So essentially the answer here is just what is written in the comments but let me go through this in a bit more detail. So the idea is essential that if we do not look too closely the sun behaves a lot like a black body, though it is not one! The spectrum of the sun is given below (Figure from https://en.wikipedia.org/wiki/Sunlight (20.03.2023))

The thin gray line represents the spectrum of a perfect black body, as it is theoretically described. Now if we compare this to the yellow spectrum (which is the suns spectrum as measured from space) this fits really well (especially at long wavelengths) however there are some deviations from the spectrum. Now what people mean when they say that the sun is a black body is that the spectrum approximately looks like that of a black body. And for example if you only care about the total amount of energy produced by the sun or, which frequency has the maximum intensity, treating the sun as a perfect black body works pretty well and is often enough. However, we find that the spectra do differ and do so in an interesting way. Especially interesting are the sharp dips in the spectrum at e.g. slightly below the UV line shown in the figure. These should never be there in the spectrum of a black body and as such require a different explanation. These lines are essentially exactly what permits us to determine the contents of the sun. (These lines are known as Fraunhofer lines btw. if you want to learn more about them)

One way to model this is to think of the sun as a black body surrounded by a cloud of gas. (The black body referees to the inner regions of the sun, while the cloud of gas referees to the outermost layers). Now the black body radiates with a "perfect" spectrum. However, as this light passes through this cloud of gas some of the light is absorbed. Not the absorption probability does heavily depend on the frequency of light, where essentially atoms (and molecules) only absorb light corresponding closely to the energy of some transition from an internal level to a different one. Now, these levels differ for different elements such that, by determining where these lines are we can determine the the composition of the gas cloud.