0
$\begingroup$

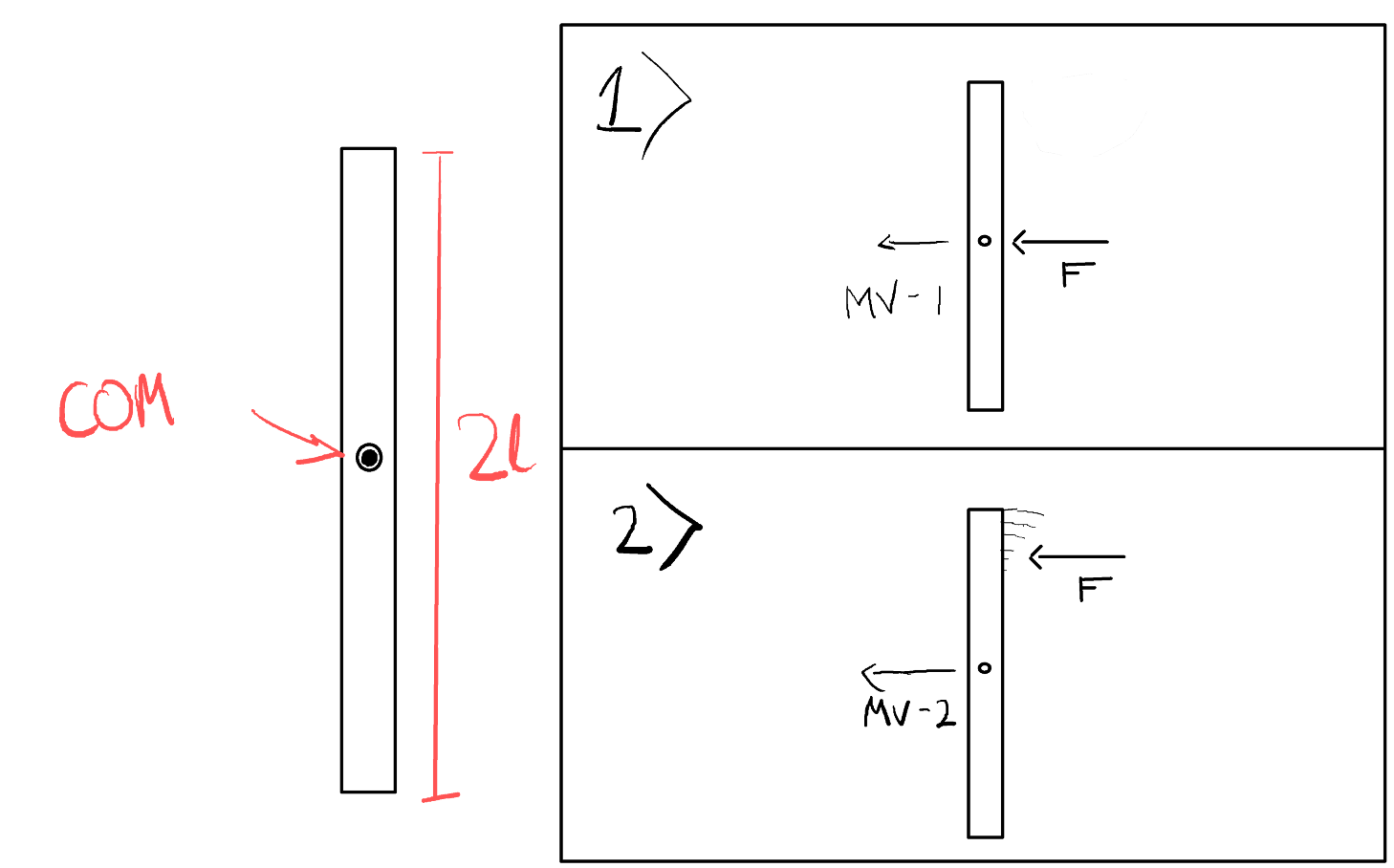
If there is a rod on a smooth surface, and a force F acts on it in 2 cases:

enter image description here

1>
F acts on the centre of mass, and it is the only force acting on the rod.
Since the change in momentum of the system would be equal to the Force acting on it,
MV-1(of the whole system) = F

2> F is the only force acting on the system, but it doesnt act on the centre of mass.
Here also, the change in momentum of the system would be equal to the Force acting on it, so
MV-2 = F
And MV-1 = MV-2
Even though the linear momentum (and mass, and therefore translational KE) of the system in the two cases are the same, as in the second case, the Force acts on one side of the rod, won't it also have angular momentum due to the torque that acted on it

The assumptions of this question :
Net Force on the system as a whole = (Vector) sum of all forces acting on it regardless of position
Change in linear momentum of the system as a whole = Net Force on the system as a whole

Now, when there is equal force being exerted in both cases, does the second case have more total energy than the first ? And if it does, why does the energy imparted depend on the position of where you apply the force?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Your question is more likely to attract attention if the images of you notes are converted into text. $\endgroup$
    – GrapefruitIsAwesome
    Commented Mar 19, 2023 at 15:16
  • $\begingroup$ ill keep note of that, thanks $\endgroup$
    – Tushar Hegde
    Commented Mar 19, 2023 at 16:27

1 Answer 1

Reset to default
0
$\begingroup$

When the three particles are not connected to one another you have a stationary particle hit by moving particle with the same mass.
If the collision is head on and elastic then the incoming particle is at rest after the collision with all the momentum and kinetic energy that it had taken away by the particle which it hit.

In the example with the three particles connected rigidly together it is impossible to satisfy simultaneously the condition that the incoming particle loses all it momentum and kinetic energy whilst also requiring the collision to be elastic.
Thus, if want the collision to be elastic then the incoming particle will have some momentum and kinetic energy after the collision.
Thus, when the particles are joined together your statement that $V_{\rm com} = V/3$ is not correct.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Ah okay. Thank you $\endgroup$
    – Tushar Hegde
    Commented Mar 19, 2023 at 15:55
  • $\begingroup$ If instead of taking the collision of the other particle, you consider just force acting on the rigid rod, does that impart a different amount of energy? Because the sum of all forces acting on the system would be the net force on the centre of mass in both cases, so the change in linear momentum would be same. But then again the second one is rotating $\endgroup$
    – Tushar Hegde
    Commented Mar 19, 2023 at 16:02

Not the answer you're looking for? Browse other questions tagged or ask your own question.