0
$\begingroup$

Typically inductance is calculated in the framework of DC currents, i.e. magnetostatic conditions. Then, the value of the inductance is adjusted for the case where the current is no longer DC. One such case is for wires of finite conductivity, and frequency of operation such that there is a change in current distribution of the conductor's cross section. The effect associated with skin depth can be explained via fields and waves incident on a conducting surface, or with so called "eddy currents". The latter concept stems from Lenz's Law

$$ \epsilon_i = - \dfrac{d \Phi_B}{dt} $$

The voltage induced by changes of flux is such as to create a current that counters this change of flux.

Now, the question is this: the treatment of the problem of the inductance of a wire over an infinite ground plane, does not seem to take effects eddy currents into account at all, at least in all the references I was able to find.

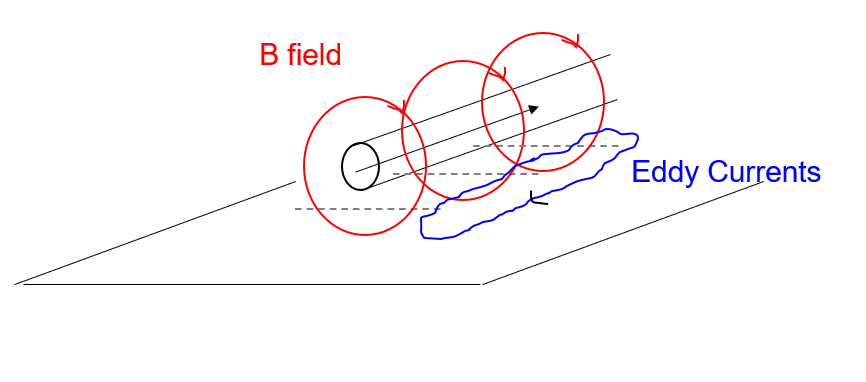
I am wondering whether this should not be the case even when all conductors are ideal. Here's what I'm thinking, with reference to this drawing:

wire over gnd plane

The varying B field generated by an alternating current also generates a varying flux across the GND plane. Therefore, there should be currents induced in the plane, to counter the flux variation. These currents add/subtract to the total field generated within the structure, therefore its flux, therefore the inductance.

Before embarking in an attempt a calculation, I would first like to understand whether what I'm thinking is correct or not.

Thanks.

EDIT :

After some more thinking, I came to realize that the condition for the magnetic field at a perfect conductor's interface is to be parallel to it. Therefore, there cannot be any flux variation in a surface coplanar with the ground, the way my previous pic illustrates. The red lines going into the conductor cannot be a result of this configuration and the pic is wrong. There is still a magnetic field parallel to the conductor, however given that the ground plane is perfectly conducting, any varying component should be confined to the surface of the plane, giving a flux of a varying B across any surface actually vanishing to zero. So this is the reason why no one bothers with this case.

Am I thinking correctly?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ The calculation of mutual inductance is much easier when ignoring the skin effect. In first approximation, we ignore it and then inductance is independent of frequency. Taking skin effect into account, inductance should depend on frequency somewhat. $\endgroup$
    – Ján Lalinský
    Commented Mar 11, 2023 at 23:49
  • $\begingroup$ The effect of distributed resistive losses on distributed planar inductors is actually a very important problem in the design of on-chip Si or SiGe integrated circuit inductors and it has a vast literature; just look in the IEEE database, see for example ieeexplore.ieee.org/search/…. $\endgroup$
    – hyportnex
    Commented Mar 12, 2023 at 1:55
  • $\begingroup$ Thanks for your comments. Actually, I meant to study the case where all conductors are perfect. The case where one is lossy is pretty clear. In other words: do eddy currents appear in a perfectly conducting ground? Do they change the shape of the field? How? I'll edit the post to make the question clearer. Thanks. $\endgroup$
    – Mike Anblips
    Commented Mar 12, 2023 at 15:14

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.