2
$\begingroup$

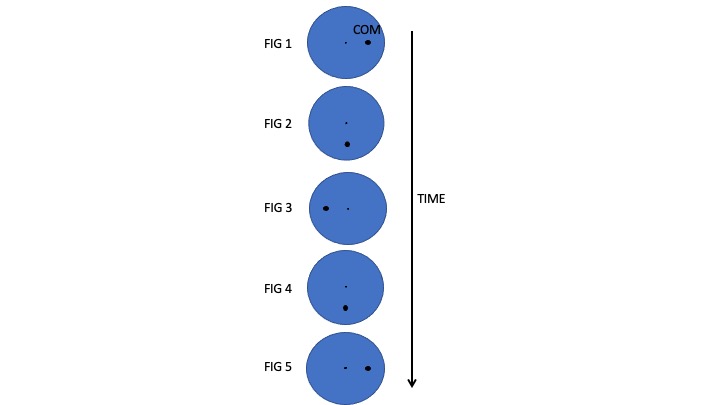
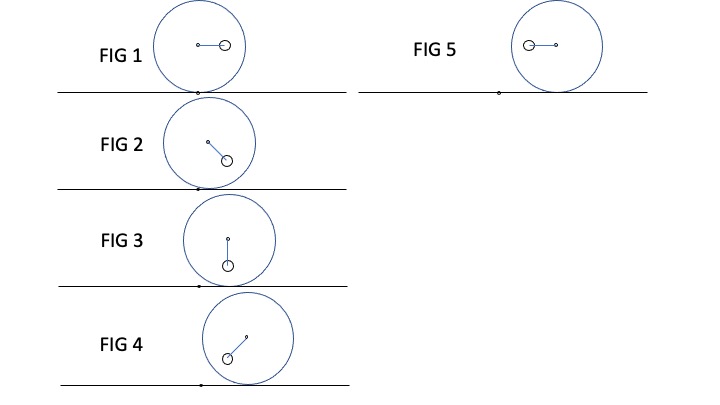
Assume a non uniform sphere, on a smooth horizontal floor. Its center of mass isn't at its geometrical center, but on the line passing through geometrical center and parallel to horizontal floor.

My textbook claims that it will move with a horizontal acceleration and rotate with an angular acceleration. I have no doubt about the angular acceleration as there is a net torque in some direction.

But what about the horizontal acceleration? Both the gravitational and the normal force are opposite to each other in vertical direction. How will the horizontal acceleration produced?

Improve this question
$\endgroup$
7
  • $\begingroup$ Simper scenario, imagine a rigid stick at an angle with a mass on the end, what force comes to mind then, the word rigid being key $\endgroup$
    – jensen paull
    Commented Mar 8, 2023 at 19:30
  • $\begingroup$ Since there would be no horizontal acceleration on a frictionless floor, as clearly explained by other answers, I'm wondering whether the problem implies that the floor is not frictionless, but just not bumpy so the sphere will roll nicely. $\endgroup$
    – Bob Wildfong
    Commented Mar 8, 2023 at 22:26
  • 1
    $\begingroup$ What textbook is this in? Can you give a reference? $\endgroup$
    – pwf
    Commented Mar 9, 2023 at 17:12
  • $\begingroup$ @pwf it is not a known one but my friend recommended. Name of the author is John Hooke. $\endgroup$
    – Chesx
    Commented Mar 9, 2023 at 19:00
  • 1
    $\begingroup$ Even simpler example: when you try to walk, no one pushes you, it is you who pushes against the ground and due to friction(and reaction force), you move forward. The same logic extends here. $\endgroup$
    – khaxan
    Commented Mar 10, 2023 at 15:31

3 Answers 3

Reset to default
7
$\begingroup$

If the floor is smooth (i.e. absolutely no friction) and horizontal then there is no horizontal force acting on the sphere and hence no horizontal acceleration. If the sphere is initially stationary then it will just rotate back and forth, with its centre of mass moving up and down along a vertical line.

If there is friction between the sphere and the floor then this friction provides a horizontal force which accelerates the sphere horizontally.

Improve this answer
$\endgroup$
9
  • $\begingroup$ This isn't quite right. You are correct that there is no horizontal acceleration for the CM, and so the CM will only move up and down, but in order for the CM to maintain its horizontal position the ball will shift left and right. The point of contact will not remain constant - if the CM starts to the right of the point of contact, later, when the CM is at its lowest point, the point of contact will be below it, and then as the CM rises the point of contact will be to the right of the CM. If the CM is interior and not on the surface, the ball will both roll and slip back and forth. $\endgroup$
    – pwf
    Commented Mar 9, 2023 at 16:55
  • $\begingroup$ @pwf Thank you. Fixed. $\endgroup$
    – gandalf61
    Commented Mar 9, 2023 at 17:49
  • $\begingroup$ @pwf do you mean sphere will oscillate about initial position horizontally? $\endgroup$
    – Chesx
    Commented Mar 9, 2023 at 19:02
  • $\begingroup$ @Chesx Yes, the geometric center will. $\endgroup$
    – pwf
    Commented Mar 9, 2023 at 19:04
  • $\begingroup$ @pwf So is there any criteria we can found horizontal acceleration (or velocity maybe). Just after releasing? $\endgroup$
    – Chesx
    Commented Mar 9, 2023 at 19:05
2
$\begingroup$

The first observation is that the normal force does not have to have the same magnitude as the gravitational force. They are not an action/reaction pair. Normal force on the floor must be the same size as normal force on the sphere. Gravitational force on the ball's center of mass must be the same size as the corresponding force on the Earth's center of mass. Net external force equals mass times the acceleration of the object's center of mass.

If smooth means zero friction, then there is no external horizontal force, so the center of mass cannot accelerate horizontally. As it begins at rest, it must move vertically. The normal force, whatever it may be, provides angular acceleration around the center of mass. The normal force will end up being whatever is necessary to coordinate the angular velocity around the C of M with the velocity of the C of M so as to keep that C of M acceleration vertical. The normal force will change as needed. The sphere must remain in contact with the floor to maintain the normal force.

Although the sphere does rotate and appear to move, it does not actually "roll". The ball will slide along the floor as necessary to hold to Newton's 2nd Law for rigid extend objects: net external force equals total mass times acceleration of the center of mass, net external torque around the center of mass equals rotational inertial around the cnter of mass times angular acceleration.

Improve this answer
$\endgroup$
2
$\begingroup$

How will the horizontal acceleration produced?

It won't if the surface is completely frictionless and horizontal, because only friction can produce a horizontal force acting on the sphere in response to the applied torque. Without friction the sphere will essentially spin in place, i.e., spin with no horizontal motion relative to the floor.

I suggest the sphere will be behave as follows:

If the sphere is initially released from rest as shown in FIG 1 below with the center of mass (COM) located to the right of, and in line with, the geometric center, it will initially rotate clockwise, due to the clockwise torque about the geometric center created by gravity.

It will reach maximum angular velocity when the COM is directly above the frictionless surface as shown in FIG 2. It will continue rotating until the COM again comes to rest at its original height as shown in FIG 3. The rotation will then reverse as shown in FIGs 4 and 5 and the whole process repeat itself.

In this manner I suggest the COM of the sphere will rotate from side to side in place, similar to the motion of the bob of a pendulum.

Hope this helps.

enter image description here

enter image description here

Improve this answer
$\endgroup$
17
  • $\begingroup$ This is almost right. The ball will not rock around its geometric center, but about its CM. In your diagram, the CM points should all align vertically. Meanwhile, the CM will bob up and down as constrained by the shape of the ball and the floor, and the sphere will appear to rock from side to side as it bobs up and down. (Yes, frictionless surfaces are weird!) $\endgroup$
    – pwf
    Commented Mar 8, 2023 at 23:28
  • $\begingroup$ How about the initial acceleration (just after release). Will it still rotate at its own place? $\endgroup$
    – Chesx
    Commented Mar 9, 2023 at 5:34
  • $\begingroup$ @pwf what do you mean by rock side to side? $\endgroup$
    – Bob D
    Commented Mar 9, 2023 at 10:15
  • $\begingroup$ @Chesx what do you mean by “its own place”? $\endgroup$
    – Bob D
    Commented Mar 9, 2023 at 11:59
  • $\begingroup$ By "rock side to side," I mean that the geometric center will shift left and right. The CM will not, but from the outside it will look like it is (since when we see a round balll we tend instinctively to assume its CM is at its geometric center). Line your images up so the CM dots are all vertically aligned and I think you'll see what I mean. Fig's 1 and 5 will be shifted to the left relative to 2 and 4, and Fig 3 will be shifted to the right. $\endgroup$
    – pwf
    Commented Mar 9, 2023 at 16:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.