1
$\begingroup$

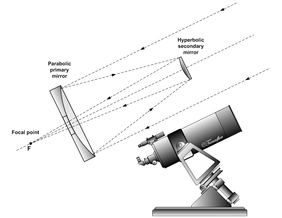
Typically, telescopes are explained in terms of bouncing light paths around. For example, this image from wikipedia shows "photon tracks" being redirected:

enter image description here

I realize this is a very effective way to model telescopes, but I was wondering if this is actually reflective of what happens or if it's just a really great approximation.

Wouldn't the wave function of a photon arriving from far away be spread out over quite a huge area? So the photon's wavefunction would be arriving as a sort of spread-out wall? But in that case the mirror isn't working by redirecting the path, it's working by creating interference effects depending on how the wall arrives. Or maybe that's totally wrong! I dunno! That's the question!

What is the quantum mechanical description of a photon arriving at a telescope, from extremely far away?

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ Your are jumping a step here: before talking about photons it is worthwhile to understand how telescope works from the point of view of classical em waves theory (and how ray optics approximate it.) Transition to photons is then is rather trivial. $\endgroup$
    – Roger V.
    Commented Feb 15, 2023 at 7:47
  • 1
    $\begingroup$ Those lines are ligth rays, not photons., en.wikipedia.org/wiki/Ray_(optics) $\endgroup$
    – anna v
    Commented Feb 15, 2023 at 12:27
  • 1
    $\begingroup$ @RogerVadim Pointing out where to read about how ray optics emerges from classical EM would be a nice partial answer. $\endgroup$
    – Craig Gidney
    Commented Feb 15, 2023 at 20:35
  • $\begingroup$ I have puzzled about this for years. A photon escaping from an excited atom in a distant galaxy surely escapes in many simultaneous directions, like the radiation from an antenna. The wave packet of the photon surely describes this. It's spread must be enormous laterally, although longitudally it does not disperse. If it is an infra-red photon it is able to cope with a long distance array telescope however large. Is there a reason why the wave-packet theory of an electron (apart from it's dispersion) does not apply to the photon? $\endgroup$
    – John Roche
    Commented Sep 25, 2023 at 14:36
  • $\begingroup$ Are you familiar and completely comfortable with the Mott problem? That's really what you are asking about: spherical waves as straight particle trajectories. $\endgroup$
    – Cosmas Zachos
    Commented Sep 25, 2023 at 19:53

2 Answers 2

Reset to default
1
$\begingroup$

A telescope receiving enough photons to take a picture of a distant galaxy is also receiving enough photons (in terms of sheer numbers!) that the classical description of optics (regarding lenses, mirrors, prisms, etc.) furnishes a perfectly adequate overall picture of the process- no quantum mechanics is needed.

If we look at a single photon departing that galaxy, it is still a single photon by the time it reaches our telescope and its wave function is not "spread out" as such- it's just that there are fewer photons in the beam by the time it enters the telescope.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ This answer is kind of dodging the question instead of answering it. I'm looking for something more quantitatively detailed, like a specific wave function with x,y,z,t arguments. It's fine if you have to assume we're talking about a really dim image, so that there's only one photon in the apparatus at a time in expectation. I'm specifically interested in knowing the purely quantum description so I can get a better sense of how the classical limit emerges from it. $\endgroup$
    – Craig Gidney
    Commented Feb 15, 2023 at 20:31
  • $\begingroup$ (I do appreciate the answer, it's just not quite what I'm looking for!) $\endgroup$
    – Craig Gidney
    Commented Feb 15, 2023 at 20:32
  • $\begingroup$ For example, when you say its wave function is not spread out, could you give a number? A nanometer? A meter? A kilometer? $\endgroup$
    – Craig Gidney
    Commented Feb 15, 2023 at 20:35
-1
$\begingroup$

From what I've read scientists have not used QM to describe the transmission of a photon in free space. Maxwell derived an equation based on electro and magnetic fields that say a simple sine wave is possible.

Feynman used a path integral theory which is related to QM to describe a probability function .... where a photon is likely to go in an experimental setup.

For a single photon the site has many questions about "how big is a photon" but there is no specific answer.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.