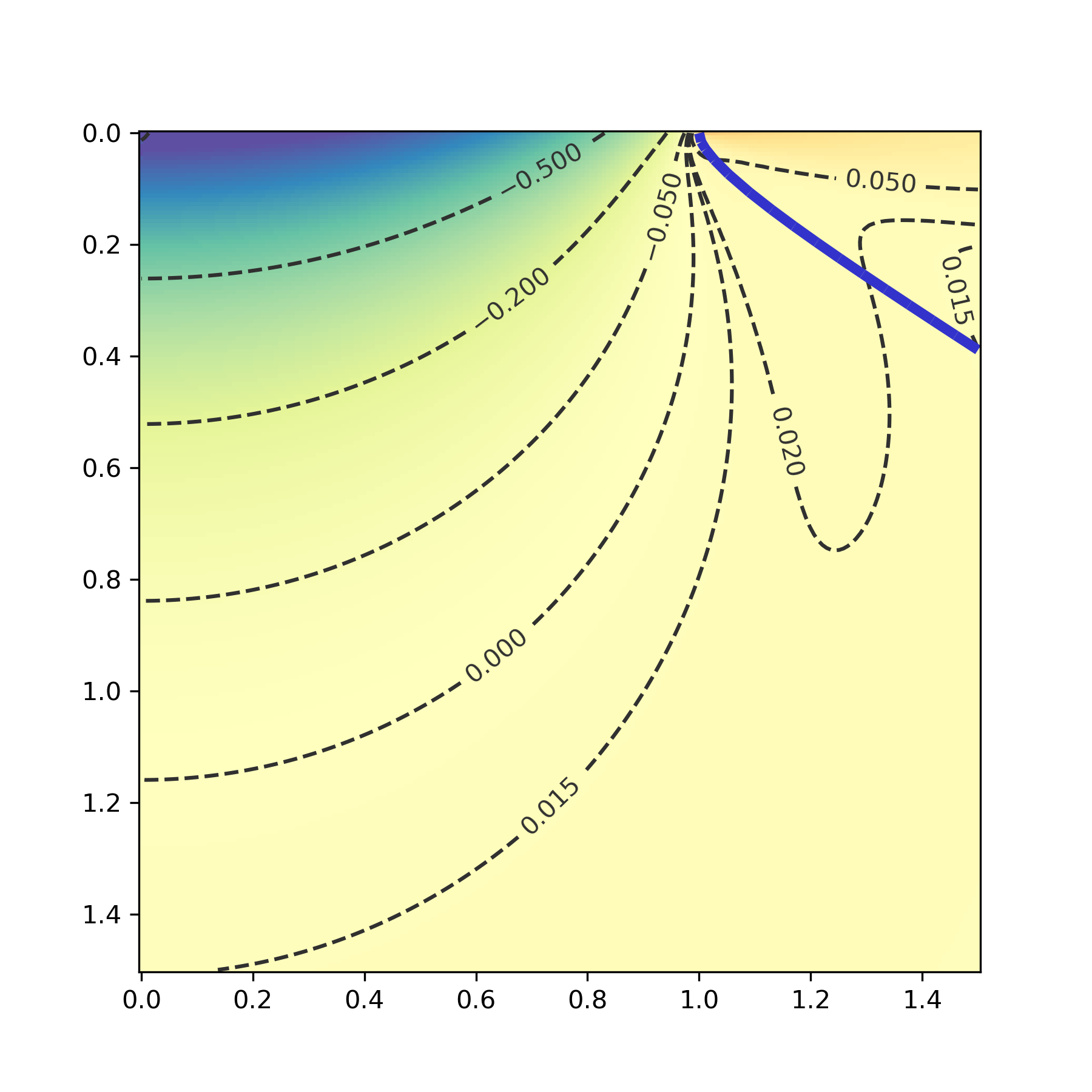
I'm trying to understand the Hertzian cone fracture process from a continuum mechanics point of view. I'm considering a problem where an elastic sphere is pressed quasi-statically onto an elastic semi-infinite space. Following chapter 2 in the reference Handbook of contact mechanics Ch. 2 by V. L. Popov, M. Hess, and E. Willert. The Cauchy stress tensor in the elastic half space takes the following form (cylindrical coordinates):
$$\sigma_{ij} = \begin{bmatrix} \sigma_{rr} & 0 & \tau_{rz}\\ 0 & \sigma_{\theta \theta} & 0\\ \tau_{rz} & 0 & \sigma_{zz} \end{bmatrix}$$ The $z$ axis points down into the half space, the $r$ axis points parallel to the initially half space surface, and $\theta$ is the azimuthal angle (rotations around the z-axis). The shear stresses $\tau_{r\theta}$ and $\tau_{z \theta}$ are zero by symmetry. The components of the stress tensor takes the following explicit form: \begin{equation} \begin{split} \frac{2\sigma_{rr}}{3p_0} &= \frac{1-2\nu}{3} \left(\frac{a}{r}\right)^2 \left[1- \left(\frac{z}{\sqrt{u}}\right)^3 \right] + \left(\frac{z}{\sqrt{u}}\right)^3 \frac{a^2 u}{u^2 + a^2 z^2}\\ &+\left(\frac{z}{\sqrt{u}}\right)\left[\frac{1-\nu}{a^2+u}u + \left(1+\nu\right) \frac{\sqrt{u}}{a}\arctan\left(\frac{a}{\sqrt{u}}\right) - 2 \right], \end{split} \end{equation}
\begin{equation} \begin{split} -\frac{2\sigma_{\theta \theta}}{3p_0} &= \frac{1-2\nu}{3} \left(\frac{a}{r}\right)^2 \left[1- \left(\frac{z}{\sqrt{u}}\right)^3 \right]\\ &+\left(\frac{z}{\sqrt{u}}\right)\left[2\nu +\frac{1-\nu}{a^2+u}u - \left(1+\nu\right) \frac{\sqrt{u}}{a}\arctan\left(\frac{a}{\sqrt{u}}\right) \right], \end{split} \end{equation}
\begin{equation} \begin{split} -\frac{2\sigma_{zz}}{3p_0} &= \left(\frac{z}{\sqrt{u}}\right)^3 \frac{a^2 u}{u^2 + a^2 z^2}, \end{split} \end{equation} and \begin{equation} \begin{split} -\frac{2\tau_{rz}}{3p_0} &= \frac{rz^2}{u^2 + a^2 z^2}\cdot \frac{a^2 \sqrt{u}}{u+a^2}. \end{split} \end{equation} I hope I got all of those factors right. $\nu$ denotes the Poisson ratio of the elastic half space. $a$ is the contact radius between the sphere and elastic half space. $p_0$ is a mean stress. For our purposes $\nu$, $a$, and $p_0$ can be considered constants. $a$ and $p_0$ set the scale for lengths and stresses.
The parameter $u$ is defined as \begin{equation} u = \frac{1}{2} \left(r^2 + z^2 - a^2 +\sqrt{\left(r^2 + z^2 - a^2\right)^2 + 4a^2 z^2} \right) \end{equation}
The eigenvalues of the Cauchy tensor (the principal stresses) are \begin{equation} \begin{split} &\sigma_1 = \frac{1}{2} \left( \sigma_{rr} + \sigma_{zz} + \sqrt{\left(\sigma_{rr}-\sigma_{zz}\right)^2 + 4 \tau_{rz}^2} \right),\\ &\sigma_2 = \sigma_{\theta \theta},\\ &\sigma_3 = \frac{1}{2} \left( \sigma_{rr} + \sigma_{zz} - \sqrt{\left(\sigma_{rr}-\sigma_{zz}\right)^2 + 4 \tau_{rz}^2} \right). \end{split} \end{equation}
Now in the references, B. R. Lawn, A. Franco, and P. D. Warren, et. al. these equations are used to argue for that cone fracture can be understood. For instance in B. R. Lawn Fig. 2 looks like this:
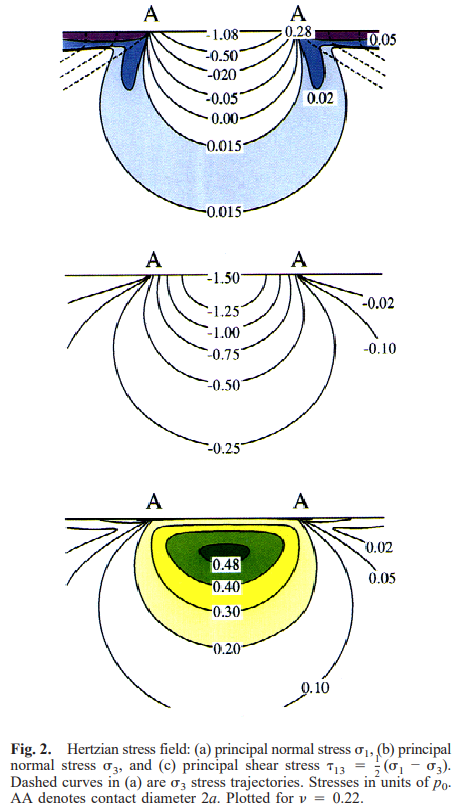
I have then read that this diagram is supposed to explain cone fractures because outside of the contact area AA $\sigma_1$ (most positive principal stress) changes from being compressive to tensile.
In H. Chen et. al. there is a relationship between the direction of the cone crack. In the z-r plane this relationship is $$\frac{dz}{dr} = \frac{\tau_{rz}}{\sigma_1-\sigma_{rr}}.$$
My issue is that I do not understand how the figure explains cone cracks, and how the above relationship comes about. The above relationship looks alot like the angle necessary to rotate the coordinate system to obtain principal stresses. Finally, I have a vague idea of that the cone cracks are supposed to follow the trajectories of one of the principle stresses but I do not understand why.
If anyone can elaborate on these issues I would be very thankful.