The following is a question from a tutorial in my Physics 2 course about conductors and the Method of image charges.
We are given two infinite perpendicular and grounded plains.
The first plain is in the $X-Z$ plain and the second one is on the $Z-Y$ plain.
A point charge $q$ is set at the point $(a,b)$, where $a,b>0$.
Find image charges to the problem
Find the electric field
This question has a solution in the tutorial, the answer to the first question is given as the following image which also explains the setting of the problem:
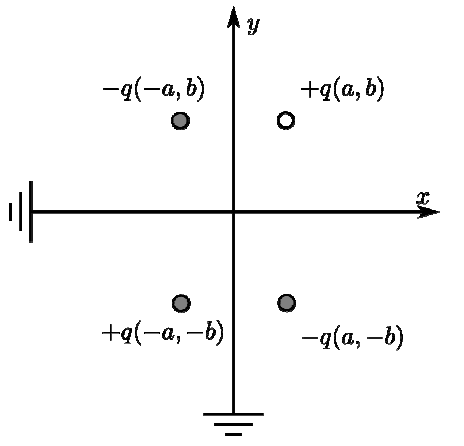
The answer to the second question is divided into two parts, for the region $x,y>0$ and elsewhere.
The solution claims that in the region $x,y>0$ the electric field is that of the four charges seen in the image, and I agree (this follows from the uniqueness theorem).
However, I don't understand the last part of the solution, the solution says that the electric field in those regions $x<0$ or $y<0$ is $0$.
Why is the electric field $0$ there ?
I know that since we put charges there we can not use the method of image charges since we actually changed the charge density in the place we are trying to calculate the electric field at, so I don't have a method for tackling this.
Since the answer doesn't justify this claim, and since the answer is $0$, I figured there must be a simple explanation, but I am not able to think of one.